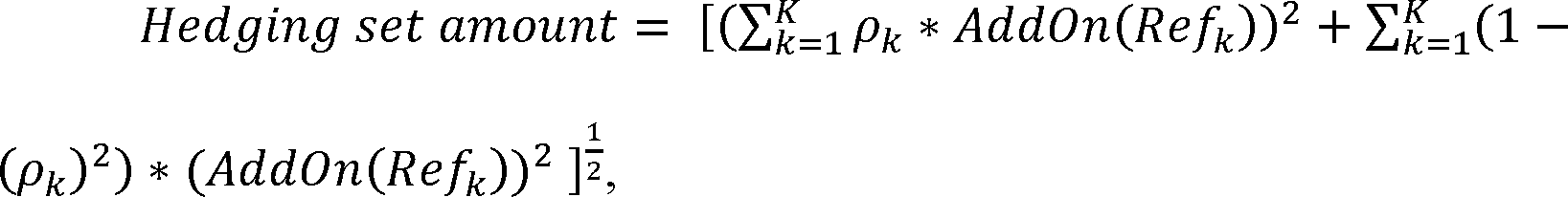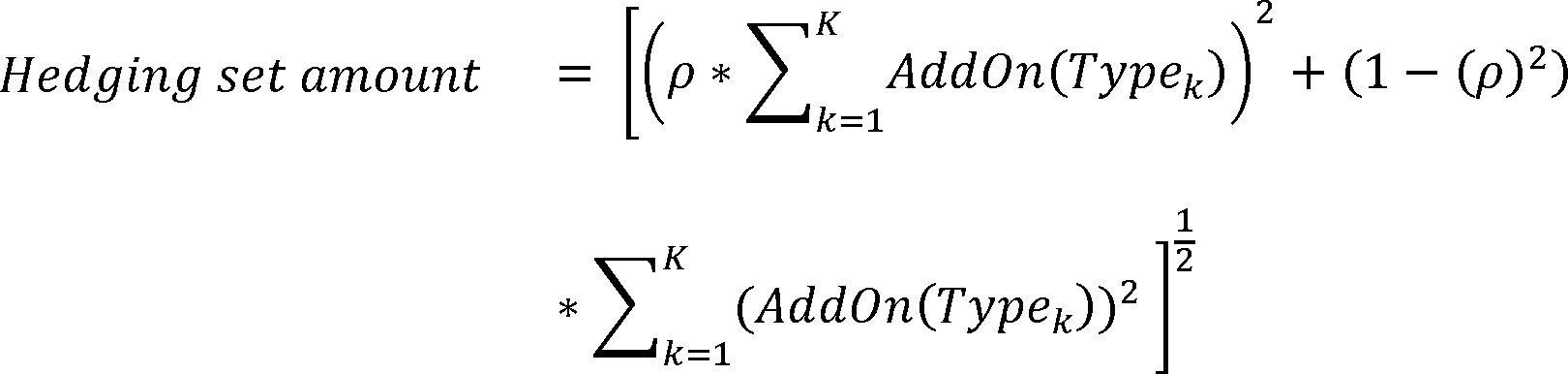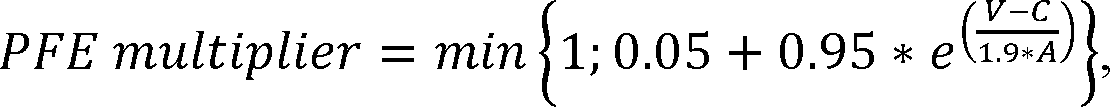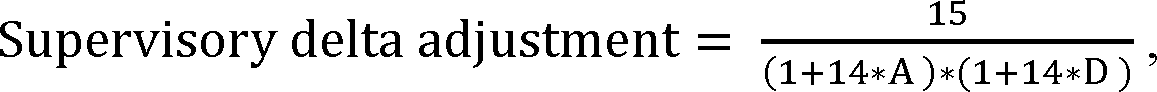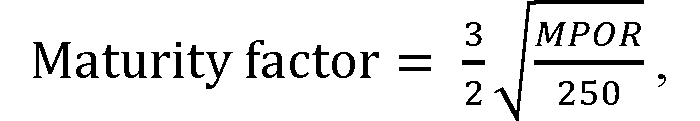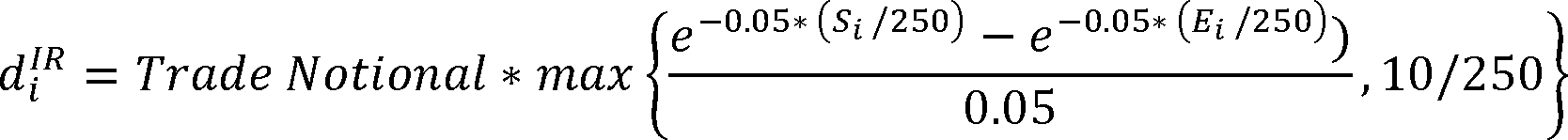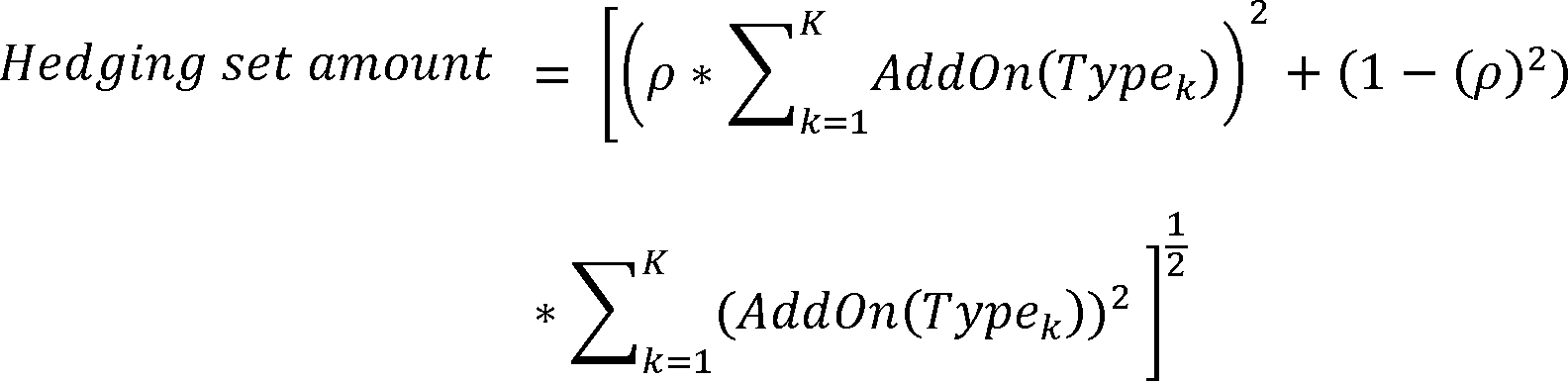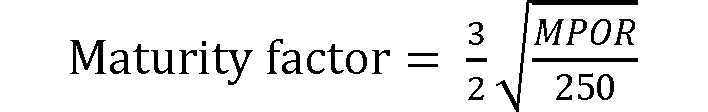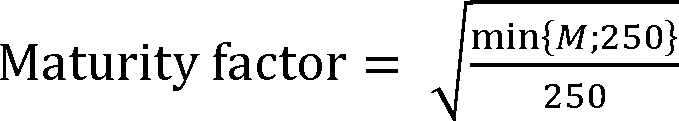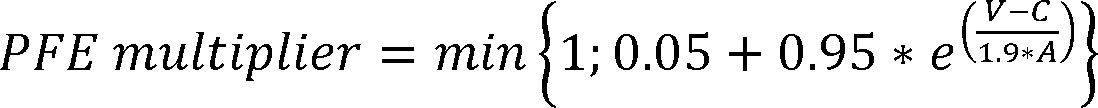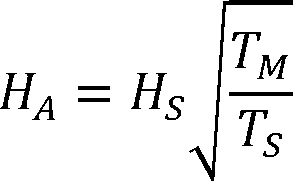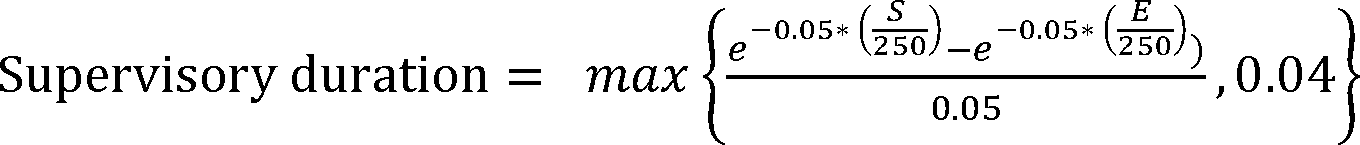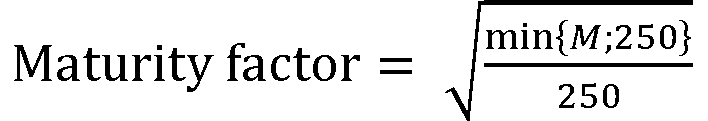2018-24924. Standardized Approach for Calculating the Exposure Amount of Derivative Contracts

AGENCY:

The Board of Governors of the Federal Reserve System; the Federal Deposit Insurance Corporation; and the Office of the Comptroller of the Currency, Treasury.

ACTION:

Notice of proposed rulemaking.

SUMMARY:

The Board of Governors of the Federal Reserve System, the Federal Deposit Insurance Corporation, and the Office of the Comptroller of the Currency (together, the agencies) are inviting public comment on a proposal that would implement a new approach for calculating the exposure amount of derivative contracts under the agencies' regulatory capital rule. The proposed approach, called the standardized approach for counterparty credit risk (SA-CCR), would replace the current exposure methodology (CEM) as an additional methodology for calculating advanced approaches total risk-weighted assets under the capital rule. An advanced approaches banking organization also would be required to use SA-CCR to calculate its standardized total risk-weighted assets; a non-advanced approaches banking organization could elect to use either CEM or SA-CCR for calculating its standardized total risk-weighted assets. In addition, the proposal would modify other aspects of the capital rule to account for the proposed implementation of SA-CCR. Specifically, the proposal would require an advanced approaches banking organization to use SA-CCR with some adjustments to determine the exposure amount of derivative contracts for calculating total leverage exposure (the denominator of the supplementary leverage ratio). The proposal also would incorporate SA-CCR into the cleared transactions framework and would make other amendments, generally with respect to cleared transactions. The proposed introduction of SA-CCR would indirectly affect the Board's single counterparty credit limit rule, along with other rules. The Office of the Comptroller of the Currency also is proposing to update cross-references to CEM and add SA-CCR as an option for determining exposure amounts for derivative contracts in its lending limit rules.

DATES:

Comments should be received on or before February 15, 2019.

ADDRESSES:

Comments should be directed to:

Board: You may submit comments, identified by Docket No. [R-1629 and RIN 7100-AF22], by any of the following methods:

1. Agency Website: http://www.federalreserve.gov. Follow the instructions for submitting comments at http://www.federalreserve.gov/​generalinfo/​foia/​ProposedRegs.cfm.

2. Email: [e-mail]. Include docket number in the subject line of the message.

3. Fax: [phone] or [phone].

4. Mail: Ann E. Misback, Secretary, Board of Governors of the Federal Reserve System, 20th Street and Constitution Avenue NW, Washington, DC 20551. All public comments are available from the Board's website at http://www.federalreserve.gov/​generalinfo/​foia/​ProposedRegs.cfm as submitted, unless modified for technical reasons or to remove sensitive personal identifying information (PII) at the commenter's request. Public comments may also be viewed electronically or in paper form in Room 3515, 1801 K Street NW (between 18th and 19th Streets NW), Washington, DC 20006 between 9:00 a.m. and 5:00 p.m. on weekdays.

FDIC: You may submit comments, identified by RIN 3064-AE80, by any of the following methods:

• Agency Website: http://www.fdic.gov/​regulations/​laws/​federal. Follow instructions for submitting comments on the Agency website.
• Email: [e-mail]. Include “RIN 3064-AE80” on the subject line of the message.
• Mail: Robert E. Feldman, Executive Secretary, Attention: Comments/RIN 3064-AE80, Federal Deposit Insurance Corporation, 550 17th Street NW, Washington, DC 20429.
• Hand Delivery/Courier: Comments may be hand delivered to the guard station at the rear of the 550 17th Street Building (located on F Street) on business days between 7 a.m. and 5 p.m. All comments received must include the agency name (FDIC) and RIN 3064-AE80 and will be posted without change to http://www.fdic.gov/​regulations/​laws/​federal,, including any personal information provided.

OCC: You may submit comments to the OCC by any of the methods set forth below. Commenters are encouraged to submit comments through the Federal eRulemaking Portal or email, if possible. Please use the title “Capital Adequacy: Standardized Approach for Calculating the Exposure Amount of Derivative Contracts” to facilitate the organization and distribution of the comments. You may submit comments by any of the following methods:

• Federal eRulemaking Portal—“Regulations.gov”: Go to www.regulations.gov. Enter “Docket ID OCC-2018-0030” in the Search Box and click “Search.” Click on “Comment Now” to submit public comments.
• Click on the “Help” tab on the Regulations.gov home page to get information on using Regulations.gov, including instructions for submitting public comments.
• Email: [e-mail].
• Mail: Legislative and Regulatory Activities Division, Office of the Comptroller of the Currency, 400 7th Street SW, suite 3E-218, Washington, DC 20219.
• Hand Delivery/Courier: 400 7th Street SW, Suite 3E-218, Washington, DC 20219.

Instructions: You must include “OCC” as the agency name and “Docket ID OCC-2018-0030” in your comment. In general, the OCC will enter all comments received into the docket and publish the comments on the Regulations.gov website without change, including any business or personal information that you provide such as name and address information, email addresses, or phone numbers. Comments received, including attachments and other supporting materials, are part of the public record and subject to public disclosure. Do not include any information in your comment or supporting materials that you consider confidential or inappropriate for public disclosure.

You may review comments and other related materials that pertain to this rulemaking action by any of the following methods:

• Viewing Comments Electronically: Go to www.regulations.gov. Enter “Docket ID OCC-2018-0030” in the Search box and click “Search.” Click on “Open Docket Folder” on the right side of the screen. Comments and supporting materials can be viewed and filtered by clicking on “View all documents and comments in this docket” and then using the filtering tools on the left side of the screen.
• Click on the “Help” tab on the Regulations.gov home page to get information on using Regulations.gov. The docket may be viewed after the close of the comment period in the same manner as during the comment period.
• Viewing Comments Personally: You may personally inspect comments at the OCC, 400 7th Street SW, Washington, DC 20219. For security reasons, the OCC requires that visitors make an appointment to inspect comments. You may do so by calling [phone] or, for persons who are deaf or hearing impaired, TTY, [phone]. Upon arrival, visitors will be required to present valid government-issued photo identification and submit to security screening in order to inspect comments.

FOR FURTHER INFORMATION CONTACT:

 

Board: Constance M. Horsley, Deputy Associate Director, [phone]; David Lynch, Deputy Associate Director, [phone]; Elizabeth MacDonald, Manager, [phone]; Michael Pykhtin, Manager, [phone]; Mark Handzlik, Senior Supervisory Financial Analyst, [phone]; Sara Saab, Supervisory Financial Analyst, [phone]; or Noah Cuttler, Senior Financial Analyst, [phone]; Division of Supervision and Regulation; or Benjamin W. McDonough, Assistant General Counsel, [phone]; Mark Buresh, Counsel, [phone]; Andrew Hartlage, Counsel, [phone]; Legal Division, Board of Governors of the Federal Reserve System, 20th and C Streets NW, Washington, DC 20551. For the hearing impaired only, Telecommunication Device for the Deaf, [phone].

FDIC: Bobby R. Bean, Associate Director, [e-mail]; Irina Leonova, Senior Policy Analyst, [e-mail]; Peter Yen, Senior Policy Analyst, [e-mail], Capital Markets Branch, Division of Risk Management Supervision, [phone]; or Michael Phillips, Counsel, [e-mail]; Catherine Wood, Counsel, [e-mail]; Supervision Branch, Legal Division, Federal Deposit Insurance Corporation, 550 17th Street NW, Washington, DC 20429.

OCC: Guowei Zhang, Risk Expert, Capital Policy, [phone]; Kevin Korzeniewski, Counsel, [phone]; or Ron Shimabukuro, Senior Counsel, [phone], or, for persons who are deaf or hearing impaired, TTY, [phone], Chief Counsel's Office, Office of the Comptroller of the Currency, 400 7th Street SW, Washington, DC 20219.

SUPPLEMENTARY INFORMATION:

Table of Contents

I. Background

A. Scope and Application of the Proposed Rule

B. Proposal's Interaction With Agency Requirements and Other Proposals

C. Overview of Derivative Contracts

D. Mechanics of the Current Exposure Methodology

E. Mechanics of the Internal Models Methodology

F. Review of the Capital Rule's Treatment of Derivative Contracts

II. Standardized Approach for Counterparty Credit Risk

A. Key Concepts

1. Netting Sets

2. Hedging Sets

3. Derivative Contract Amount for the PFE Component Calculation

4. Collateral Recognition and Differentiation Between Margined and Unmargined Derivative Contracts

B. Mechanics of the Standardized Approach for Counterparty Credit Risk

1. Exposure Amount

2. Replacement Cost

3. Aggregated Amount and Hedging Set Amounts

4. PFE Multiplier

5. PFE Calculation for Nonstandard Margin Agreements

6. Adjusted Derivative Contract Amount

7. Example of Calculation

III. Revisions to the Cleared Transactions Framework

A. Trade Exposure Amount

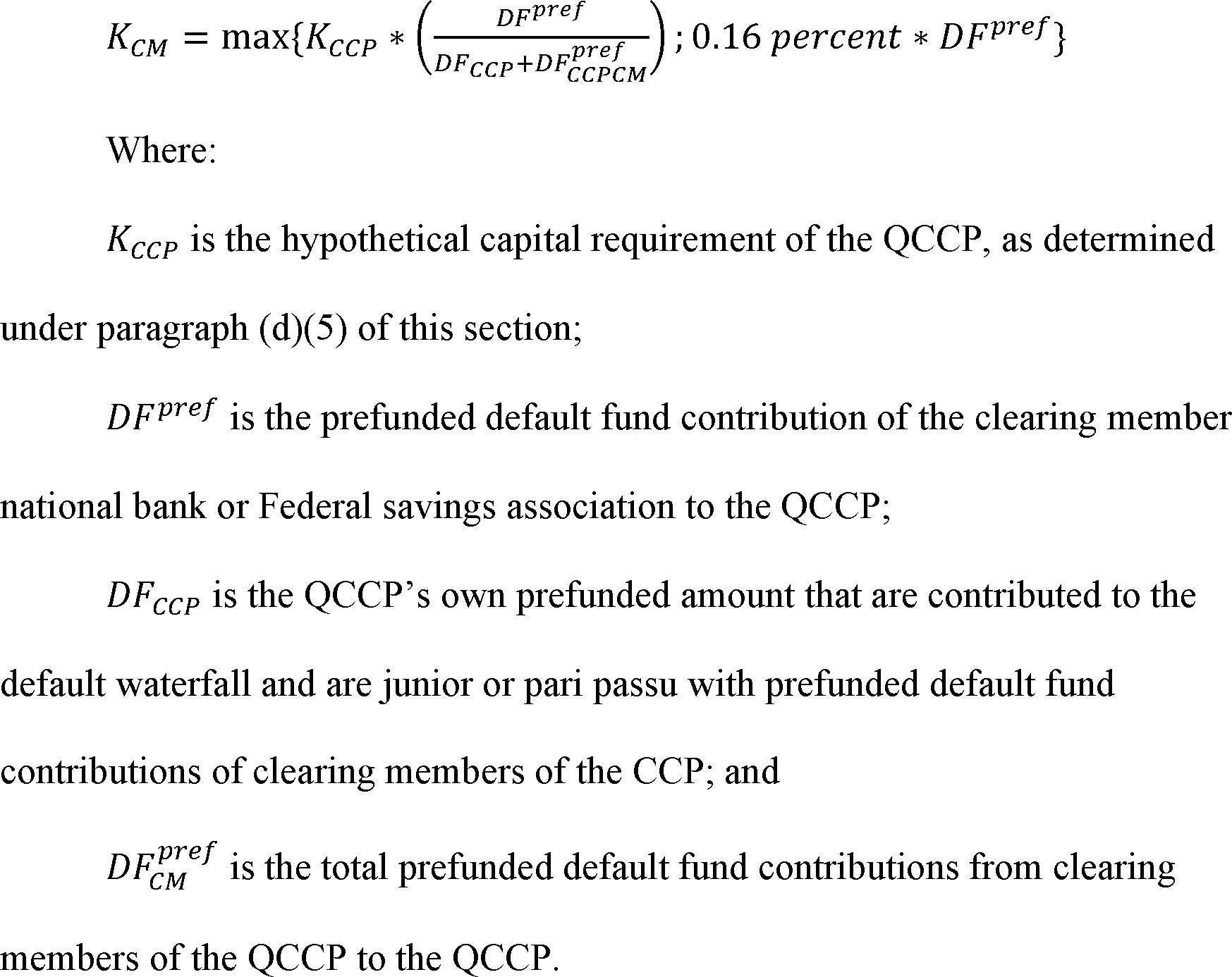
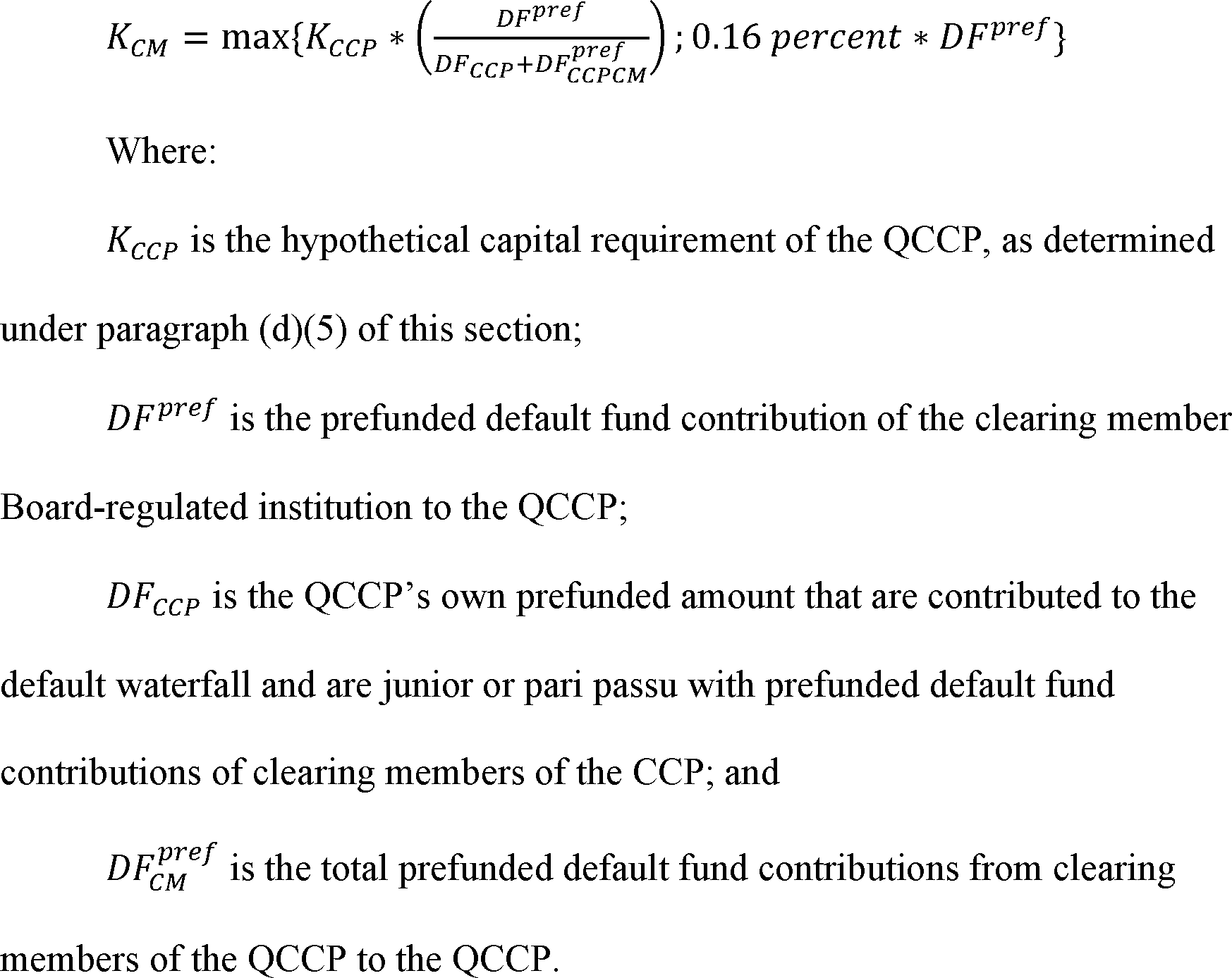
B. Treatment of Collateral

C. Treatment of Default Fund Contributions

IV. Revisions to the Supplementary Leverage Ratio

V. Technical Amendments

A. Receivables Due From a QCCP

B. Treatment of Client Financial Collateral Held by a CCP

C. Clearing Member Exposure When CCP Performance is Not Guaranteed

D. Bankruptcy Remoteness of Collateral

E. Adjusted Collateral Haircuts for Derivative Contracts

F. OCC Revisions to Lending Limits

VI. Impact of the Proposed Rule

VII. Regulatory Analyses

A. Paperwork Reduction Act

B. Regulatory Flexibility Act

C. Plain Language

D. Riegle Community Development and Regulatory Improvement Act of 1994

E. OCC Unfunded Mandates Reform Act of 1995 Determination

I. Background

A firm with a positive exposure on a derivative contract expects to receive a payment from its counterparty and is subject to the credit risk that the counterparty will default on its obligations and fail to pay the amount owed under the derivative contract. Because of this, the regulatory capital rule (capital rule) ^[1] of the Board of Governors of the Federal Reserve System (Board), the Federal Deposit Insurance Corporation (FDIC), and the Office of the Comptroller of the Currency (OCC) (together, the agencies) requires a banking organization ^[2] to hold regulatory capital based on the exposure amount of its derivative contracts. The agencies are issuing this notice of proposed rulemaking (proposal) to implement a new approach for calculating the exposure amount of derivative contracts under the capital rule.

As discussed in greater detail below, the capital rule prescribes different approaches to measuring the exposure amount of derivative contracts, depending on the size and complexity of the banking organization. For example, all banking organizations are required to use the current exposure methodology (CEM) to determine the exposure amount of their derivative contracts under the standardized approach of the capital rule, which is based on formulas described in the capital rule. Advanced approaches banking organizations also may use an internal models-based approach, the internal models methodology (IMM), to determine the exposure amount of their derivative contracts under the advanced approaches of the capital rule.^[3] The addition of a new approach, called the standardized approach for counterparty credit risk (SA-CCR), would provide important improvements to risk-sensitivity and calibration relative to CEM, but also would provide a less complex and non-model-dependent approach than IMM.

In addition, the agencies are proposing to revise the capital rule's cleared transactions framework and the supplementary leverage ratio to accommodate the proposed implementation of SA-CCR, as well as make certain other changes to the cleared transaction framework in the capital rule.

A. Scope and Application of the Proposed Rule

The capital rule provides two methodologies for determining total risk-weighted assets: The standardized approach, which applies to all banking organizations, and the advanced approaches, which apply only to advanced approaches banking organizations. The standardized approach serves as a floor on advanced approaches banking organizations' total risk-weighted assets, and thus such banking organizations must calculate total risk-weighted assets under both approaches.^[4] Total risk-weighted assets are the denominator of the risk-based capital ratios; regulatory capital is the numerator.

Under the standardized approach, the risk-weighted asset amount for a derivative contract is the product of the exposure amount of the derivative contract and the risk weight applicable to the counterparty, as provided under the capital rule. Under the advanced approaches, the risk-weighted asset amount for a derivative contract is derived using the internal ratings-based approach, which multiplies the exposure amount (or exposure at default amount) of the derivative contract by a models-based formula that uses risk parameters determined by a banking organization's internal methodologies.^[5]

Both the standardized approach and the advanced approaches require a banking organization to determine the exposure amount for its derivative contracts that are not cleared transactions (i.e., over-the-counter derivative contracts or noncleared derivative contracts). As part of the cleared transactions framework, both the standardized approach and the advanced approaches require a banking organization to determine the exposure amount of its derivative contracts that are cleared transactions (i.e., cleared derivative contracts) and determine the risk-weighted asset amounts of its contributions or commitments to mutualized loss sharing agreements with central counterparties (i.e., default fund contributions). For the advanced approaches, an advanced approaches banking organization may use either CEM or IMM to calculate the exposure amount of its noncleared and cleared derivative contracts, as well as the risk-weighted asset amounts of its default fund contributions. For purposes of determining these amounts for the standardized approach, all banking organizations must use CEM.

The proposal would revise the standardized approach and the advanced approaches for advanced approaches banking organizations by replacing CEM with SA-CCR. As a result, for purposes of determining total risk-weighted assets under the advanced approaches, an advanced approaches banking organization would have the option to use SA-CCR or IMM to calculate the exposure amount of its noncleared and cleared derivative contracts, as well as to determine the risk-weighted asset amount of its default fund contributions. For purposes of determining the exposure amount of these items under the standardized approach, an advanced approaches banking organization would be required to use SA-CCR.

The capital rule also requires an advanced approaches banking organization to meet a supplementary leverage ratio. The denominator of the supplementary leverage ratio, called total leverage exposure, includes the exposure amount of a banking organization's derivative contracts. The capital rule requires an advanced approaches banking organization to use CEM to determine the exposure amount of its derivative contracts for total leverage exposure. Under the proposal, an advanced approaches banking organization would be required to use SA-CCR to determine the exposure amount of its derivative contracts for total leverage exposure.

As it applies to advanced approaches banking organizations, the proposed implementation of SA-CCR would provide important improvements to risk-sensitivity and calibration relative to CEM, resulting in more appropriate capital requirements for derivative contracts. SA-CCR also would be responsive to concerns raised regarding the current regulatory capital treatment for derivative contracts under CEM. For example, the industry has raised concerns that CEM does not appropriately recognize collateral, including the risk-reducing nature of variation margin, and does not provide sufficient netting for derivative contracts that share similar risk factors. The agencies intend for the proposed implementation of SA-CCR to respond to these concerns, and to be substantially consistent with international standards issued by the Basel Committee on Banking Supervision (Basel Committee). In addition, requiring an advanced approaches banking organization to use SA-CCR or IMM for all purposes under the advanced approaches would facilitate regulatory reporting and the supervisory assessment of an advanced approaches banking organization's capital management program.

The proposed implementation of SA-CCR would require advanced approaches banking organizations to augment existing systems or develop new ones. Accordingly, the proposal includes a transition period, until July 1, 2020, by which time an advanced approaches banking organization must implement SA-CCR. An advanced approaches banking organization may, however, adopt SA-CCR as of the effective date of the final rule. In addition, the technical revisions in this proposal, as described in section V of this Supplementary Information, would become effective as of the effective date of the final rule.

While the agencies recognize that implementation of SA-CCR offers several improvements to CEM, it also will require, particularly for banking organizations with relatively small derivatives portfolios, internal systems enhancements and other operational modifications that could be costly and present additional burden. Therefore, the proposal would not require non-advanced approaches banking organizations to use SA-CCR, but instead would provide SA-CCR as an optional approach. However, a non-advanced approaches banking organization that elects to use SA-CCR for calculating its exposure amount for noncleared derivative contracts also would be required to use SA-CCR to calculate the exposure amount for its cleared derivative contracts and for calculating the risk-weighted asset amount of its default fund contributions. This approach should provide meaningful flexibility, while promoting consistency for the regulatory capital treatment of derivative contracts for non-advanced approaches banking organizations. The proposal also would allow non-advanced approaches banking organizations to adopt SA-CCR as of the effective date of the final rule.

Table 1—Scope and Applicability of the Proposed Rule

	Non-cleared derivative contracts	Cleared transactions framework	Default fund contribution
Advanced approaches banking organizations, advanced approaches total risk-weighted assets	Option to use SA-CCR or IMM to determine exposure amount for derivative contracts under the advanced approaches	Must use the approach selected for purposes of the counterparty credit risk framework (either SA-CCR or IMM), to determine the trade exposure amount for cleared derivative contracts	Must use SA-CCR for purposes of the default fund contribution included in risk-weighted assets.
Advanced approaches banking organizations, standardized approach total risk-weighted assets	Must use SA-CCR to determine exposure amount for derivative contracts	Must use SA-CCR to determine trade exposure amount for cleared derivative contracts	Must use SA-CCR for purposes of the default fund contribution included in risk-weighted assets.
Non-advanced approaches banking organizations, standardized approach total risk-weighted assets	Option to use CEM or SA-CCR to determine exposure amount for derivative contracts	Must use the approach selected for purposes of the counterparty credit risk framework (either CEM or SA-CCR), to determine the trade exposure amount for cleared derivative contracts	Must use the approach selected for purposes of the counterparty credit risk framework (either CEM or SA-CCR) for purposes of the default fund contribution included in risk-weighted assets.
Advanced approaches banking organizations, supplementary leverage ratio	Must use modified SA-CCR to determine the exposure amount of derivative contracts for total leverage exposure under the supplementary leverage ratio.

Question 1: The agencies invite comment on all aspects of this proposal. In addition to the risk-sensitivity enhancements SA-CCR provides relative to CEM, what other considerations relevant to the determination of whether to replace CEM with SA-CCR for advanced approaches banking organizations should the agencies consider?

Question 2: The agencies invite comment on the proposed effective date of SA-CCR for advanced approaches banking organizations. What alternative timing should be considered and why?

B. Proposal's Interaction With Agency Requirements and Other Proposals

The Board's single counterparty credit limit rule (SCCL) authorizes a banking organization subject to the SCCL to use any methodology that such a banking organization may use under the capital rule to value a derivative contract for purposes of the SCCL.^[6] Thus, for valuing a derivative contract under the SCCL, the proposal would require an advanced approaches banking organization that is subject to the SCCL to use SA-CCR or IMM and would require a non-advanced approaches banking organization that is subject to the SCCL to use CEM or SA-CCR.^[7] In addition, the agencies net stable funding ratio proposed rules would cross-reference provisions of the agencies' supplementary leverage ratio that are proposed to be amended in this proposal, and thus this proposal potentially could affect elements of the net stable funding ratio rulemaking.^[8]

The agencies also are in the process of considering the appropriate scope of “advanced approaches banking organizations” and may propose changes to the scope of this term in the near future. The agencies anticipate that the proposal on the scope of “advanced approaches banking organizations” would have an overlapping comment period with this proposal. Commenters should consider both proposals together for purposes of their comments to the agencies.

C. Overview of Derivative Contracts

In general, derivative contracts represent agreements between parties either to make or receive payments or to buy or sell an underlying asset on a certain date (or dates) in the future. Parties generally use derivative contracts to mitigate risk, although nonhedging use of derivative contracts also occurs. For example, an interest rate derivative contract allows a party to manage the risk associated with a change in interest rates, while a commodity derivative contract allows a party to lock in commodity prices in the future and thereby minimize any exposure attributable to any uncertainty with respect to subsequent movements in those prices.

The value of a derivative contract, and thus a party's exposure to its counterparty, changes over the life of the contract based on movements in the value of the reference rates, assets, or indices underlying the contract. A party with a positive current exposure expects to receive a payment or other beneficial transfer from the counterparty and is considered to be “in the money.” A party that is in the money is subject to counterparty credit risk: The risk that the counterparty will default on its obligations and fail to pay the amount owed under the transaction. In contrast, a party with a zero or negative current exposure does not expect to receive a payment or beneficial transfer from the counterparty and is considered to be “at the money” or “out of the money.” A party that has no current exposure to counterparty credit risk may have exposure to counterparty credit risk in the future if the derivative contract becomes “in the money.”

To mitigate the counterparty credit risk of a derivative contract, parties typically exchange collateral. In the derivatives context, collateral is either variation margin or initial margin (also known as independent collateral). Parties exchange variation margin on a periodic basis during the term of a derivative contract, as typically specified in a variation margin agreement or by regulation.^[9] Variation margin offsets changes in the market value of a derivative contract and thereby covers the potential loss arising from default of a counterparty. Variation margin may not always be sufficient to cover a party's positive exposure (e.g., due to delays in receiving collateral), and thus parties may exchange initial margin. Parties typically exchange initial margin at the outset of the derivative contract and usually in an amount that does not directly depend on changes in the value of the derivative contract. Parties typically post initial margin in amounts that would reduce the likelihood of a positive exposure amount for the derivative contract in the event of the counterparty's default, resulting in overcollateralization.

To facilitate the exchange of collateral, variation margin agreements typically provide for a threshold amount and a minimum transfer amount. The threshold amount is the amount by which the market value of the derivative contract can change before a party must collect or post variation margin (in other words, the threshold amount specifies an acceptable amount of under-collateralization). The minimum transfer amount is the smallest amount of collateral that a party must transfer when it is required to exchange collateral under the variation margin agreement. Parties generally apply a discount (also known as a haircut) to collateral to account for a potential reduction in the value of the collateral during the period between the last exchange of collateral before the close out of the derivative contract (as in the case of default of the counterparty) and the replacement of the contract on the market. This period is known as the margin period of risk (MPOR). Often, two parties will enter into a large number of derivative contracts together. In such cases, the parties may enter into a netting agreement to allow for offsetting of the derivative contracts and to streamline certain aspects of the contracts, including the exchange of collateral.

Parties to a derivative contract may clear their derivative contracts through a central counterparty (CCP). The use of central clearing is designed to improve the safety and soundness of the derivatives markets through the multilateral netting of exposures, establishment and enforcement of collateral requirements, and the promotion of market transparency. A party engages with a CCP either as a clearing member or as a clearing member client. A clearing member is a member of, or direct participant in, a CCP that is entitled to enter into transactions with the CCP. A clearing member client is a party to a cleared transaction associated with a CCP in which a clearing member acts as a financial intermediary with respect to the clearing member client and either takes one position with the client and an offsetting position with the CCP (the principal model) or guarantees the performance of the clearing member client to the CCP (the agency model). With respect to the latter, the clearing member generally is responsible for fulfilling CCP initial and variation margin calls irrespective of the client's ability to post collateral.

D. Mechanics of the Current Exposure Methodology

Under CEM, the exposure amount of a single derivative contract is equal to the sum of its current credit exposure and potential future exposure (PFE).^[10] Current credit exposure reflects a banking organization's current exposure to its counterparty and is equal to the greater of zero and the on-balance sheet fair value of the derivative contract.^[11] PFE approximates the banking organization's potential exposure to its counterparty over the remaining maturity of the derivative contract. PFE equals the product of the notional amount of the derivative contract and a supervisory-provided conversion factor, which reflects the potential volatility in the reference asset for the derivative contract.^[12] The capital rule gives the supervisory-provided conversion factors via a simple look-up table, based on the derivative contract's type and remaining maturity.^[13] In general, potential exposure increases as volatility and duration of the derivative contract increases.

If certain criteria are met, CEM allows a banking organization to measure the exposure amount of a portfolio of its derivative contracts with a counterparty on a net basis, rather than on a gross basis, resulting in a lower measure of exposure and thus a lower capital requirement. A banking organization may measure, on a net basis, derivative contracts that are subject to the same qualifying master netting agreement (QMNA). A QMNA, in general, means a netting agreement that permits a banking organization to terminate, close-out on a net basis, and promptly liquidate or set off collateral upon an event of default of the counterparty.^[14] To qualify as a QMNA, the netting agreement must satisfy certain operational requirements under § _.3 of the capital rule.^[15]

For derivative contracts subject to a QMNA, the exposure amount equals the sum of the net current credit exposure and the adjusted sum of the PFE amounts of the derivative contracts.^[16] The net current credit exposure is the greater of the net sum of all positive and negative fair values of the individual derivative contracts subject to the QMNA or zero.^[17] Thus, derivative contracts that have positive and negative fair values can offset each other to reduce the net current credit exposure, subject to a floor of zero. The adjusted sum of the PFE amount component provides the netting function, and is a function of the gross PFE amount of the derivative contracts and the net-to-gross ratio. The gross PFE amount is the sum of the PFE of each derivative contract subject to the QMNA. The net-to-gross ratio is the ratio of the net current credit exposure of each derivative contract subject to the QMNA to the sum of the positive current credit exposure of these derivative contracts. Specifically, the adjusted sum of the PFE amounts equals the sum of (1) the gross PFE amount multiplied by 0.4 and (2) the gross PFE amount multiplied by the net-to-gross ratio and 0.6.^[18] Thus, as the net-to-gross ratio decreases so will the adjusted sum of the PFE amounts.

For all derivative contracts calculated under CEM, a banking organization may recognize the credit-risk-mitigating benefits of financial collateral, pursuant to § _.37 of the capital rule. In particular, a banking organization may either apply the risk weight applicable to the collateral to the secured portion of the exposure or net exposure amounts and collateral amounts according to a regulatory formula that includes certain haircuts for collateral.^[19]

E. Mechanics of the Internal Models Methodology

Under IMM, an advanced approaches banking organization uses its own internal models of exposure to determine the exposure amount of its derivative contracts. The exposure amount under IMM is calculated as the product of the effective expected positive exposure (EEPE) for a netting set, which is the time-weighted average of the effective expected exposures (EE) profile over a one-year horizon, and an alpha factor.^[20] For the purposes of regulatory capital calculations, the resulting exposure amount is treated as a loan equivalent exposure, which is the amount effectively loaned by the banking organization to the counterparty under the derivative contract.

F. Review of the Capital Rule's Treatment of Derivative Contracts

CEM was developed several decades ago and, as a result, does not reflect recent market conventions and regulatory requirements that are designed to reduce the risks associated with derivative contracts.^[21] For banking organizations with substantial derivatives portfolios in particular, this can result in a significant mismatch between the risk posed by these portfolios and the regulatory capital that the banking organization must hold against them. For instance, CEM does not differentiate between margined and unmargined derivative contracts, and it does not function well with other regulatory requirements, including the swap margin rule, which mandates the exchange of initial margin and variation margin for specified covered swap entities.^[22] In addition, the net-to-gross ratio under CEM does not recognize, in an economically meaningful way, the risk-reducing benefits of a balanced derivative portfolio (i.e., mixed long and short positions). Further, the agencies developed the supervisory conversion factors provided under CEM prior to the 2007-2008 financial crisis and they have not been recalibrated to reflect stress volatilities observed in recent years.

Although IMM is more risk-sensitive than CEM, IMM is more complex and requires prior supervisory approval before an advanced approaches banking organization may use it. Specifically, an advanced approaches banking organization seeking to use IMM must demonstrate to its primary federal supervisor that it has established and maintains an infrastructure with risk measurement and management processes appropriate for the firm's size and level of complexity.^[23]

For these reasons, the Basel Committee developed SA-CCR and published it as a final standard in 2014.^[24] Relative to CEM, SA-CCR provides a more risk-sensitive approach to determining the replacement cost and PFE for a derivative contract. Notably, SA-CCR improves collateral recognition (e.g., by differentiating between margined and unmargined derivative contracts); allows a banking organization to recognize meaningful, risk-reducing relationships between derivative contracts within a balanced derivative portfolio; and better captures recently observed stress volatilities among the primary risk drivers for derivative contracts. In addition, relative to IMM, SA-CCR provides a standardized, nonmodelled approach that is more accessible to banking organizations to determine the exposure amount for derivative contracts.

II. Standardized Approach for Counterparty Credit Risk

A. Key Concepts

1. Netting Sets

Under SA-CCR, a banking organization would calculate the exposure amount of its derivative contracts at the netting set level. The Basel Committee standard provides that a netting set may not be subject to more than one margin agreement. Thus, a banking organization, under the Basel Committee standard, would need to calculate the exposure amount at the level of each margin agreement and not at the level of each QMNA, regardless whether multiple margin agreements are under the same QMNA. The agencies recognize, however, that the Basel Committee standard does not reflect current industry practice and regulatory requirements, in which QMNAs often cover multiple margin agreements to order to reduce credit risk by increasing the net settlement of derivative contracts. Accordingly, and as with CEM, the proposal would allow a banking organization to calculate the exposure amount of multiple derivative contracts under the same netting set so long as each derivative contract is subject to the same QMNA. For purposes of SA-CCR, a derivative contract that is not subject to a QMNA would comprise a netting set of one derivative contract. Thus, the proposal would define a netting set to mean either one derivative contract between a banking organization and a single counterparty, or a group of derivative contracts between a banking organization and a single counterparty that are subject to a QMNA. The proposal would retain the capital rule's current definition of a QMNA.

2. Hedging Sets

For the PFE calculation under SA-CCR, a banking organization would fully or partially net derivative contracts within the same netting set that share similar risk factors. This approach would recognize that derivative contracts with similar risk factors share economically meaningful relationships (i.e., are more tightly correlated) and thus netting would be appropriate. In contrast, CEM recognizes only 60 percent of the netting benefits of derivative contracts subject to a QMNA, without accounting for relationships between derivative contracts' underlying risk factors.

To effectuate this approach, the proposal would introduce the concept of hedging sets, which would generally mean those derivative contracts within the same netting set that share similar risk factors. The proposal would define five types of hedging sets—interest rate, exchange rate, credit, equity, and commodities—and would provide formulas for netting within each hedging set. Each formula would be particular to each hedging set type and would reflect regulatory correlation assumptions between risk factors in the hedging set.

3. Derivative Contract Amount for the PFE Component Calculation

As with CEM, a banking organization would use an adjusted derivative contract amount for the PFE component calculation under SA-CCR. Unlike CEM, the agencies intend for the adjusted derivative contract amount under SA-CCR to reflect, in general, a conservative estimate of EEPE for a netting set composed of a single derivative contract, assuming zero fair value and zero collateral. As part of the estimate, SA-CCR would use updated supervisory factors that reflect stress volatilities observed during the financial crisis. The supervisory factors would reflect the variability of the primary risk factor of the derivative contract over a one-year horizon. In addition, SA-CCR would apply a separate maturity factor to each derivative contract that would scale down, if necessary, the default one-year risk horizon of the supervisory factor to the risk horizon appropriate for the derivative contract. A banking organization would apply a positive sign to the derivative contract amount if the derivative contract is long the risk factor and a negative sign if the derivative contract is short the risk factor. This adjustment, along with the assumption of zero fair value and zero collateral, would allow a banking organization to recognize offsetting and diversification between derivative contracts that share similar risk factors (i.e., long and short derivative contracts within the same hedging set would be able to fully or partially offset one another).

4. Collateral Recognition and Differentiation Between Margined and Unmargined Derivative Contracts

The proposal would make several improvements to the recognition of collateral under SA-CCR. The proposal would account for collateral directly within the SA-CCR exposure amount calculation, whereas under CEM a banking organization recognizes the collateral only after the exposure amount has been determined. For replacement cost, the proposal would recognize collateral on a one-for-one basis. For PFE, SA-CCR would introduce the concept of a PFE multiplier, which would allow a banking organization to reduce the PFE amount through recognition of overcollateralization, in the form of both variation margin and independent collateral, and account for negative fair value amounts of the derivative contracts within the netting set. In addition, the proposal would differentiate between margined and unmargined derivative contracts such that a netting set that is subject to a variation margin agreement (as defined in the proposal) would always have a lower or equal exposure amount than an equivalent netting set that is not subject to a variation margin agreement.

B. Mechanics of the Standardized Approach for Counterparty Credit Risk

1. Exposure Amount

Under § _.132(c)(5) of the proposed rule, the exposure amount of a netting set would be equal to an alpha factor of 1.4 multiplied by the sum of the replacement cost of the netting set and PFE of the netting set. The can be represented as follows:

exposure amount = 1.4 * (replacement cost + PFE)

The alpha factor was included in the Basel Committee standard under the view that a standardized approach, such as SA-CCR, should not produce lower exposure amounts than a modelled approach. Therefore, to instill a level of conservatism consistent with the Basel Committee standard, the proposal would apply an alpha factor of 1.4 in order to produce exposure measure outcomes that generally are no lower than those amounts calculated using IMM. While the estimates of PFE under SA-CCR are conservative in many cases, the estimates of the sum of the replacement cost and PFE under SA-CCR would necessarily be close to IMM's EEPE for netting sets where the replacement cost dominates PFE.^[25] Thus, reducing the value of alpha in SA-CCR below 1.4 could result in exposure amounts produced by SA-CCR that are smaller than exposure amounts produced by IMM for such deep in-the-money netting sets.

The exposure amount would be zero, however, for a netting set that consists only of sold options in which the counterparties to the options have paid the premiums up front and the options are not subject to a variation margin agreement.

Question 3: The agencies invite comment on whether the objective of ensuring that SA-CCR produces more conservative exposure amounts than IMM is appropriate for the implementation of SA-CCR. Does the incorporation of the alpha factor support this objective, why or why not? Are there alternative measures the agencies could incorporate into SA-CCR to support this objective? Are there other objectives regarding the comparability of SA-CCR and IMM that the agencies should consider? The agencies encourage commenters to provide appropriate data or examples to support their response.

2. Replacement Cost

SA-CCR would provide separate formulas for replacement cost depending on whether the counterparty to a banking organization is required to post variation margin. In general, when a banking organization is a net receiver of financial collateral, the amount of financial collateral would be positive, which would reduce replacement cost. Conversely, when the banking organization is a net provider of financial collateral, the amount of financial collateral would be negative, which would increase replacement cost. In all cases, replacement cost cannot be lower than zero. In addition, for purposes of calculating the replacement cost component (and the PFE multiplier), the fair value amount of the derivative contract would exclude any valuation adjustments. The purpose of excluding valuation adjustments is to arrive at the risk-free value of the derivative contract, and this requirement would exclude credit valuation adjustments, among other adjustments, as applicable.

Section _.2 of the proposed rule provides a definition of variation margin and independent collateral, as well as the variation margin amount and the independent collateral amount. The proposal would define variation margin as financial collateral that is subject to a collateral agreement provided by one party to its counterparty to meet the performance of the first party's obligations under one or more transactions between the parties as a result of a change in value of such obligations since the last time such financial collateral was provided. Variation margin amount would mean the fair value amount of the variation margin that a counterparty to a netting set has posted to a banking organization less the fair value amount of the variation margin posted by the banking organization to the counterparty.

Further, consistent with the capital rule, the amount of variation margin included in the variation margin amount would be adjusted by the standard supervisory haircuts under § _.132(b)(2)(ii) of the capital rule. The standard supervisory haircuts ensure that the derivative contract remains appropriately collateralized from a regulatory capital perspective, notwithstanding any changes in the value of the financial collateral. In particular, the standard supervisory haircuts address the possible decrease in the value of the financial collateral received by a banking organization and an increase in the value of the financial collateral posted by the banking organization over a one-year time horizon.

The standard supervisory haircuts are based on a ten-business-day holding period for derivative contracts, and the capital rule requires a banking organization to adjust, as applicable, the standard supervisory haircuts to align with the risk horizon of the associated derivative contract. To be consistent with this proposal, the agencies are proposing to revise the standard supervisory haircuts so that they align with the maturity factor adjustments as provided under SA-CCR. In particular, an unmargined derivative contract and a margined derivative contract that is not a cleared transaction would receive a holding period of 10 business days. A derivative contract that is a cleared transaction would receive a holding period of five business days.^[26] A banking organization would be required to use a holding period of 20 business days for collateral associated with a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, and if a netting set contains one or more trades involving illiquid collateral or a derivative contract that cannot be easily replaced. Notwithstanding the aforementioned, a banking organization would be required to double the applicable holding period if the derivative contract is subject to an outstanding dispute over variation margin.

The proposal would define independent collateral as financial collateral, other than variation margin, that is subject to a collateral agreement, or in which a banking organization has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; and notwithstanding the prior security interest of any custodial agent or any prior security interest granted to a CCP in connection with collateral posted to that CCP), and the amount of which does not change directly in response to the value of the derivative contract or contracts that the financial collateral secures.

The proposal would define the net independent collateral amount as the fair value amount of the independent collateral that a counterparty to a netting set has posted to a banking organization less the fair value amount of the independent collateral posted by the banking organization to the counterparty, excluding such amounts held in a bankruptcy remote manner,^[27] or posted to a qualifying central counterparty (QCCP) and held in conformance with the operational requirements in § _.3 of the capital rule. As with variation margin, independent collateral also would be subject to the standard supervisory haircuts under § _.132(b)(2)(ii) of the capital rule.

Under § _.132(c)(6)(ii) of the proposed rule, the replacement cost of a netting set that is not subject to a variation margin agreement is the greater of (1) the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set, less the net independent collateral amount applicable to such derivative contracts, or (2) zero. This can be represented as follows:

replacement cost = max{V−C; 0}

Where:

V is the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set; and

C is the net independent collateral amount applicable to such derivative contracts.

The same requirement would apply to a netting set that is subject to a variation margin agreement under which the counterparty is not required to post variation margin. In the latter case, C would also include the negative amount of the variation margin that the banking organization posted to the counterparty (thus increasing replacement cost).

For netting sets subject to a variation margin agreement under which the counterparty must post variation margin, the replacement cost, as provided under § _.132(c)(6)(i) of the proposed rule, would equal the greater of (1) the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts; (2) the sum of the variation margin threshold and the minimum transfer amount applicable to the derivative contracts within the netting set less the net independent collateral amount applicable to such derivative contracts; or (3) zero. This can be represented as follows:

replacement cost = max{V−C; VMT + MTA−NICA; 0}

Where:

V is the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

VMT is the variation margin threshold applicable to the derivative contracts within the netting set;

MTA is the minimum transfer amount applicable to the derivative contracts within the netting set; and

C is the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts.

NICA is the net independent collateral amount applicable to such derivative contracts.

The requirement for the replacement cost of a netting set subject to a variation margin agreement is designed to account for the maximum possible unsecured exposure amount of the netting set that would not trigger a variation margin call. For example, a derivative contract with a high variation margin threshold would have a higher replacement cost compared to an equivalent derivative contract with a lower variation margin threshold. Section _.2 of the proposed rule would define the variation margin threshold and the minimum transfer amount. The variation margin threshold would mean the amount of the credit exposure of a banking organization to its counterparty that, if exceeded, would require the counterparty to post variation margin to the banking organization. The minimum transfer amount would mean the smallest amount of variation margin that may be transferred between counterparties to a netting set.

In the agencies' experience, variation margin agreements can include variation margin thresholds that are set at such high levels that the netting set is effectively unmargined since the counterparty would never breach the threshold and be required to post variation margin. The agencies are concerned that in such a case the variation margin threshold would result in an unreasonably high replacement cost, because it is not attributable to the risk associated with the derivative contract but rather the terms of the variation margin agreement. Therefore, the proposal would cap the exposure amount of a netting set subject to a variation margin agreement at the exposure amount of the same netting set calculated as if the netting set were not subject to a variation margin agreement.^[28]

For a netting set that is subject to multiple variation margin agreements, or a hybrid netting set, a banking organization would determine replacement cost using the methodology described in § _.132(c)(11)(i) of the proposed rule. A hybrid netting set is a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement. In particular, a banking organization would use the methodology described in § _.132(c)(6)(ii) for netting sets subject to a variation margin agreement, except that the variation margin threshold would equal the sum of the variation margin thresholds of all the variation margin agreements within the netting set and the minimum transfer amount would equal the sum of the minimum transfer amounts of all the variation margin agreements within the netting set.

For multiple netting sets subject to a single variation margin agreement, a banking organization would assign a single replacement cost to the multiple netting sets, according to the following formula, as provided under § _.132(c)(10)(i) of the proposed rule:

Replacement Cost = max{Σ_NSmax{V_NS; 0}−max{C_MA; 0}; 0} + max{Σ_NSmin{V_NS; 0}−min{C_MA; 0}; 0},

Where:

NS is each netting set subject to the variation margin agreement MA;

V_NS is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set NS; and

C_MA is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting sets subject to the single variation margin agreement.

The component max{S_NSmax{V_NS; 0}−max{C_MA; 0}; 0} reflects the exposure amount produced by the netting sets that have current positive market value. The exposure amount can be offset by variation margin and independent collateral when the banking organization is the net receiver of such amounts (i.e., when C_MA is positive). However, netting sets that have current negative market value would not be allowed to offset the exposure amount. The component max{S_NSmin{V_NS; 0}−min{C_MA; 0}; 0} reflects the exposure amount produced when the banking organization posts variation margin and independent collateral to its counterparty (i.e., this component contributes to replacement cost only in instances when C_MA is negative), and the exposure amount would be offset by the netting sets that have current negative market value.

Question 4: What are the potential consequences of the proposal to cap the exposure amount for a netting set subject to a variation margin agreement at the exposure amount for such netting set in the absence of a variation margin agreement?

Question 5: What are the potential consequences of the proposal to exclude from the fair value amount of the derivative contract any valuation adjustments? What are the potential consequences of instead using the market value of the derivative contract less any valuation adjustments that are specific to the banking organization?

Question 6: The agencies invite comment on the proposed alignment of the standard supervisory haircuts with the maturity factor adjustments. How could the agencies better align the standard supervisory haircuts under the capital rule with the maturity factor adjustments provided under SA-CCR?

Question 7: The agencies invite comment on the proposed definitions included in this proposal. What, if any, alternative definitions should the agencies consider, particularly to achieve greater consistency across other agencies' regulations?

3. Aggregated Amount and Hedging Set Amounts

Under § _.132(c)(7) of the proposed rule, the PFE of a netting set would be the product of the PFE multiplier and the aggregated amount. The proposal would define the aggregated amount as the sum of all hedging set amounts within the netting set. This can be represented as follows:

PFE = PFE multiplier * aggregated amount,

Where:

aggregated amount is the sum of each hedging set amount within the netting set.

To determine the hedging set amounts, a banking organization would first group into separate hedging sets derivative contracts that share similar risk factors based on the following asset classes: Interest rate, exchange rate, credit, equity, and commodities. Basis derivative contracts and volatility derivative contracts would require separate hedging sets. A banking organization would then determine each hedging set amount using asset-class specific formulas that allow for full or partial netting. If the risk of a derivative contract materially depends on more than one risk factor, whether interest rate, exchange rate, credit, equity, or commodity risk factor, a banking organization's primary federal regulator ^[29] may require the banking organization to include the derivative contract in each appropriate hedging set. The hedging set amount of a hedging set composed of a single derivative contract would equal the absolute value of the adjusted derivative contract amount of the derivative contract.

Section _.132(c)(2)(iii) of the proposal provides the respective hedging set definitions. Specifically, an interest rate hedging set would mean all interest rate derivative contracts within a netting set that reference the same reference currency. Thus, there would be as many interest rate hedging sets in a netting set as distinct currencies referenced by the interest rate derivative contracts. A credit derivative hedging set would mean all credit derivative contracts within a netting set. Similarly, an equity derivative hedging set would mean all equity derivative contracts within a netting set. Thus, there could be at most one equity hedging set and one credit hedging set within a netting set. A commodity derivative contract hedging set would mean all commodity derivative contracts within a netting set that reference one of the following commodity classes: Energy, metal, agricultural, or other commodities. Thus, there could be no more than four commodity derivative contract hedging sets within a netting set.

The proposal would define an exchange rate hedging set as all exchange rate derivative contracts within a netting set that reference the same currency pair. Thus, under this approach, there could be as many exchange rate hedging sets within a netting set as distinct currency pairs referenced by the exchange rate derivative contracts. This treatment would be generally consistent with the Basel Committee's standard. The agencies recognize, however, that the proposed approach to grouping exchange rate derivative contracts into hedging sets would not recognize economic relationships of exchange rate chains (i.e., when more than one currency pair can offset the risk of another). For example, a Yen/Dollar forward contract and a Dollar/Euro forward contract, taken together, may be economically equivalent, with properly set notional amounts, to a Yen/Euro forward contract. To capture this economic relationship, the agencies are seeking comment on an alternative definition of an exchange rate hedging set that differs from the one in the Basel Committee's standard. Under the alternative definition, an exchange rate derivative contract hedging set would mean all exchange rate derivative contracts within a netting set that reference the same non-U.S. currency. Thus, a banking organization would be required, under the proposed alternative definition, to include in separate hedging sets an exchange rate derivative contract that references two or more foreign currencies. For example, a banking organization would include the Yen/Euro forward contract both in one hedging set consisting of Yen derivative contracts and another hedging set consisting of Euro derivative contracts. Under this alternative approach, there could be as many exchange rate derivative contract hedging sets as non-U.S. referenced currencies.

The proposal sets forth treatments for volatility derivative contracts and basis derivative contracts separate from the treatment for the risk factors described above. A basis derivative contract would mean a non-foreign-exchange derivative contract (i.e., the contract is denominated in a single currency) in which the cash flows of the derivative contract depend on the difference between two risk factors that are attributable solely to one of the following derivative asset classes: Interest rate, credit, equity, or commodity. A basis derivative contract hedging set would mean all basis derivative contracts within a netting set that reference the same pair of risk factors and are denominated in the same currency. A volatility contract would mean a derivative contract in which the payoff of the derivative contract explicitly depends on a measure of the volatility of an underlying risk factor to the derivative contract. Examples of volatility derivative contracts include variance and volatility swaps and options on realized or implied volatility. A volatility derivative contract hedging set would mean all volatility derivative contracts within a netting set that reference one of interest rate, exchange rate, credit, equity, or commodity risk factors, separated according to the requirements under § _.132(c)(2)(iii)(A)-(E) of the proposed rule.

Question 8: Should SA-CCR include the alternative treatment for exchange rate derivative contracts in order to recognize the economic equivalence of chains of exchange rate transactions? What would be the benefit of including such an alternative treatment? Commenters providing information regarding an alternative treatment are encouraged to provide support for such treatment, together with information regarding any associated burden and complexity.

a. Interest Rate Derivative Contracts

The hedging set amount for interest rate derivative contracts would be determined under § _.132(c)(8)(i) of the proposed rule. The agencies recognize that interest rate derivative contracts with close tenors (i.e., the amount of time remaining before the end date of the derivative contract) are generally highly correlated, and thus provide a greater offset relative to interest rate derivative contracts that do not have close tenors. Accordingly, the formula to determine the hedging set amount for interest rate derivative contracts would permit full offsetting within a tenor category, and partial offsetting across tenor categories. The tenor categories are less than one year, between one and five years, and more than five years. The proposal would use a correlation factor of 70 percent across adjacent tenor categories and a correlation factor of 30 percent across nonadjacent tenor categories.^[30] The tenor of a derivative contract would be based on the period between the present date and the end date of the derivative contract, which, under the proposal, would mean the last date of the period referenced by the derivative contract, or if the derivative contract references another instrument, the period referenced by the underlying instrument.

Accordingly, a banking organization would calculate the hedging set amount for interest rate derivative contracts according to the following formula:

The proposal also includes a simpler formula that does not provide an offset across tenor categories. In this case, the hedging set amount of the interest rate derivative contracts would equal the sum of the absolute amounts of each tenor category, which would be the sum of the adjusted derivative contract amounts within each respective tenor category. The simpler formula would always result in a more conservative measure of the hedging set amount for interest rate derivative contracts of different tenor categories but may be less burdensome for banking organizations with smaller interest rate derivative contract portfolios. Under the proposal, a banking organization could elect to use this simpler formula for some or all of its interest rate derivative contracts.

b. Exchange Rate Derivative Contracts

The hedging set amount for exchange rate derivative contracts would be determined under § _.132(c)(8)(ii) of the proposed rule. The agencies recognize that exchange rate derivative contracts that reference the same currency pair generally are driven by the same market factor (i.e., the exchange spot rate between these currencies) and thus are highly correlated. Therefore, the formula to determine the hedging set amount for exchange rate derivative contracts would allow for full offsetting within the exchange rate derivative contract hedging set. Accordingly, the hedging set amount for exchange rate derivative contracts would equal the absolute value of the sum of the adjusted derivative contract amounts within the hedging set.

c. Credit Derivative Contracts and Equity Derivative Contracts

A banking organization would use the same formula to determine the hedging set amount for both its credit derivative contracts and equity derivative contracts. The formula would be provided under § _.132(c)(8)(iii) of the proposed rule. The formula would allow for full offsetting for credit or equity contracts referencing the same entity, and would use a single-factor model to allow for partial offsetting when aggregating across distinct reference entities. The proposed single-factor model recognizes that credit spreads and equity prices of different entities within a hedging set are, on average, positively correlated.^[31] The proposed single-factor model would use a single systematic component to describe joint movement of credit spreads or equity prices that are responsible for positive correlations, and would use an idiosyncratic component to describe entity-specific dynamics of each derivative contract.

The proposal would provide supervisory correlation parameters for credit derivative contracts and equity derivative contracts that depend on whether the derivative contract references a single name entity or an index. A single name entity credit derivative and a single name entity equity derivative would receive a correlation factor of 50 percent, while a credit index and equity index would receive a correlation factor of 80 percent, the higher number reflecting partial diversification of idiosyncratic risk within an index. The pairwise correlation between two entities is the product of the corresponding correlation factors, so that the pairwise correlation between two single name entities is 25 percent, between one single name entity and one index is 40 percent, and between two indices is 64 percent. Thus, the pairwise correlation between two single name entities is less than the pairwise correlation between an entity and an index, which is less than the pairwise correlation between two indices. The application of a higher correlation factor does not necessarily result in a higher exposure amount, as there would be a reduction of the exposure amount for balanced portfolios but an increase in the exposure amount for directional portfolios.^[32]

A banking organization would calculate the hedging set amount for a credit derivative contract hedging set or an equity derivative contract hedging set according to the following formula:

Where:

k is each reference entity within the hedging set;

K is the number of reference entities within the hedging set;

AddOn  (Ref_k) equals the sum of the adjusted derivative contract amounts for all derivative contracts within the hedging set that reference reference entity k; and

r_k equals the applicable supervisory correlation factor, as provided in Table 2.

d. Commodity Derivative Contracts

A banking organization would use a similar single-factor model to determine the hedging set amount for commodity derivative contracts as it would use for credit derivative contracts and equity derivative contracts. The hedging set amount of commodity derivative contracts would be determined under § _.132(c)(8)(iv) of the proposed rule. Under the proposal, a banking organization would group commodity derivatives into one of four hedging sets based on the following commodity classes: Energy, metal, agricultural and other. Under the single-factor model used for commodity derivative contracts, a banking organization would be able to offset fully all derivative contracts within a hedging set that reference the same commodity type; however, the banking organization could only partially offset derivative contracts within a hedging set that reference different commodity types. For example, a hedging set composed of energy commodities may include crude oil derivatives and coal derivatives. Under the proposal, a banking organization could fully offset all crude oil derivatives; however, it could only partially offset a crude oil derivative against a coal derivative. In addition, a banking organization cannot offset commodity derivatives that belong to different hedging sets (i.e., a forward contract on crude oil cannot hedge a forward contract on corn).

The agencies recognize that specifying individual commodity types is operationally difficult. Indeed, it is likely impossible to specify sufficiently all relevant distinctions between commodity types so that all basis risk is captured. Accordingly, the proposal would allow banking organizations to recognize commodity types without regard to characteristics such as location or quality. For example, a banking organization may recognize crude oil as a commodity type, and would not need to distinguish further between West Texas Intermediate and Saudi Light crude oil. The agencies expect to monitor the commodity-type distinctions made within the industry to ensure that they are sufficiently correlated for full-offset treatment under SA-CCR.

The agencies are proposing not to provide separate supervisory factors for electricity and oil/gas components of the energy commodity class, as provided under the Basel Committee standard. Rather, the agencies are proposing to provide a single supervisory factor for an energy commodity class that generally would include derivative contracts that reference electricity and oil/gas. In addition, the agencies are proposing not to provide more granular commodity categories than those provided under the Basel Committee's standard. The agencies believe that more granular commodity classes could pose operational challenges for banking organizations and could negate certain hedging benefits that may otherwise be available. This is because SA-CCR only permits offsetting within commodity classes, and additional commodity classes thereby may reduce the derivative contracts across which a banking organization may hedge.

A banking organization would calculate the hedging set amount for a commodity derivative contract hedging set according to the following formula:

Where:

k is each commodity type within the hedging set;

K is the number of commodity types within the hedging set;

AddOn (Type_k) equals the sum of the adjusted derivative contract amounts for all derivative contracts within the hedging set that reference commodity type k; and

r equals the applicable supervisory correlation factor, as provided in Table 2.

Question 9: What other commodity classes should the agencies consider for hedging set treatment, taking into account operational challenges for banking organizations and potential hedging benefits of the derivative contracts? What would be the consequences of not specifying the commodity types within each commodity class that are eligible for full offsetting? What level of granularity regarding the attributes of a commodity type would be required to appropriately distinguish among them?

4. PFE Multiplier

Under SA-CCR, the aggregated amount formula would not recognize financial collateral and would assume a zero market value for all derivative contracts. However, excess collateral and negative fair value of the derivative contracts within the netting set reduce PFE. This reduction in PFE is achieved through the PFE multiplier, which would recognize, if present, the amount of excess collateral available and the negative fair value of the derivative contracts within the netting set.

Under the proposal, the PFE multiplier would decrease exponentially from a value of one as the value of the financial collateral held exceeds the net fair value of the derivative contracts within the netting set, subject to a floor of 0.05. The PFE multiplier would decrease as the net fair value of the derivative contracts within the netting set decreases below zero, to reflect that “out-of-the-money” transactions have less chance to return to a positive, “in-the-money” value. Specifically, when the component V−C is greater than zero, the multiplier would be equal to one. When the component V−C is less than zero, the multiplier would be less than one and would decrease exponentially in value as the absolute value of V−C increases. The PFE multiplier would approach the floor of 0.05 as the absolute value of V−C becomes very large as compared with the aggregated amount of the netting set. Thus, the combination of the exponential function and the floor provides a sufficient level of conservatism by prohibiting overly favorable decreases in PFE when excess collateral increases and preventing PFE from reaching zero at any amounts of margin.

Under § _.132(c)(7)(i) of the proposal, a banking organization would calculate the PFE multiplier according to the following formula:

Where:

V is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

C is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting set; and

A is the aggregated amount of the netting set.

Question 10: Can the PFE multiplier be calibrated to more appropriately recognize the risk-reducing effects of collateral and a netting set with a negative market value for purposes of the PFE calculation? Is the 5 percent floor appropriate, particularly in view of the exponential functioning of the formula for PFE multiplier, why or why not? Commenters are encouraged to provide data to support their responses.

5. PFE Calculation for Nonstandard Margin Agreements

When a single variation margin agreement covers multiple netting sets, the parties exchange variation margin based on the aggregated market value of the netting sets. Thus, netting sets with positive and negative market values can offset one another to reduce the amount of variation margin that the parties must exchange. However, a banking organization's exposure amount for a netting set is floored by zero. Thus, for purposes of determining a banking organization's aggregate exposure amount, a netting set with a negative market value cannot offset a netting set with a positive market value. Therefore, in cases when a single variation agreement covers multiple setting sets and at least one netting set has a negative market value, the amount of variation margin exchanged between the parties will be insufficient relative to the banking organization's exposure amount for the netting sets.^[33] Under § _.132(c)(10)(ii) of the proposed rule, for multiple netting sets covered by a single variation margin agreement such that the banking organization's counterparty must post variation margin, a banking organization would be required to assign a single PFE equal to the sum of PFEs for each such netting set calculated as if none of the derivative contracts within the netting set are subject to a variation margin agreement.

Since swap margin requirements came into effect in September 2016, the amounts of netting agreements that are subject to more than one variation margin agreement and hybrid netting sets have increased. While all derivative contracts within a netting set can fully offset each other in the replacement cost component calculation, regardless of whether the netting set is subject to multiple variation margin agreements or is a hybrid netting set, margined derivative contracts cannot offset unmargined derivative contracts in the PFE component calculation because of different applicable risk horizons. Similarly, derivative contracts with different MPORs cannot offset each other.

Therefore, the agencies are proposing, under § _.132(c)(11)(ii) of the proposed rule, that for a netting set subject to multiple variation margin agreements such that the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to a variation margin agreement under which the counterparty to the derivative contract must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, a banking organization must divide the netting set into sub-netting sets and calculate the aggregated amount for each sub-netting set.

All derivative contracts within the netting set that are not subject to a variation margin agreement or that are subject to a variation margin agreement under which the counterparty is not required to post variation margin would form a single sub-netting set. A banking organization would calculate the aggregated amount for this sub-netting set as if the netting set were not subject to a variation margin agreement. All derivative contracts within the netting set that are subject to variation margin agreements under which the counterparty must post variation margin and that share the same MPOR value would form another sub-netting set. A banking organization would calculate the aggregated amount for this sub-netting set as if the netting set is subject to a variation margin agreement, using the MPOR value shared by the derivative contracts within the netting set. A banking organization would calculate the PFE multiplier at the netting set level.

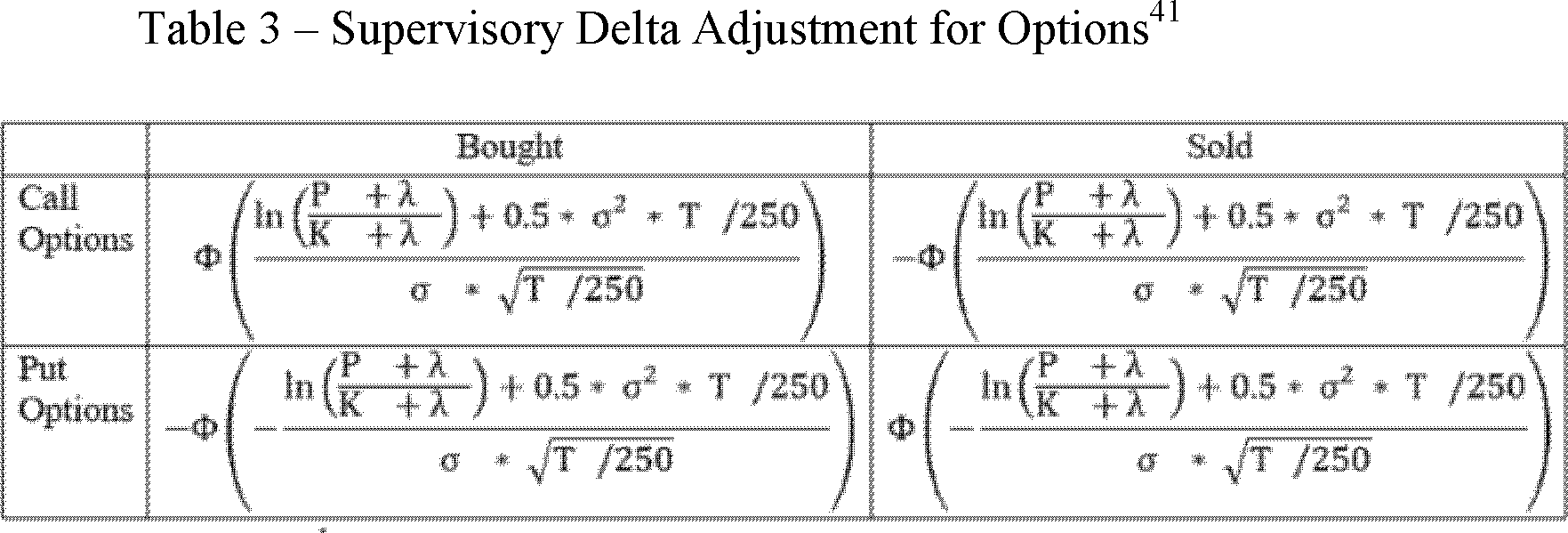
6. Adjusted Derivative Contract Amount

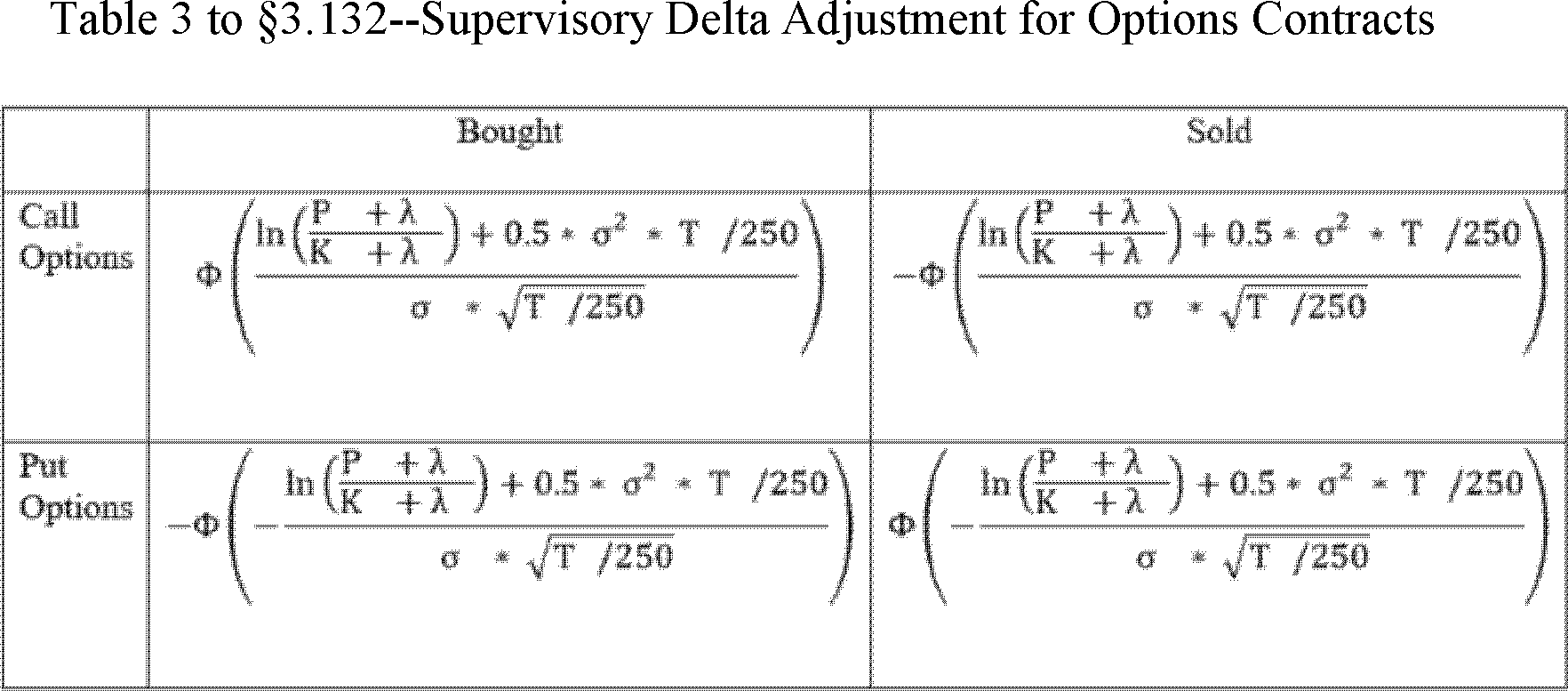
The agencies intend for the adjusted derivative contract amount to represent a conservative estimate of EEPE of a netting set consisting of a single derivative contract, assuming zero market value and zero collateral, that is either positive (if a long position) or negative (if a short position).^[34] The proposal would calculate the adjusted derivative contract amount as a product of four quantities: The adjusted notional amount, the applicable supervisory factor, the applicable supervisory delta adjustment, and the maturity factor. This can be represented as follows:

adjusted derivative contract amount = d_i * δ_i * MF _i * SF _i

Where:

d_i is the adjusted notional amount;

δ_i is the applicable supervisory delta adjustment;

MF _i is the applicable maturity factor; and

SF _i is the applicable supervisory factor.

The adjusted notional amount accounts for the size of the derivative contract and reflects attributes of the most common derivative contracts in each asset class. The supervisory factor would convert the adjusted notional amount of the derivative contract into an EEPE based on the measured volatility specific to each asset class over a one-year horizon.^[35] Multiplication by the supervisory delta adjustment accounts for the sensitivity of a derivative contract (scaled to unit size) to the underlying primary risk factor, including the correct sign (positive or negative) to account for the direction of the derivative contract amount relative to the primary risk factor.^[36] Finally, multiplication by the maturity factor scales down, if necessary, the derivative contract amount from the standard one-year horizon used for supervisory factor calibration to the risk horizon relevant for a given contract. The adjusted derivative contract amount is determined under § _.132(c)(9) of the proposed rule.

a. Adjusted Notional Amount

A banking organization would apply the same formula to interest rate derivative contracts and credit derivative contracts to arrive at the adjusted notional amount. For such contracts, the adjusted notional amount would equal the product of the notional amount of the derivative contract, as measured in U.S. dollars, using the exchange rate on the date of the calculation, and the supervisory duration. The agencies intend for the supervisory duration to recognize that interest rate derivative contracts and credit derivative contracts with a longer tenor would have a greater degree of variability than an identical derivative contract with a shorter tenor for the same change in the underlying risk factor (interest rate or credit spread).

The supervisory duration would be calculated for the period that starts at S and ends at E. S would be equal to the number of business days between the present date and the start date for the derivative contract, or zero if the start date has passed, and E would be equal to the number of business days from the present date until the end date for the derivative contract. The supervisory duration is based on the assumption of a continuous stream of equal payments and a constant continuously compounded interest rate of 5 percent. The exponential function provides discounting for S and E at 5 percent continuously compounded. In all cases, the supervisory duration is floored at 10 business days (or 0.04, based on an average of 250 business days per year).

The supervisory duration formula is provided as follows:

Where:

S is the number of business days from the present day until the start date for the derivative contract, or zero if the start date has already passed; and

E is the number of business days from the present day until the end date for the derivative contract.

For an interest rate derivative contract or credit derivative contract that is a variable notional swap, the notional amount would equal the time-weighted average of the contract notional amounts of such a swap over the remaining life of the swap. For an interest rate derivative contract or credit derivative contract that is a leveraged swap, in which the notional amounts of all legs of the derivative contract are divided by a factor and all rates of the derivative contract are multiplied by the same factor, the notional amount would equal the notional amount of an equivalent unleveraged swap.

For an exchange rate derivative contract, the adjusted notional amount would equal the notional amount of the non-U.S. denominated currency leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation. In general, the non-U.S. dollar denominated currency leg is the source of exchange rate volatility. If both legs of the exchange rate derivative contract are denominated in currencies other than U.S. dollars, the adjusted notional amount of the derivative contract would be the largest leg of the derivative contract, measured in U.S. dollars. Under the agencies' alternative approach for treating exchange rate derivative contracts discussed above, the adjusted notional amount of an exchange rate derivative contract would be the notional amount of the derivative contract that is denominated in the foreign currency of the hedging set, as measured in U.S. dollars using the exchange rate on the date of the calculation. For an exchange rate derivative contract with multiple exchanges of principal, the notional amount would equal the notional amount of the derivative contract multiplied by the number of exchanges of principal under the derivative contract. For an equity derivative contract or a commodity derivative contract, the adjusted notional amount is the product of the fair value of one unit of the reference instrument underlying the derivative contract and the number of such units referenced by the derivative contract. The proposed treatment is designed to reflect the current price of the underlying reference entity. For example, if a banking organization has a derivative contract that references 15,000 pounds of frozen concentrated orange juice currently priced at $0.0005 a pound then the adjusted notional amount would be $75.

The payoff of a volatility derivative contract generally is determined based on a notional amount and the realized or implied volatility (or variance) referenced by the derivative contract and not necessarily the unit price of the underlying reference entity. Accordingly, for an equity derivative contract or a commodity derivative contract that is a volatility derivative contract, a banking organization would be required to replace the unit price with the underlying volatility referenced by the volatility derivative contract and replace the number of units with the notional amount of the volatility derivative contract.

The agencies anticipate that for most derivative contracts banking organizations would be able to determine the adjusted notional amount using one of the formulas or methodologies described above. The agencies recognize, however, that such approaches may not be applicable to all types of derivative contracts, and that a different approach may be necessary to determine the adjusted notional amount of a derivative contract. In such a case, the agencies would expect a banking organization to consult with its appropriate federal supervisor prior to using an alternative approach to the formulas or methodologies described above.

Question 11: The agencies invite comment on the proposed approaches to determine the adjusted notional amount of derivative contracts. In particular, how can the agencies improve the approaches set forth in the proposal to determine the adjusted notional amount for nonstandard derivative contracts so that they are appropriate for such transactions, including using formulas of the market value of underlying contracts? What, if any, nonstandard derivative contracts are not addressed by the proposal, and what approaches should be used to determine the adjusted notional amount for those contracts? Please provide examples and descriptions of how such adjusted notional amounts would be determined.

b. Supervisory Factor

Table 2 to § _.132 of the proposed rule provides the proposed supervisory factors. The agencies are proposing to use the same supervisory factors provided in the Basel Committee standard, with the exception of the supervisory factors for credit derivative contracts that reference single-name entities, which are based on the applicable credit rating of the reference entity.^[37] Section 939A of the Dodd-Frank Wall Street Reform and Consumer Protection Act (Dodd-Frank Act) prohibits the use of credit ratings in federal regulations, and therefore, the agencies are unable to propose implementing this feature of the Basel Committee standard.^[38] Accordingly, the agencies are proposing an approach that satisfies the requirements of section 939A while allowing for a level of granularity among the supervisory factors applicable to single-name credit derivatives that is generally consistent with the Basel Committee standard.

Specifically, the agencies are proposing to apply a supervisory factor to single-name credit derivative contracts based on the following categories: Investment grade, speculative grade, and sub-speculative grade. For credit derivative contracts that reference indices, the agencies are proposing to apply a higher supervisory factor to speculative grade indices than investment grade indices, because of the additional risk present with speculative grade credits. The proposal would maintain the current definition of investment grade in the capital rule and would propose new definitions for speculative grade and sub-speculative grade.

The investment grade category would capture single-name credit derivative contracts consistent with the three highest supervisory factor categories under the Basel Committee standard. The capital rule defines investment grade to mean that the entity to which the banking organization is exposed through a loan or security, or the reference entity with respect to a credit derivative contract, has adequate capacity to meet financial commitments for the projected life of the asset or exposure. Such an entity or reference entity has adequate capacity to meet financial commitments, as the risk of its default is low and the full and timely repayment of principal is expected.^[39]

The agencies intend for the speculative grade category to cover single-name credit derivative contracts consistent with the next two lower supervisory factor categories under the Basel Committee standard. The proposal would define speculative grade to mean that the reference entity has adequate capacity to meet financial commitments in the near term, but is vulnerable to adverse economic conditions, such that should economic conditions deteriorate, the reference entity would present an elevated default risk. The agencies intend for the sub-speculative grade category to cover the lowest supervisory factor category under the Basel Committee standard. The proposal would define sub-speculative grade to mean that the reference entity depends on favorable economic conditions to meet its financial commitments, such that should economic conditions deteriorate, the reference entity likely would default on its financial commitments. The agencies believe that each of the proposed categories include exposures that perform largely in accordance with the performance criteria that would define each category under the proposed rule, and therefore would result in capital requirements that are largely equivalent to those resulting from application of the supervisory factors under the Basel Committee standard.

To determine the supervisory factor that would apply to the investment and speculative grade categories, the agencies reviewed ratings issuance data from 2012 to 2017, using information made publicly available by the Depository Trust & Clearing Corporation (DTCC).^[40] The agencies used the DTCC data to determine the weighted-average supervisory factor for the investment and speculative grade categories, and rounded that supervisory factor to the nearest tenth. The agencies are proposing to retain the supervisory factor from the Basel Committee standard for the sub-speculative grade category, because that category would consist only of single name credit derivatives with the lowest credit quality.

The agencies considered using the same investment grade/non-investment grade distinction as provided under the standardized approach for determining whether a guarantor is an eligible guarantor for purposes of the rule. However, the agencies are concerned that this approach would not provide for sufficient risk differentiation across credit derivative products. The agencies also considered calibrating the supervisory factor for the investment and speculative grade categories by using a simple average of the ratings issued in accordance with the DTCC data, or the most conservative supervisory factor applicable to the credit ratings that mapped to each category. For example, if for purposes of the investment grade category the DTCC data demonstrated that the average rating in that category is AA (using a simple average of all ratings issued for single-name credit derivatives), the proposal would apply a 0.38 percent supervisory factor to investment grade single-name credit derivatives, because that supervisory factor corresponds to a AA rating under the Basel Committee standard. Under the other alternative considered, the proposal would apply the most conservative (i.e., stringent) supervisory factor among the supervisory factors that apply to a given category. Under this approach, a supervisory factor of 1.6 percent would apply to speculative grade single-name credit derivatives, as that is the most stringent supervisory factor under the Basel Committee standard that corresponds to the categories intended to be captured by the term “speculative grade.” The agencies believe, however, that the weighted-average approach more accurately reflects the ratings issuance data and therefore would more closely align to the single-name credit derivatives held in banking organizations' derivatives portfolios.

The agencies expect that banking organizations would conduct their own due diligence to determine the appropriate category for a single-name credit derivative, in view of the performance criteria in the definitions for each category under the proposed rule. Although a banking organization would be able to consider the credit rating for a single-name credit derivative in making that determination, the credit rating should be part of a multi-factor analysis. In addition, the agencies would expect a banking organization to support its analysis and assignment of the respective credit categories.

Interest rate derivative contracts and exchange rate derivative contracts would each be subject to a single supervisory factor. Equity derivative contracts that reference single-name equities would be subject to a higher supervisory factor than derivative contracts that reference equity indices in recognition of the effect of diversification in the index. Commodity derivative contracts that reference energy would receive a higher supervisory factor than commodity derivative contracts that reference metals, agriculture, and other commodities (each of which would receive the same supervisory factor), to reflect the observed additional volatility inherent in the energy markets.

For volatility derivative contracts, a banking organization would multiply the applicable supervisory factor based on the asset class related to the volatility measure by a factor of five. The agencies are proposing this treatment because volatility derivative contracts are inherently subject to more price volatility than the underlying asset classes they reference. For basis derivative contracts, the agencies are proposing to multiply the applicable supervisory factor based on the asset class related to the basis measure by a factor of one half. The agencies are proposing this treatment because the volatility of a basis between highly correlated risk factors would be less than the volatility of the risk factors (assuming the factors have equal volatility).

Table 2—Supervisory Option Volatility and Supervisory Factors for Derivative Contracts

Asset class	Subclass	Supervisory option volatility (%)	Supervisory correlation parameters (%)	Supervisory factor a (%)
Interest rate	N/A	50	N/A	0.5
Exchange rate	N/A	15	N/A	4.0
Credit, single name	Investment grade	100	50	0.5
	Speculative grade	100	50	1.3
	Sub-speculative grade	100	50	6.0
Credit, index	Investment Grade	80	80	0.38
	Speculative Grade	80	80	1.06
Equity, single name	N/A	120	50	32
Equity, index	N/A	75	80	20
Commodity	Energy	150	40	40
	Metals	70	40	18
	Agricultural	70	40	18
	Other	70	40	18
a The applicable supervisory factor for basis derivative contract hedging sets is equal to one-half of the supervisory factor provided in Table 2, and the applicable supervisory factor for volatility derivative contract hedging sets is equal to 5 times the supervisory factor provided in Table 2.

Question 12: Can the agencies improve the supervisory factors under the proposal to reflect more appropriately the volatility specific to each asset class? What, if any, additional categories and respective supervisory factors should the agencies consider? Commenters supporting changes to the supervisory factors or the categories within the asset classes should provide analysis supporting their request.

Question 13: Can the agencies improve the non-ratings-based methodology under the proposal to determine the supervisory factor applicable to a single-name credit derivative contract? Are there other non-ratings-based methodologies that could be used to determine the applicable supervisory factor for single-name credit derivatives? What would be the benefit of any such alternative relative to the proposal? What would be the burden associated with the proposed methodology, as well as any alternative suggested by commenters?

c. Supervisory Delta Adjustment

Under the proposal, derivative contracts that are not options or collateralized debt obligation tranches are considered to be linear in the primary underlying risk factor. For such derivative contracts, the supervisory delta adjustment would need to account only for the direction of the derivative contract (positive or negative) with respect to the underlying risk factor. Therefore, the supervisory delta adjustment would be equal to one if such a derivative contract is long in the primary risk factor and negative one if such a derivative contract is short in the primary risk factor. A derivative contract is long in the primary risk factor if the fair value of the instrument increases when the value of the primary risk factor increases. A derivative contract is short in the primary risk factor if the fair value of the instrument decreases when the value of the primary risk factor increases.

Because option contracts are nonlinear, the proposal would require a banking organization to use the Black-Scholes Model to determine the supervisory delta adjustment, as provided in Table 2. The agencies are proposing to use the Black-Scholes Model to determine the supervisory delta adjustment because the model is a widely used option-pricing model within the industry. The Black Scholes-Model assumes, however, that the underlying risk factor is greater than zero. In particular, the Black Scholes delta formula contains a ratio P/K that is an input into the natural logarithm function. P is the fair value of the underlying instrument and K is the strike price. Because the natural logarithm function can be defined only for amounts greater than zero, a reference risk factor with a negative value (e.g., negative interest rates) would make the supervisory delta adjustment inoperable. Therefore, the formula incorporates a parameter, lambda, the purpose of which is to adjust the fraction P/K so that it has a positive value.

Where:

F is the standard normal cumulative distribution function;

P equals the current fair value of the instrument or risk factor, as applicable, underlying the option;

K equals the strike price of the option;

T equals the number of business days until the latest contractual exercise date of the option; and

l equals zero for all derivative contracts, except that for interest rate options that reference currencies currently associated with negative interest rates l must be equal to; max {−L + 0.1%; 0}; ^[42]

and σ equals the supervisory option volatility, determined in accordance with Table 2.

For a derivative contract that is a collateralized debt obligation tranche, the supervisory delta adjustment would be determined according to the following formula:

Where:

A is the attachment point, which equals the ratio of the notional amounts of all underlying exposures that are subordinated to the banking organization's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; ^[43]

D is the detachment point, which equals one minus the ratio of the notional amounts of all underlying exposures that are senior to the banking organization's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; and

The proposal would apply a positive sign to the resulting amount if the banking organization purchased the collateralized debt obligation tranche and would apply a negative sign if the banking organization sold the collateralized debt obligation tranche.

d. Maturity Factor

For derivative contracts not subject to a variation margin agreement, or derivative contracts subject to a variation margin agreement under which the counterparty to the variation margin agreement is not required to post variation margin to the banking organization, the risk horizon would be the lesser of one year and the remaining maturity of the derivative contract, subject to a 10-business-day floor. Accordingly, for such a derivative contract, a banking organization would use the following formula:

Where M equals the greater of 10 business days and the remaining maturity of the contract, as measured in business days.

For derivative contracts subject to a variation margin agreement under which the counterparty must post variation margin, the risk horizon would be equal to the MPOR of the variation margin agreement. Accordingly, for such a derivative contract a banking organization would use the following formula:

Where MPOR refers to the period from the most recent exchange of collateral under a variation margin agreement with a defaulting counterparty until the derivative contracts are closed out and the resulting market risk is re-hedged.

For derivative contracts that are not cleared transactions, MPOR would be floored at 10 business days. For derivative contracts between a clearing member banking organization and its client that are cleared transactions, MPOR would be floored at five business days. Under the capital rule, however, the exposure of a clearing member banking organization to its clearing member client is not a cleared transaction where the clearing member banking organization is either acting as a financial intermediary and enters into an offsetting transaction with a CCP or where the clearing member banking organization provides a guarantee to the CCP on the performance of the client. Accordingly, in such cases, MPOR may not be less than 10 business days. If either a cleared or noncleared derivative contract is subject to an outstanding dispute over variation margin, the applicable MPOR would be twice the MPOR provided for those transactions in the absence of such a dispute.^[44] For a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, MPOR would be floored at 20 business days.

For a derivative contract in which on specified dates any outstanding exposure of the derivative contract is settled and the terms of the derivative contract are reset so that the fair value of the derivative contract is zero, the remaining maturity of the derivative contract is the period until the next reset date.^[45] In addition, derivative contracts with daily settlement would be treated as unmargined derivative contracts.

7. Example Calculation ⁴⁶

To calculate the exposure amount of a netting set a banking organization would need to determine (1) the replacement cost, (2) the adjusted derivative contract amount of each derivative contract within the netting set, (3) the aggregated amount, which is the sum of each hedging set within the netting set, (4) the PFE multiplier, and (5) PFE. A banking organization may calculate these items together for derivative contracts that are subject to the same QMNA.

In this example, the netting set consists of two fixed versus floating interest rate swaps that are subject to the same QMNA. Table 4 summarizes the relevant contractual terms for these derivative contracts. The netting set is subject to a variation margin agreement, and the banking organization has received from the counterparty, as of the calculation date, variation margin in the amount of $10,000 and initial margin in the amount of $200,000. Both the variation margin threshold and the minimum transfer amount are zero. All notional amounts and market values in Table 4 are denominated in U.S. Dollars.

Table 4—Contractual Terms for the Derivative Contracts

Derivative	Type	Residual maturity (years)	Base currency	Pay leg	Notional (thousands)	Fair value excluding valuation adjustments (thousands)
1	Interest rate swap	10	USD	Fixed	$10,000	$30
2	Interest rate swap	4	USD	Floating	10,000	−20

Step 1: Determine the Replacement Cost

Under § _.132(c)(6)(i) of the proposed rule, the replacement cost of a netting set subject to a variation margin agreement would equal the greater of (1) the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts; (2) the sum of the variation margin threshold and the minimum transfer amount applicable to the derivative contracts within the netting set less the net independent collateral amount applicable to such derivative contracts; and (3) zero.

The replacement cost of the netting set in the example is given as follows:

RC = max{(30−20)−(200 + 10); 0 + 0 − 200; 0} = 0

Step 2: Determine the Adjusted Derivative Contract Amount of Each Derivative Contract Within the Netting Set

A banking organization would determine the adjusted derivative contract amount of each derivative contract within the netting set, in accordance with § _.132(c)(9) of the proposed rule. The adjusted derivative contract amount would be the product of the adjusted notional amount, the supervisory delta adjustment, the maturity factor, and the applicable supervisory factor, which are given as follows:

Adjusted derivative contract amount_i^iR = d_i^IR * _di * MF_i * SF_i

Under § _.132(c)(9)(ii)(A) of the proposed rule, for each derivative contract i, the adjusted notional amount would be calculated as follows:

S_i and E_i represent the number of business days from the present day until the start date and the end date, respectively, of the period referenced by the interest rate derivative contracts. The residual maturity of derivative contract 1 is 10 years and thus term E_i equals 250 multiplied by 10. The residual maturity of derivative contract 2 is 4 years and thus term E_i equals 250 multiplied by 4. Accordingly, the adjusted notional amounts for derivative contract 1 and derivative contract 2 are given as follows:

The supervisory delta adjustment would be assigned to each derivative contract in accordance with § _.132(c)(9)(iii) of the proposed rule. Derivative contract 1 is long in the primary risk factor and is not an option; therefore, the supervisory delta is equal to one. Derivative contract 2 is short in the primary risk factor and is not an option; therefore, the supervisory delta is equal to negative one.

The maturity factor would be assigned to each derivative contract in accordance with § _.132(c)(9)(iv)(A) of the proposed rule. Assuming a MPOR of 15 business days, the maturity factor is given as follows:

The supervisory factor for interest rate derivative contracts is 0.50 percent, as provided in Table 2.

For derivative contract 1, the adjusted derivative contract amount would equal 1 * 78,694 * 0.3674 * 0.50% = 144.57. For derivative contract 2, the adjusted derivative contract amount equals −1 * 36,254 * 0.3674 * 0.50% = −66.60.

Step 3: Determine the Hedging Set Amount

A banking organization would determine the hedging set amount for interest rate derivative contracts in accordance with § _.134(c)(8)(i) of the proposed rule, as follows:

Step 4: Determine the Aggregated Amount

Because the netting set includes only one hedging set, the aggregated amount is equal to 108.89.

Step 5: Determine the PFE Multiplier

A banking organization would calculate the PFE multiplier in accordance with § _.132(c)(7)(i) of the proposed rule, as follows:

Where:

(A) V is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

(B) C is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting set

(C) A is the aggregated amount of the netting set

The PFE multiplier would be given as:

Step 6: Determine PFE

In accordance with § _.132(c)(7) of the proposed rule, PFE would equal the product of the PFE multiplier and the aggregated amount. Thus, PFE would be calculated as 0.4113 * 108.89 = 44.79.

Step 7: Determine the Exposure Amount

In accordance with § _.132(c)(5) of the proposed rule, the exposure amount of a netting net would equal sum of the replacement cost of the netting set and the PFE of the netting set multiplied by 1.4. Therefore, the exposure amount of the netting set in the example would be calculated as, 1.4 * (0 + 44.79) = 62.70.

III. Revisions to the Cleared Transactions Framework

Under the cleared transactions framework in the capital rule, a banking organization is required to hold risk-based capital for its exposure to, and certain collateral posted in connection with, a derivative contract that is a cleared transaction. In addition, a clearing member banking organization must hold risk-based capital for its default fund contributions. The capital requirement for a cleared derivative contract reflects the counterparty credit risk of the derivative contract, whereas the capital requirement for collateral posted in connection with such a derivative contract reflects the risk that a banking organization may not be able to recover its collateral upon default of the entity holding the collateral. The capital requirement for a default fund contribution reflects the risk that a clearing member banking organization may incur loss on such contribution resulting from the CCP's or another clearing member's default. In addition, in recognition of the credit risk of the collateral itself, a banking organization must calculate a risk-weighted asset amount for any collateral provided to a CCP, clearing member, or a custodian in connection with a cleared transaction.

In general, the risk-based capital treatment under the cleared transactions framework distinguishes between derivative contracts cleared through a CCP and those cleared through a QCCP, whether the derivative contract is with a clearing member or clearing member client, and, with respect to collateral, the treatment depends on whether the collateral is held in a bankruptcy remote manner. Compared to transactions cleared through a CCP, those involving a QCCP generally are considered to be less risky, because to qualify as a QCCP for purposes of the capital rule a central counterparty must meet certain risk-management, supervision, and other requirements.^[47] For purposes of the capital rule, “bankruptcy remote” generally means that collateral posted by a clearing member to a CCP would be excluded from the CCP's estate in receivership, insolvency, liquidation, or similar proceeding, and thus the banking organization would be more likely to recover such collateral upon the CCP's default.

The agencies are proposing to revise the cleared transactions framework under the capital rule by requiring certain banking organizations to use SA-CCR to determine the trade exposure amount for a cleared derivative contract. In addition, the agencies are proposing to simplify the formula used to determine the risk-weighted asset amount for a default fund contribution. The proposed revisions are consistent with standards developed by the Basel Committee.^[48]

Notwithstanding the proposed implementation of SA-CCR, the requirements under the capital rule regarding the treatment of cleared derivative contracts, including the definition for cleared transactions and the operational requirements for cleared derivative contracts, would still apply irrespective of whether the exposure is associated with a CCP or a QCCP.^[49]

A. Trade Exposure Amount

To determine the risk-weighted asset amount for a cleared derivative contract, a banking organization must multiply the trade exposure amount of the derivative contract by the risk weight applicable to the CCP. In general, the trade exposure amount is the sum of the exposure amount of the derivative contract and the fair value of any related collateral held in a manner that is not bankruptcy remote. Under the standardized approach, a banking organization must use CEM to determine the trade exposure amount of its derivative contracts, whereas under the advanced approaches, an advanced approaches banking organization may use CEM or IMM to determine the trade exposure amount.

Consistent with the proposal to replace the use of CEM with SA-CCR in the advanced approaches for determining the exposure amount for a noncleared derivative contract, the agencies are proposing to require advanced approaches banking organizations to use SA-CCR or IMM to determine the trade exposure amount for a cleared derivative contract. Thus, an advanced approaches banking organization would be required to use the same approach (SA-CCR or IMM) for both noncleared and cleared derivative contracts. As noted above, the agencies believe that requiring an advanced approaches banking organization to use either SA-CCR or IMM for all purposes under the advanced approaches would facilitate regulatory reporting and the supervisory assessment of a banking organization's capital management program. In addition, for purposes of the standardized approach, an advanced approaches banking organization would be required to use SA-CCR to determine the trade exposure amount of its cleared derivative contracts.

For non-advanced approaches banking organizations, the proposal would permit the use of CEM or SA-CCR to determine the trade exposure amount for a derivative contract. However, similar to the uniformity requirement for the elections of advanced approaches banking organizations, a non-advanced approaches banking organization that elects to use SA-CCR for purposes of determining the exposure amount of a derivative contract (under § _.34 of the capital rule) would also be required to use SA-CCR (instead of CEM) to determine the trade exposure amount for a cleared derivative contract under the cleared transactions framework. Similarly, a non-advanced approaches banking organization that continues to use CEM under § _.34 of the proposed capital rule would continue to use CEM to determine the trade exposure amount of all its derivative contracts.

Question 14: Should the agencies maintain the use of CEM for purposes of the cleared transactions framework under the advanced approaches? What other factors should the agencies consider in determining whether SA-CCR is a more or less appropriate approach for calculating the trade exposure amount for derivative transactions with central counterparties?

Question 15: What would be the pros and cons of allowing advanced approaches banking organizations to use either SA-CCR or IMM for purposes of determining the risk-weighted asset amount of both centrally and noncentrally cleared derivative transactions?

B. Treatment of Default Fund Contributions

Under the capital rule, a clearing member banking organization must determine a risk-weighted asset amount for its default fund contributions according to one of three approaches. A clearing member banking organization's risk-weighted asset amount for its default fund contributions to a CCP that is not a QCCP generally is the sum of such default fund contributions multiplied by 1,250 percent. A clearing member banking organization's risk-weighted asset amount for its default fund contributions to a QCCP equals the sum of its capital requirement for each QCCP to which a banking organization contributes to a default fund, as calculated under one of two methods. Method one is a complex three-step approach that compares the default fund of the QCCP to the capital the QCCP would be required to hold if it were a banking organization and provides a method to allocate the default fund deficit or excess back to the clearing member. Method two is a simplified approach in which the risk-weighted asset amount for a default fund contribution to a QCCP equals 1,250 percent multiplied by the default fund contribution, subject to a cap.

The proposal would eliminate method one and method two under the capital rule and implement a new method for a clearing member banking organization to determine the risk-weighted asset amount for its default fund contributions to a QCCP. The agencies intend for the new method to be less complex than the current method one but also more granular than the current method two. Under the proposal, the risk-weighted asset amount for a clearing member banking organization's default fund contribution would be its pro-rata share of the QCCP's default fund.

To determine the capital requirement for a default fund contribution, a clearing member banking organization would first calculate the hypothetical capital requirement of the QCCP (K_CCP), unless the QCCP has already disclosed it, in which case the banking organization must rely on that disclosed figure. In either case, a banking organization may choose to use a higher amount of K_CCP than the minimum calculated under the formula if the banking organization has concerns about the nature, structure, or characteristics of the QCCP. In effect, K_CCP would serve as a consistent measure of a QCCP's default fund amount.

A clearing member banking organization would calculate K_CCP according to the following formula:

K_CCP = S_CMiEAD_i * 1.6 percent,

Where:

CM_i is each clearing member of the QCCP; and

EAD_i is the exposure amount of each clearing member of the QCCP to the QCCP, as determined under § _.133(d)(6).

The component EAD_i would include both the clearing member banking organization's own transactions, its client transactions guaranteed by the clearing member, and all values of collateral held by the QCCP (including the clearing member banking organization's pre-funded default fund contribution against these transactions).^[50] The amount 1.6 percent represents the product of a capital ratio of 8 percent and a 20 percent risk weight of a clearing member banking organization, which is equal to the sum of the 2 percent capital requirement for trade exposure plus 18 percent for the default fund portion of a banking organization's exposure to a QCCP.

A banking organization that is required to use SA-CCR to determine the exposure amount for its derivative contracts under the standardized approach would be required to use SA-CCR to calculate K_CCP for both the standardized approach and the advanced approaches.^[51] For purposes of calculating K_CCP, the PFE multiplier would include collateral held by a QCCP in which the QCCP has a legal claim in the event of the default of the member or client, including default fund contributions of that member. In addition, a banking organization would use a MPOR of 10 days in the maturity factor adjustment. A banking organization that elects to use CEM to determine the exposure amount of its derivative contracts under the standardized approach would use CEM to calculate K_CCP.

EAD must be calculated separately for each clearing member's sub-client accounts and sub-house account (i.e., for the clearing member's propriety activities). If the clearing member's collateral and its client's collateral are held in the same account, then the EAD of that account would be the sum of the EAD for the client-related transactions within the account and the EAD of the house-related transactions within the account. In such a case, for purposes of determining such EADs, the independent collateral of the clearing member and its client would be allocated in proportion to the respective total amount of independent collateral posted by the clearing member to the QCCP. This treatment would protect against a clearing member recognizing client collateral to offset the CCP's exposures to the clearing members' proprietary activity in the calculation of K_CCP.

In addition, if any account or sub-account contains both derivative contracts and repo-style transactions, the EAD of that account is the sum of the EAD for the derivative contracts within the account and the EAD of the repo-style transactions within the account. If independent collateral is held for an account containing both derivative contracts and repo-style transactions, then such collateral must be allocated to the derivative contracts and repo-style transactions in proportion to the respective product specific exposure amounts. The respective product specific exposure amounts would be calculated, excluding the effects of collateral, according to § _.132(b) of the capital rule for repo-style transactions and to § _.132(c)(5) for derivative contracts. Second, a clearing member banking organization would calculate its capital requirement (K_CMi), which would be the clearing member's share of the QCCP's default fund, subject to a floor equal to a 2 percent risk weight multiplied by the clearing member banking organization's prefunded default fund contribution to the QCCP and an 8 percent capital ratio. This calculation would allocate K_CCP on a pro rata basis to each clearing member based on the clearing member's share of the overall default fund contributions. Thus, a clearing member banking organization's capital requirement would increase as its contribution to the default fund increases relative to the QCCP's own prefunded amounts and the total prefunded default fund contributions from all clearing members to the QCCP. In all cases, a banking organization's capital requirement for its default fund contribution to a QCCP may not exceed the capital requirement that would apply if the same exposure were calculated as if it were to a CCP.

A clearing member banking organization would calculate according to the following formula:

IV. Revisions to the Supplementary Leverage Ratio

Under the capital rule, an advanced approaches banking organization must satisfy a minimum supplementary leverage ratio of 3 percent. An advanced approaches banking organization's supplementary leverage ratio is the ratio of its tier 1 capital to its total leverage exposure. Total leverage exposure includes both on-balance sheet assets and certain off-balance sheet exposures.^[52] For the on-balance sheet amount, a banking organization must include the balance sheet carrying value of its derivative contracts and certain cash variation margin.^[53] For the off-balance sheet amount, the banking organization must include the PFE for each derivative contract (or each single-product netting set of derivative contracts), using CEM, as provided under § _.34 of the capital rule, but without regard to financial collateral.

The agencies are proposing to revise the capital rule to require advanced approaches banking organizations to use a modified version of SA-CCR to determine the on- and off-balance sheet amounts of derivative contracts for purposes of calculating total leverage exposure.^[54] The agencies believe that SA-CCR provides a more appropriate measure of derivative contracts for leverage capital purposes than the current approach. The agencies also are sensitive to the operational complexity that could result from requiring advanced approaches banking organizations to continue to use CEM for leverage capital purposes and another approach, SA-CCR, for risk-based capital purposes. Further, in comments on prior proposals, banking organizations have requested that the agencies adopt SA-CCR for leverage capital purposes.^[55] The proposal is consistent with the Basel Committee's standard on leverage capital requirements.^[56]

For the on-balance sheet amount, an advanced approaches banking organization would include in total leverage exposure 1.4 multiplied by the greater of (1) the sum of the fair value of the derivative contracts within a netting set less the net amount of applicable cash variation margin, or (2) zero. Consistent with CEM, an advanced approaches banking organization would be able to recognize cash variation margin in the on-balance component calculation only if (1) the cash variation margin meets the conditions under § _.10(c)(4)(ii)(C)(3)-(7) of the proposed rule; and (2) it has not been recognized in the form of a reduction in the fair value of the derivative contracts within the netting set under the advanced approaches banking organization's operative accounting standard. The proposed rule would maintain the current treatment for the recognition of cash variation margin in the supplementary leverage ratio.

A banking organization would use this same approach to determine the on-balance sheet amount for a single netting set subject to multiple variation margin agreements. To calculate the on-balance sheet amount for multiple netting sets that are subject to a single variation margin agreement or a hybrid netting set, a banking organization would use the formula under § _.132(c)(10)(i) of the proposed rule, except the term “C_MA” in § _.132(c)(10)(i)(C) would include only cash variation margin that meets the requirements under § _.10(c)(4)(ii)(C)(3)-(7) of the proposed rule.

For the off-balance sheet amount, an advanced approaches banking organization would include in total leverage exposure 1.4 multiplied by the PFE of each netting set, calculated according to § _.132(c)(7) of the proposal, except an advanced approaches banking organization would not be permitted to recognize collateral in the PFE multiplier.^[57] Thus, for purposes of calculating total leverage exposure, the term “C” under § _.132(c)(7)(i)(B) of the proposal would be equal to zero. These adjustments are consistent with the current treatment under the capital rule, which generally limits collateral recognition in leverage capital requirements, and also with the leverage standards developed by the Basel Committee. While the proposal would limit recognition of collateral in the PFE multiplier, the proposal would recognize the shorter default risk horizon applicable to margined derivative contracts. Thus, under the proposal, a netting set subject to a variation margin agreement would apply the maturity factor as provided under § _.132(c)(9)(iv) of the proposed rule.

Compared to CEM, the implementation of a modified SA-CCR for purposes of the supplementary leverage ratio would increase advanced approaches banking organizations' supplementary leverage ratios. However, the agencies are sensitive to impediments to banking organizations' willingness and ability to provide client-clearing services. The agencies also are mindful of international commitments to support the migration of derivative contracts to central clearing frameworks,^[58] the Dodd-Frank Act mandate to mitigate systemic risk and promote financial stability by, in part, developing uniform standards for the conduct of systemically important payment, clearing, and settlement activities of financial institutions.^[59] In view of these important, post-crisis reform objectives, the agencies are inviting comment on the consequences of not recognizing collateral provided by a clearing member client banking organization in connection with a cleared transaction.

Question 16: What concerns do commenters have regarding the proposal to replace the use of CEM with a modified version of SA-CCR, as proposed, for purposes of the supplementary leverage ratio?

Question 17: The agencies invite comment on the recognition of collateral provided by clearing member client banking organizations in connection with a cleared transaction for purposes of the SA-CCR methodology. What are the pros and cons of recognizing such collateral in the calculation of replacement cost and potential future exposure? Commenters should provide data regarding how alternative approaches regarding the treatment of collateral would affect the cost of clearing services, as well as provide data regarding how such approaches would affect leverage capital allocation for that activity.

V. Technical Amendments

The proposed rule would make certain technical corrections and clarifications to the capital rule to address certain provisions that warrant revision, based on questions presented by banking organizations and further review by the agencies.

A. Receivables Due From a QCCP

The agencies are proposing to revise § _.32 of the capital rule to clarify that cash collateral posted by a clearing member banking organization to a QCCP, and which could be considered a receivable due from the QCCP under generally accepted accounting principles, would not be risk-weighted as a corporate exposure. Instead, for a client-cleared trade the cash collateral posted to a QCCP would receive a risk weight of 2 percent, if the cash associated with the trade meets the requirements under § _.35(b)(i)(3)(A) or § _.133(b)(i)(3)(A) of the capital rule, or 4 percent, if the collateral does not meet the requirements necessary to receive the 2 percent risk weight. For a trade made on behalf of the clearing member's own account, the cash collateral posted to a QCCP would receive a 2 percent risk weight. This amendment is intended to maintain incentives for banking organizations to post cash collateral and recognize that a receivable from a QCCP that arises in the context of a trade exposure should not be treated as equivalent to a receivable that would arise if, for example, a banking organization made a loan to a CCP.

B. Treatment of Client Financial Collateral Held by a CCP

Under § _.2 of the capital rule, financial collateral means, in part, collateral in which a banking organization has a perfected first-priority security interest in the collateral. However, when a banking organization is acting as a clearing member, it generally is required to post any client collateral to the CCP, in which case the CCP establishes and maintains a perfected first-priority security interest in the collateral instead of the clearing member. As a result, the capital rule does not permit a clearing member banking organization to recognize client collateral posted to a CCP as financial collateral.

Client collateral posted to a CCP remains available to support the credit risk of a derivative contract in the event of a client default. Specifically, where a client defaults the CCP will use the client collateral to offset its exposure to the client, and the clearing member would be required to cover only the amount of any deficiency between the liquidation value of the collateral and the exposure to the CCP. However, were the clearing member banking organization to enter into the derivative contract directly with the client, the clearing member would establish and maintain a perfected first-priority security interest in the collateral, and the exposure of the clearing member to the client would similarly be mitigated only to the extent the collateral is sufficient to cover the exposure amount of the transaction at the time of default. Therefore, the agencies are proposing to revise the definition of financial collateral to allow clearing member banking organizations to recognize as financial collateral noncash client collateral posted to a CCP. In this situation, the clearing member banking organization would not be required to establish and retain a first-priority security interest in the collateral for it to qualify as financial collateral under § _.2 of the capital rule.

C. Clearing Member Exposure When CCP Performance Is Not Guaranteed

The agencies are proposing to revise § _.35(c)(3) of the capital rule to align the capital requirements under the standardized approach for client-cleared transactions with the treatment under § _.133(c)(3) of the advanced approaches. Specifically, the proposal would allow a clearing member that does not guarantee the performance of the CCP to the clearing member's client to apply a zero percent risk weight to the CCP-facing portion of the transaction. The agencies already have implemented this treatment for purposes of the advanced approaches.^[60]

D. Bankruptcy Remoteness of Collateral

The agencies are proposing to remove the requirement in § _.35(b)(4)(i) of the standardized approach and § _.133(b)(4)(i) of the advanced approaches that collateral posted by a clearing member client banking organization to a clearing member must be bankruptcy-remote from a custodian in order for the client banking organization to avoid the application of risk-based capital requirements to the collateral, and clarify that a custodian must be acting in its capacity as a custodian for this treatment to apply.^[61] The agencies believe this revision is appropriate because the collateral would generally be considered to be bankruptcy-remote if the custodian is acting in its capacity as a custodian with respect to the collateral. Therefore, this revision would apply only in cases where the collateral is deposited with a third-party custodian, not in cases where a clearing member offers “self-custody” arrangements with its clients. In addition, this revision would make the collateral requirement for a clearing member client banking organization consistent with the treatment of collateral posted by a clearing member banking organization, which does not require that the posted collateral be bankruptcy-remote from the custodian, but would require in each case that the custodian be acting in its capacity as a custodian.

E. Adjusted Collateral Haircuts for Derivative Contracts

If a clearing member banking organization is acting as an agent between a client and a CCP and receives collateral from the client, the clearing member must determine the exposure amount for the client-facing portion of the derivative contract using the collateralized transactions framework under § _.37 of the capital rule or the counterparty credit risk framework under § _.132 of the capital rule. The clearing member banking organization may recognize the credit risk-mitigation benefits of the collateral posted by the client; however, under §§ _.37(c) and _.132(b) of the capital rule, the value of the collateral must be discounted by the application of a standard supervisory haircut to reflect any market price volatility in the value of the collateral over a 10-day holding period. For a repo-style transaction, the capital rule applies a scaling factor of 0.71 to the standard supervisory haircuts to reflect the limited risk to collateral in those transactions and effectively reduce the holding period to 5 days. The agencies believe a similar reduction in the haircuts should be provided for cleared derivative contracts, as they typically have a holding period of less than 10 days. Therefore, the agencies are proposing to revise §§ _.37 and _.132 of the capital rule to add an exception to the 10-day holding period for cleared derivative contracts and apply a scaling factor of 0.71 to the standard supervisory haircuts to reflect a 5-day holding period.

F. OCC Revisions to Lending Limits

The OCC proposes to revise its lending limit rule at 12 CFR part 32. The current lending limits rule references sections of CEM in the OCC's advanced approaches capital rule as one available methodology for calculating exposures to derivatives transactions. However, these sections are proposed to be amended or replaced with SA-CCR in the advanced approaches. Therefore, the OCC is proposing to replace the references to CEM in the advanced approaches with references to CEM in the standardized approach. The OCC is also proposing to adopt SA-CCR as an option for calculation of exposures under lending limits.

Question 18: Should the OCC permit or require banking organizations to calculate exposures for derivatives transactions for lending limits purposes using SA-CCR? What advantages or disadvantages does this offer compared with the current methods allowed for calculating derivatives exposures for lending limits purposes?

VI. Impact of the Proposed Rule

To assess the effect of the proposed changes to the capital rule, the agencies reviewed data provided by advanced approaches banking organizations that represent a significant majority of the derivatives market. In particular, the agencies analyzed the change in exposure amount between CEM and SA-CCR, as well as the change in risk-weighted assets as determined under the standardized approach.^[62] The data covers diverse portfolios of derivative contracts, both in terms of asset type and counterparty. In addition, the data includes firms that serve as clearing members, allowing the agencies to consider the effect of the proposal under the cleared transactions framework for both a direct exposure to a CCP and an exposure to a CCP on behalf of a client. As a result, the analysis provides a reasonable proxy for the potential changes for all advanced approaches banking organizations.

As noted above, SA-CCR would improve risk-sensitivity when measuring the exposure amount for derivative contracts compared to CEM, including through improved collateral recognition. For instance, the exposure amount of margined derivative contracts for these firms would decrease by approximately 44 percent, while the exposure amount of unmargined derivative contracts for these firms would increase by approximately 90 percent. Overall, the agencies estimate that, under the proposal, the exposure amount for derivative contracts held by advanced approaches banking organizations would decrease by approximately 7 percent.

The agencies also analyzed the changes based on both asset classes and counterparties for these firms. With respect to asset classes, the exposure amount would increase for interest rate derivative contracts, equity derivative contracts, and commodity derivative contracts, while the exposure amount would decrease for exchange rate derivative contracts and credit derivative contracts. These changes are largely due to the updated supervisory factors, which reflect stress volatilities observed during the financial crisis. With respect to counterparties, the exposure amount would decrease for derivative contracts with banks, broker-dealers, and CCPs, which are typically margined, hedged, and subject to QMNAs. In contrast, exposure amounts would increase for derivative contracts with other financial institutions, such as asset managers, investment funds, and pension funds; sovereigns and municipalities; and commercial entities that use derivative contracts to hedge commercial risk.

The agencies estimate that the proposal would result in an approximately 5 percent increase in advanced approaches banking organizations' standardized risk-weighted assets associated with derivative contract exposures.^[63] This would result in a reduction (approximately 6 basis points) in advanced approaches banking organizations' tier 1 risk-based capital ratios, on average. This estimate assumes, consistent with the proposal, that a netting set is defined to include all derivative contracts subject to a QMNA.

The agencies estimate that the proposal would result in an increase (approximately 30 basis points) in advanced approaches banking organizations' supplementary leverage ratio, on average. However, this estimate does not reflect the broad definition of netting set in the proposal, which, if adopted, would likely result in an additional increase in advanced approaches banking organizations' supplementary leverage ratio. The proposal would use a modified version of SA-CCR that would recognize only certain cash variation margin in the replacement cost component calculation for purposes of the supplementary leverage ratio. Additional recognition of client collateral in the modified version of SA-CCR would further increase clearing member banking organizations' supplementary leverage ratio, but such an increase would largely depend on the degree of client clearing services provided by a clearing member banking organization.

The effects of the proposed rule likely would be limited for non-advanced approaches banking organizations. First, these banking organizations hold relatively small derivative portfolios. Non-advanced approaches banking organizations account for less than 8 percent of derivative contracts of all banking organizations, even though they account for 40 percent of total assets of all banking organizations.^[64] Second, non-advanced approaches banking organization are not subject to supplementary leverage ratio requirements, and thus would not be affected by any changes to the calculation of total leverage exposure. Finally, these banking organizations retain the option of using CEM, and the agencies anticipate that only those banking organizations that receive a net benefit from using SA-CCR would elect to use it.

VII. Regulatory Analyses

A. Paperwork Reduction Act

Certain provisions of the proposed rule contain “collection of information” requirements within the meaning of the Paperwork Reduction Act (PRA) of 1995 (44 U.S.C. 3501-3521). In accordance with the requirements of the PRA, the agencies may not conduct or sponsor, and the respondent is not required to respond to, an information collection unless it displays a currently-valid Office of Management and Budget (OMB) control number. The OMB control number for the OCC is 1557-0318, Board is 7100-0313, and FDIC is 3064-0153. These information collections will be extended for three years, with revision. The information collection requirements contained in this proposed rulemaking have been submitted by the OCC and FDIC to OMB for review and approval under section 3507(d) of the PRA (44 U.S.C. 3507(d)) and § 1320.11 of the OMB's implementing regulations (5 CFR part 1320). The Board reviewed the proposed rule under the authority delegated to the Board by OMB.

Comments are invited on:

a. Whether the collections of information are necessary for the proper performance of the Board's functions, including whether the information has practical utility;

b. The accuracy or the estimate of the burden of the information collections, including the validity of the methodology and assumptions used;

c. Ways to enhance the quality, utility, and clarity of the information to be collected;

d. Ways to minimize the burden of the information collections on respondents, including through the use of automated collection techniques or other forms of information technology; and

e. Estimates of capital or startup costs and costs of operation, maintenance, and purchase of services to provide information.

All comments will become a matter of public record. Comments on aspects of this notice that may affect reporting, recordkeeping, or disclosure requirements and burden estimates should be sent to the addresses listed in the ADDRESSES section of this document. A copy of the comments may also be submitted to the OMB desk officer by mail to U.S. Office of Management and Budget, 725 17th Street NW, #10235, Washington, DC 20503; facsimile to [phone]; or email to [e-mail], Attention, Federal Banking Agency Desk Officer.

Proposed Information Collection

Title of Information Collection: Recordkeeping and Disclosure Requirements Associated With Capital Adequacy.

Frequency: Quarterly, annual.

Affected Public: Businesses or other for-profit.

Respondents:

OCC: National banks and federal savings associations.

Board: State member banks (SMBs), bank holding companies (BHCs), U.S. intermediate holding companies (IHCs), savings and loan holding companies (SLHCs), and global systemically important bank holding companies (GSIBs) domiciled in the United States.

FDIC: State nonmember banks, state savings associations, and certain subsidiaries of those entities.

Current Actions: The proposal would revise §§ _.2, _.10, _.32, _.34 (including Table 1), _.35, _.132 (including Table 2), and _.133 of the capital rule to implement SA-CCR in order to calculate the exposure amount of derivatives contracts under the agencies' regulatory capital rule as well as update other parts of the capital rule to account for the proposed incorporation of SA-CCR.

The proposal will not, however, result in changes to the burden. In order to be consistent across the agencies, the agencies are applying a conforming methodology for calculating the burden estimates. The agencies are also updating the number of respondents based on the current number of supervised entities even though this proposal only affects a limited number of entities. The agencies believe that any changes to the information collections associated with the proposed rule are the result of the conforming methodology and updates to the respondent count, and not the result of the proposed rule changes.

PRA Burden Estimates

OCC

OMB control number: 1557-0318.

Estimated number of respondents: 1,365 (of which 18 are advanced approaches institutions).

Estimated average hours per response:

Minimum Capital Ratios (1,365 institutions affected for ongoing)

Recordkeeping (Ongoing)—16.

Standardized Approach (1,365 institutions affected for ongoing)

Recordkeeping (Initial setup)—122.

Recordkeeping (Ongoing)—20.

Disclosure (Initial setup)—226.25.

Disclosure (Ongoing quarterly)—131.25.

Advanced Approach (18 institutions affected for ongoing)

Recordkeeping (Initial setup)—460.

Recordkeeping (Ongoing)—540.77.

Recordkeeping (Ongoing quarterly)—20.

Disclosure (Initial setup)—280.

Disclosure (Ongoing)—5.78.

Disclosure (Ongoing quarterly)—35.

Estimated annual burden hours: 1,088 hours initial setup, 64,929 for ongoing.

Board

Agency form number: FR Q.

OMB control number: 7100-0313.

Estimated number of respondents: 1,431 (of which 17 are advanced approaches institutions).

Estimated average hours per response:

Minimum Capital Ratios (1,431 institutions affected for ongoing)

Recordkeeping (Ongoing)—16.

Standardized Approach (1,431 institutions affected for ongoing)

Recordkeeping (Initial setup)—122.

Recordkeeping (Ongoing)—20.

Disclosure (Initial setup)—226.25.

Disclosure (Ongoing quarterly)—131.25.

Advanced Approach (17 institutions affected)

Recordkeeping (Initial setup)—460.

Recordkeeping (Ongoing)—540.77.

Recordkeeping (Ongoing quarterly)—20.

Disclosure (Initial setup)—280.

Disclosure (Ongoing)—5.78.

Disclosure (Ongoing quarterly)—35.

Disclosure (Table 13 quarterly)—5.

Risk-based Capital Surcharge for GSIBs (21 institutions affected)

Recordkeeping (Ongoing)—0.5.

Estimated annual burden hours: 1,088 hours initial setup, 78,183 hours for ongoing.

FDIC

OMB control number: 3064-0153.

Estimated number of respondents: 3,604 (of which 2 are advanced approaches institutions).

Estimated average hours per response:

Minimum Capital Ratios (3,604 institutions affected)

Recordkeeping (Ongoing)—16.

Standardized Approach (3,604 institutions affected)

Recordkeeping (Initial setup)—122.

Recordkeeping (Ongoing)—20.

Disclosure (Initial setup)—226.25.

Disclosure (Ongoing quarterly)—131.25.

Advanced Approach (2 institutions affected)

Recordkeeping (Initial setup)—460.

Recordkeeping (Ongoing)—540.77.

Recordkeeping (Ongoing quarterly)—20.

Disclosure (Initial setup)—280.

Disclosure (Ongoing)—5.78.

Disclosure (Ongoing quarterly)—35.

Estimated annual burden hours: 1,088 hours initial setup, 131,802 hours for ongoing.

Also as a result of this proposed rule, the agencies would clarify the reporting instructions for the Consolidated Reports of Condition and Income (Call Reports) (FFIEC 031, FFIEC 041, and FFIEC 051) and Regulatory Capital Reporting for Institutions Subject to the Advanced Capital Adequacy Framework (FFIEC 101). The OCC and FDIC would clarify the reporting instructions for DFAST 14A, and the Board would clarify the reporting instructions for the Consolidated Financial Statements for Holding Companies (FR Y-9C), Capital Assessments and Stress Testing (FR Y-14A and FR Y-14Q), and Banking Organization Systemic Risk Report (FR Y-15) to reflect the changes to the capital rules that would be required under this proposal. The OCC also is proposing to update cross-references in its lending limit rules to account for the proposed incorporation of SA-CCR.

B. Regulatory Flexibility Act

OCC: The Regulatory Flexibility Act, 5 U.S.C. 601 et seq., (RFA), requires an agency, in connection with a proposed rule, to prepare an Initial Regulatory Flexibility Analysis describing the impact of the rule on small entities (defined by the Small Business Administration (SBA) for purposes of the RFA to include commercial banks and savings institutions with total assets of $550 million or less and trust companies with total revenue of $38.5 million or less) or to certify that the proposed rule would not have a significant economic impact on a substantial number of small entities. As of December 31, 2017, the OCC supervised 886 small entities. The rule would impose requirements on all OCC supervised entities that are subject to the advanced approaches risk-based capital rules, which typically have assets in excess of $250 billion, and therefore would not be small entities. While small entities would have the option to adopt SA-CCR, the OCC does not expect any small entities to elect that option. Therefore, the OCC estimates the proposed rule would not generate any costs for small entities. Therefore, the OCC certifies that the proposed rule would not have a significant economic impact on a substantial number of OCC-supervised small entities.

FDIC: The Regulatory Flexibility Act (RFA), 5 U.S.C. 601 et seq., generally requires an agency, in connection with a proposed rule, to prepare and make available for public comment an initial regulatory flexibility analysis that describes the impact of a proposed rule on small entities.^[65] However, a regulatory flexibility analysis is not required if the agency certifies that the rule will not have a significant economic impact on a substantial number of small entities. The Small Business Administration (SBA) has defined “small entities” to include banking organizations with total assets of less than or equal to $550 million.^[66]

As of March 31, 2018, there were 3,604 FDIC-supervised institutions, of which 2,804 are considered small entities for the purposes of RFA. These small entities hold $505 billion in assets, accounting for 17 percent of total assets held by FDIC-supervised institutions.^[67]

The proposed rule would require advanced approaches institutions to replace CEM with SA-CCR as an option for calculating EAD. There are no FDIC-supervised advanced approaches institutions that are considered small entities for the purposes of RFA.

In addition, the proposed rule would allow non-advanced approaches institutions to replace CEM with SA-CCR as the approach for calculating EAD. This allowance applies to all 2,804 small institutions supervised by the FDIC. Institutions that elect to use SA-CCR would incur some costs related to other compliance requirements of the proposed rule. However, these costs are difficult to estimate given that adoption of SA-CCR is voluntary. The FDIC expects that non-advanced approaches institutions will elect to use SA-CCR only if the net benefits of doing so are positive. Thus, the FDIC expects the proposed rule will not impose any net economic costs on these entities.

According to recent data, 395 (14.1 percent) small FDIC-supervised institutions, reporting $107 billion in assets, report holding some volume of derivatives and would thus have the option of electing to use SA-CCR. However, these institutions report holding only $5.4 billion (or 5 percent of assets) in derivatives.^[68] Therefore, the potential effects of electing SA-CCR are likely to be insignificant for these institutions.

Based on the information above, the FDIC certifies that the proposed rule will not have a significant economic impact on a substantial number of small entities.

The FDIC invites comments on all aspects of the supporting information provided in this RFA section. In particular, would this rule have any significant effects on small entities that the FDIC has not identified?

Board: The Board is providing an initial regulatory flexibility analysis with respect to this proposed rule. The Regulatory Flexibility Act, 5 U.S.C. 601 et seq., (RFA), requires an agency to consider whether the rules it proposes will have a significant economic impact on a substantial number of small entities.^[69] In connection with a proposed rule, the RFA requires an agency to prepare an Initial Regulatory Flexibility Analysis describing the impact of the rule on small entities or to certify that the proposed rule would not have a significant economic impact on a substantial number of small entities. An initial regulatory flexibility analysis must contain (1) a description of the reasons why action by the agency is being considered; (2) a succinct statement of the objectives of, and legal basis for, the proposed rule; (3) a description of, and, where feasible, an estimate of the number of small entities to which the proposed rule will apply; (4) a description of the projected reporting, recordkeeping, and other compliance requirements of the proposed rule, including an estimate of the classes of small entities that will be subject to the requirement and the type of professional skills necessary for preparation of the report or record; (5) an identification, to the extent practicable, of all relevant Federal rules which may duplicate, overlap with, or conflict with the proposed rule; and (6) a description of any significant alternatives to the proposed rule which accomplish its stated objectives.

The Board has considered the potential impact of the proposed rule on small entities in accordance with the RFA. Based on its analysis and for the reasons stated below, the Board believes that this proposed rule will not have a significant economic impact on a substantial number of small entities. Nevertheless, the Board is publishing and inviting comment on this initial regulatory flexibility analysis. A final regulatory flexibility analysis will be conducted after comments received during the public comment period have been considered. The proposal would also make corresponding changes to the Board's reporting forms.

As discussed in detail above, the proposed rule would amend the capital rule to provide a new methodology for calculating the exposure amount for derivative contracts. For purposes of calculating advanced approaches total risk-weighted assets, an advanced approaches Board-regulated institution would be able to use either SA-CCR or the internal models methodology. For purposes of calculating standardized approach total risk-weighted assets, an advanced approaches Board-regulated institution would be required to use SA-CCR and a non-advanced approaches Board-regulated institution would be able to elect either SA-CCR or the existing methodology. In addition, for purposes of the denominator of the supplementary leverage ratio, the proposal would integrate SA-CCR into the calculation of the denominator, replacing CEM.

The Board has broad authority under the International Lending Supervision Act (ILSA) ^[70] and the PCA provisions of the Federal Deposit Insurance Act ^[71] to establish regulatory capital requirements for the institutions it regulates. For example, ILSA directs each Federal banking agency to cause banking institutions to achieve and maintain adequate capital by establishing minimum capital requirements as well as by other means that the agency deems appropriate.^[72] The PCA provisions of the Federal Deposit Insurance Act direct each Federal banking agency to specify, for each relevant capital measure, the level at which an IDI subsidiary is well capitalized, adequately capitalized, undercapitalized, and significantly undercapitalized.^[73] In addition, the Board has broad authority to establish regulatory capital standards for bank holding companies, savings and loan holding companies, and U.S. intermediate holding companies of foreign banking organizations under the Bank Holding Company Act, the Home Owners' Loan Act, and the Dodd-Frank Reform and Consumer Protection Act (Dodd-Frank Act).^[74]

The proposed rule would only impose mandatory changes on advanced approaches banking organizations. Advanced approaches banking organizations include depository institutions, bank holding companies, savings and loan holding companies, or intermediate holding companies with at least $250 billion in total consolidated assets or has consolidated on-balance sheet foreign exposures of at least $10 billion, or a subsidiary of a depository institution, bank holding company, savings and loan holding company, or intermediate holding company that is an advanced approaches banking organization. The proposed rule therefore would not impose mandatory requirements on any small entities. However, the proposal would allow Board-regulated institutions that are not advanced approaches Board-regulated institutions to elect to use SA-CCR instead of CEM. Small entities that are subject to the Board's capital rule could make such an election, which would require immediate changes to reporting, recordkeeping, and compliance systems, as well as the ongoing burden of maintaining these different systems. However, the entities that elect to use SA-CCR may face reduced regulatory capital requirements as a result.

Further, as discussed previously in the Paperwork Reduction Act section, the proposal would make changes to the projected reporting, recordkeeping, and other compliance requirements of the rule by proposing to collect information from advanced approaches Board-regulated institutions and non-advanced approaches Board-regulated institutions that elect to use SA-CCR. These changes would include limited revisions to the Call Report (FFIEC 031, 041, and 051), the Consolidated Financial Statements for Holding Companies (FR Y-9C), and the Regulatory Capital Reporting for Institutions Subject to the Advanced Capital Adequacy Framework (FFIEC 101) to provide for reporting of derivative contracts under SA-CCR. Firms would be required to update their systems to implement these changes to reporting forms. The Board does not expect that the compliance, recordkeeping, and reporting updates described previously would impose a significant cost on small Board-regulated institutions. These changes would only impact small entities that elect to use SA-CCR. In addition, the Board is aware of no other Federal rules that duplicate, overlap, or conflict with the proposed changes to the capital rule. Therefore, the Board believes that the proposed rule will not have a significant economic impact on small banking organizations supervised by the Board and therefore believes that there are no significant alternatives to the proposed rule that would reduce the economic impact on small banking organizations supervised by the Board.

The Board welcomes comment on all aspects of its analysis. In particular, the Board requests that commenters describe the nature of any impact on small entities and provide empirical data to illustrate and support the extent of the impact.

C. Plain Language

Section 722 of the Gramm-Leach-Bliley Act requires the Federal banking agencies to use plain language in all proposed and final rules published after January 1, 2000. The agencies have sought to present the proposed rule in a simple and straightforward manner, and invite comment on the use of plain language. For example:

• Have the agencies organized the material to suit your needs? If not, how could they present the rule more clearly?
• Are the requirements in the rule clearly stated? If not, how could the rule be more clearly stated?
• Do the regulations contain technical language or jargon that is not clear? If so, which language requires clarification?
• Would a different format (grouping and order of sections, use of headings, paragraphing) make the regulation easier to understand? If so, what changes would achieve that?
• Is this section format adequate? If not, which of the sections should be changed and how?
• What other changes can the agencies incorporate to make the regulation easier to understand?

D. Riegle Community Development and Regulatory Improvement Act of 1994

Pursuant to section 302(a) of the Riegle Community Development and Regulatory Improvement Act (RCDRIA),^[75] in determining the effective date and administrative compliance requirements for new regulations that impose additional reporting, disclosure, or other requirements on IDIs, each Federal banking agency must consider, consistent with principles of safety and soundness and the public interest, any administrative burdens that such regulations would place on depository institutions, including small depository institutions, and customers of depository institutions, as well as the benefits of such regulations. In addition, section 302(b) of RCDRIA requires new regulations and amendments to regulations that impose additional reporting, disclosures, or other new requirements on IDIs generally to take effect on the first day of a calendar quarter that begins on or after the date on which the regulations are published in final form.^[76]

Because the proposal [would/would not] impose additional reporting, disclosure, or other requirements on IDIs, section 302 of the RCDRIA therefore [does/does not] apply. Nevertheless, the requirements of RCDRIA will be considered as part of the overall rulemaking process. In addition, the agencies also invite any other comments that further will inform the agencies' consideration of RCDRIA.

E. OCC Unfunded Mandates Reform Act of 1995 Determination

The OCC analyzed the proposed rule under the factors set forth in the Unfunded Mandates Reform Act of 1995 (UMRA) (2 U.S.C. 1532). Under this analysis, the OCC considered whether the proposed rule includes a Federal mandate that may result in the expenditure by State, local, and Tribal governments, in the aggregate, or by the private sector, of $100 million or more in any one year (adjusted for inflation). The OCC has determined that this proposed rule would not result in expenditures by State, local, and Tribal governments, or the private sector, of $100 million or more in any one year. Accordingly, the OCC has not prepared a written statement to accompany this proposal.

List of Subjects

12 CFR Part 3

• Administrative practice and procedure
• Capital
• National banks
• Risk

12 CFR Part 32

• National banks
• Reporting and recordkeeping requirements

12 CFR Part 217

• Administrative practice and procedure
• Banks
• Banking
• Capital
• Federal Reserve System
• Holding companies

12 CFR Part 324

• Administrative practice and procedure
• Banks
• Banking
• Capital adequacy
• Savings associations
• State non-member banks

Office of the Comptroller of the Currency

For the reasons set out in the joint preamble, the OCC proposes to amend 12 CFR parts 3 and 32 as follows:

PART 3—CAPITAL ADEQUACY STANDARDS

1. The authority citation for part 3 continues to read as follows:

Authority: 12 U.S.C. 93a, 161, 1462, 1462a, 1463, 1464, 1818, 1828(n), 1828 note, 1831n note, 1835, 3907, 3909, and 5412(b)(2)(B).

2. Section 3.2 is amended by:

a. Adding the definitions of “Basis derivative contract” in alphabetical order;

b. Revising paragraph (2) of the definition of “Financial collateral;”

c. Adding the definitions of “Independent collateral,” “Minimum transfer amount,” and “Net independent collateral amount” in alphabetical order;

d. Revising the definition of “Netting set;” and

e. Adding the definitions of “Speculative grade,” “Sub-speculative grade,” “Variation margin,” “Variation margin agreement,” “Variation margin amount,” “Variation margin threshold,” and “Volatility derivative contract” in alphabetical order.

The additions and revisions read as follows:

§ 3.2

Definitions.

* * * * *

Basis derivative contract means a non-foreign-exchange derivative contract (i.e., the contract is denominated in a single currency) in which the cash flows of the derivative contract depend on the difference between two risk factors that are attributable solely to one of the following derivative asset classes: Interest rate, credit, equity, or commodity.

* * * * *

Financial collateral * * *

(2) In which the national bank and Federal savings association has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; and notwithstanding the prior security interest of any custodial agent or any priority security interest granted to a CCP in connection with collateral posted to that CCP).

* * * * *

Independent collateral means financial collateral, other than variation margin, that is subject to a collateral agreement, or in which a national bank and Federal savings association has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; notwithstanding the prior security interest of any custodial agent or any prior security interest granted to a CCP in connection with collateral posted to that CCP), and the amount of which does not change directly in response to the value of the derivative contract or contracts that the financial collateral secures.

* * * * *

Minimum transfer amount means the smallest amount of variation margin that may be transferred between counterparties to a netting set.

* * * * *

Net independent collateral amount means the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 3.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a national bank or Federal savings association less the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 3.132(b)(2)(ii), as applicable, posted by the national bank or Federal savings association to the counterparty, excluding such amounts held in a bankruptcy remote manner, or posted to a QCCP and held in conformance with the operational requirements in § 3.3.

Netting set means either one derivative contract between a national bank or Federal savings association and a single counterparty, or a group of derivative contracts between a national bank or Federal savings association and a single counterparty, that are subject to a qualifying master netting agreement.

* * * * *

Speculative grade means the reference entity has adequate capacity to meet financial commitments in the near term, but is vulnerable to adverse economic conditions, such that should economic conditions deteriorate, the reference entity would present an elevated default risk.

* * * * *

Sub-speculative grade means the reference entity depends on favorable economic conditions to meet its financial commitments, such that should such economic conditions deteriorate the reference entity likely would default on its financial commitments.

* * * * *

Variation margin means financial collateral that is subject to a collateral agreement provided by one party to its counterparty to meet the performance of the first party's obligations under one or more transactions between the parties as a result of a change in value of such obligations since the last time such financial collateral was provided.

Variation margin agreement means an agreement to collect or post variation margin.

Variation margin amount means the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 3.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a national bank or Federal savings association less the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 3.132(b)(2)(ii), as applicable, posted by the national bank or Federal savings association to the counterparty.

Variation margin threshold means the amount of credit exposure of a national bank or Federal savings association to its counterparty that, if exceeded, would require the counterparty to post variation margin to the national bank or Federal savings association.

Volatility derivative contract means a derivative contract in which the payoff of the derivative contract explicitly depends on a measure of the volatility of an underlying risk factor to the derivative contract.

* * * * *

3. Section 3.10 is amended by revising paragraphs (c)(4)(ii)(A) through (C) to read as follows:

§ 3.10

Minimum capital requirements.

* * * * *

(c) * * *

(4) * * *

(ii) * * *

(A) The balance sheet carrying value of all the national bank's or Federal savings association's on-balance sheet assets, plus the value of securities sold under a repurchase transaction or a securities lending transaction that qualifies for sales treatment under U.S. GAAP, less amounts deducted from tier 1 capital under § 3.22(a), (c), and (d), less the value of securities received in security-for-security repo-style transactions, where the national bank or Federal savings association acts as a securities lender and includes the securities received in its on-balance sheet assets but has not sold or re-hypothecated the securities received, and less the fair value of any derivative contracts;

(B) The PFE for each netting set (including cleared transactions except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the national bank or Federal savings association, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP), as determined under § 3.132(c)(7), in which the term C in § 3.132(c)(7)(i)(B) equals zero, multiplied by 1.4;

(C) The sum of:

(1)(i) 1.4 multiplied by the replacement cost of each derivative contract or single product netting set of derivative contracts to which the national bank or Federal savings association is a counterparty, calculated according to the following formula:

Replacement Cost = max{V−CVM_r + CVM_p; 0}

Where:

V equals the fair value for each derivative contract or each single-product netting set of derivative contracts (including a cleared transaction except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the national bank or Federal savings association, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP);

CVM_r equals the amount of cash collateral received from a counterparty to a derivative contract and that satisfies the conditions in paragraph (c)(4)(ii)(C)(3) through (7); and

CVM_p equals the amount of cash collateral that is posted to a counterparty to a derivative contract and that has not off-set the fair value of the derivative contract and that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section; and

(ii) Notwithstanding paragraph (c)(4)(ii)(C)(1)(i) of this section, where multiple netting sets are subject to a single variation margin agreement, a national bank or Federal savings association must apply the formula for replacement cost provided in § 3.132(c)(10), in which the term may only include cash collateral that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(2) The amount of cash collateral that is received from a counterparty to a derivative contract that has off-set the fair value of a derivative contract and that does not satisfy the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(3) For derivative contracts that are not cleared through a QCCP, the cash collateral received by the recipient counterparty is not segregated (by law, regulation or an agreement with the counterparty);

(4) Variation margin is calculated and transferred on a daily basis based on the fair value of the derivative contract;

(5) The variation margin transferred under the derivative contract or the governing rules for a cleared transaction is the full amount that is necessary to fully extinguish the net current credit exposure to the counterparty of the derivative contracts, subject to the threshold and minimum transfer amounts applicable to the counterparty under the terms of the derivative contract or the governing rules for a cleared transaction;

(6) The variation margin is in the form of cash in the same currency as the currency of settlement set forth in the derivative contract, provided that for the purposes of this paragraph (c)(4)(ii)(C)(6), currency of settlement means any currency for settlement specified in the governing qualifying master netting agreement and the credit support annex to the qualifying master netting agreement, or in the governing rules for a cleared transaction; and

(7) The derivative contract and the variation margin are governed by a qualifying master netting agreement between the legal entities that are the counterparties to the derivative contract or by the governing rules for a cleared transaction, and the qualifying master netting agreement or the governing rules for a cleared transaction must explicitly stipulate that the counterparties agree to settle any payment obligations on a net basis, taking into account any variation margin received or provided under the contract if a credit event involving either counterparty occurs;

* * * * *

4. Section 3.32 is amended by revising paragraph (f) to read as follows:

§ 3.32

General risk weights.

* * * * *

(f) Corporate exposures. (1) A national bank or Federal savings association must assign a 100 percent risk weight to all its corporate exposures, except as provided in paragraph (f)(2) of this section.

(2) A national bank or Federal savings association must assign a 2 percent risk weight to an exposure to a QCCP arising from the national bank or Federal savings association posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 3.35(b)(3)(i)(A) and a 4 percent risk weight to an exposure to a QCCP arising from the national bank or Federal savings association posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 3.35(b)(3)(i)(B).

(3) A national bank or Federal savings association must assign a 2 percent risk weight to an exposure to a QCCP arising from the national bank or Federal savings association posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 3.35(c)(3)(i).

* * * * *

5. Section 3.34 is revised to read as follows:

§ 3.34

Derivative contracts.

(a) Exposure amount for derivative contracts—(1) National bank or Federal savings association that is not an advanced approaches national bank or Federal savings association. (i) A national bank or Federal savings association that is not an advanced approaches national bank or Federal savings association must use the current exposure methodology (CEM) described in paragraph (b) of this section to calculate the exposure amount for all its OTC derivative contracts, unless the national bank or Federal savings association makes the election provided in paragraph (a)(1)(ii) of this section.

(ii) A national bank or Federal savings association that is not an advanced approaches national bank or Federal savings association may elect to calculate the exposure amount for all its OTC derivative contracts under the standardized approach for counterparty credit risk (SA-CCR) in § 3.132(c), rather than calculating the exposure amount for all its derivative contracts using the CEM. A national bank or Federal savings association that elects under this paragraph (a)(1)(ii) to calculate the exposure amount for its OTC derivative contracts under the SA-CCR must apply the treatment of cleared transactions under § 3.133 to its derivative contracts that are cleared transactions, rather than applying § 3.35. A national bank or Federal savings association that is not an advanced approaches national bank or Federal savings association must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the OCC.

(2) Advanced approaches national bank or Federal savings association. An advanced approaches national bank or Federal savings association must calculate the exposure amount for all its derivative contracts using the SA-CCR in § 3.132(c). An advanced approaches national bank or Federal savings association must apply the treatment of cleared transactions under § 3.133 to its derivative contracts that are cleared transactions.

(b) Current exposure methodology exposure amount—(1) Single OTC derivative contract. Except as modified by paragraph (c) of this section, the exposure amount for a single OTC derivative contract that is not subject to a qualifying master netting agreement is equal to the sum of the national bank's or Federal savings association's current credit exposure and potential future credit exposure (PFE) on the OTC derivative contract.

(i) Current credit exposure. The current credit exposure for a single OTC derivative contract is the greater of the fair value of the OTC derivative contract or zero.

(ii) PFE. (A) The PFE for a single OTC derivative contract, including an OTC derivative contract with a negative fair value, is calculated by multiplying the notional principal amount of the OTC derivative contract by the appropriate conversion factor in Table 1 to this section.

(B) For purposes of calculating either the PFE under this paragraph (b) or the gross PFE under paragraph (b)(2) of this section for exchange rate contracts and other similar contracts in which the notional principal amount is equivalent to the cash flows, notional principal amount is the net receipts to each party falling due on each value date in each currency.

(C) For an OTC derivative contract that does not fall within one of the specified categories in Table 1 to this section, the PFE must be calculated using the appropriate “other” conversion factor.

(D) A national bank or Federal savings association must use an OTC derivative contract's effective notional principal amount (that is, the apparent or stated notional principal amount multiplied by any multiplier in the OTC derivative contract) rather than the apparent or stated notional principal amount in calculating PFE.

(E) The PFE of the protection provider of a credit derivative is capped at the net present value of the amount of unpaid premiums.

Table 1 to § 3.34—Conversion Factor Matrix for Derivative Contracts ¹

Remaining maturity 2	Interest rate	Foreign exchange rate and gold	Credit (investment grade reference asset) 3	Credit (non- investment- grade reference asset)	Equity	Precious metals (except gold)	Other
One year or less	0.00	0.01	0.05	0.10	0.06	0.07	0.10
Greater than one year and less than or equal to five years	0.005	0.05	0.05	0.10	0.08	0.07	0.12
Greater than five years	0.015	0.075	0.05	0.10	0.10	0.08	0.15
1 For a derivative contract with multiple exchanges of principal, the conversion factor is multiplied by the number of remaining payments in the derivative contract.
2 For an OTC derivative contract that is structured such that on specified dates any outstanding exposure is settled and the terms are reset so that the fair value of the contract is zero, the remaining maturity equals the time until the next reset date. For an interest rate derivative contract with a remaining maturity of greater than one year that meets these criteria, the minimum conversion factor is 0.005.
3 A national bank or Federal savings association must use the column labeled “Credit (investment-grade reference asset)” for a credit derivative whose reference asset is an outstanding unsecured long-term debt security without credit enhancement that is investment grade. A national bank or Federal savings association must use the column labeled “Credit (non-investment-grade reference asset)” for all other credit derivatives.

(2) Multiple OTC derivative contracts subject to a qualifying master netting agreement. Except as modified by paragraph (c) of this section, the exposure amount for multiple OTC derivative contracts subject to a qualifying master netting agreement is equal to the sum of the net current credit exposure and the adjusted sum of the PFE amounts for all OTC derivative contracts subject to the qualifying master netting agreement.

(i) Net current credit exposure. The net current credit exposure is the greater of the net sum of all positive and negative fair values of the individual OTC derivative contracts subject to the qualifying master netting agreement or zero.

(ii) Adjusted sum of the PFE amounts. The adjusted sum of the PFE amounts, Anet, is calculated as

Anet = (0.4 × Agross) + (0.6 × NGR × Agross),

Where:

(A) Agross = the gross PFE (that is, the sum of the PFE amounts as determined under paragraph (b)(1)(ii) of this section for each individual derivative contract subject to the qualifying master netting agreement); and

(B) Net-to-gross Ratio (NGR) = the ratio of the net current credit exposure to the gross current credit exposure. In calculating the NGR, the gross current credit exposure equals the sum of the positive current credit exposures (as determined under paragraph (b)(1)(i) of this section) of all individual derivative contracts subject to the qualifying master netting agreement.

(c) Recognition of credit risk mitigation of collateralized OTC derivative contracts. (1) A national bank or Federal savings association using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures an OTC derivative contract or multiple OTC derivative contracts subject to a qualifying master netting agreement (netting set) by using the simple approach in § 3.37(b).

(2) As an alternative to the simple approach, a national bank or Federal savings association using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures such a contract or netting set if the financial collateral is marked-to-fair value on a daily basis and subject to a daily margin maintenance requirement by applying a risk weight to the uncollateralized portion of the exposure, after adjusting the exposure amount calculated under paragraph (b)(1) or (2) of this section using the collateral haircut approach in § 3.37(c). The national bank or Federal savings association must substitute the exposure amount calculated under paragraph (b)(1) or (2) of this section for ΣE in the equation in § 3.37(c)(2).

(d) Counterparty credit risk for credit derivatives—(1) Protection purchasers. A national bank or Federal savings association that purchases a credit derivative that is recognized under § 3.36 as a credit risk mitigant for an exposure that is not a covered position under subpart F of this part is not required to compute a separate counterparty credit risk capital requirement under § 3.32 provided that the national bank or Federal savings association does so consistently for all such credit derivatives. The national bank or Federal savings association must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure to all relevant counterparties for risk-based capital purposes.

(2) Protection providers. (i) A national bank or Federal savings association that is the protection provider under a credit derivative must treat the credit derivative as an exposure to the underlying reference asset. The national bank or Federal savings association is not required to compute a counterparty credit risk capital requirement for the credit derivative under § 3.32, provided that this treatment is applied consistently for all such credit derivatives. The national bank or Federal savings association must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure.

(ii) The provisions of this paragraph (d)(2) apply to all relevant counterparties for risk-based capital purposes unless the national bank or Federal savings association is treating the credit derivative as a covered position under subpart F of this part, in which case the national bank or Federal savings association must compute a supplemental counterparty credit risk capital requirement under this section.

(e) Counterparty credit risk for equity derivatives. (1) A national bank or Federal savings association must treat an equity derivative contract as an equity exposure and compute a risk-weighted asset amount for the equity derivative contract under §§ 3.51 through 3.53 (unless the national bank or Federal savings association is treating the contract as a covered position under subpart F of this part).

(2) In addition, the national bank or Federal savings association must also calculate a risk-based capital requirement for the counterparty credit risk of an equity derivative contract under this section if the national bank or Federal savings association is treating the contract as a covered position under subpart F of this part.

(3) If the national bank or Federal savings association risk weights the contract under the Simple Risk-Weight Approach (SRWA) in § 3.52, the national bank or Federal savings association may choose not to hold risk-based capital against the counterparty credit risk of the equity derivative contract, as long as it does so for all such contracts. Where the equity derivative contracts are subject to a qualified master netting agreement, a national bank or Federal savings association using the SRWA must either include all or exclude all of the contracts from any measure used to determine counterparty credit risk exposure.

(f) Clearing member national bank's or Federal savings association's exposure amount. The exposure amount of a clearing member national bank or Federal savings association using the CEM under paragraph (b) of this section for an OTC derivative contract or netting set of OTC derivative contracts where the national bank or Federal savings association is either acting as a financial intermediary and enters into an offsetting transaction with a QCCP or where the national bank or Federal savings association provides a guarantee to the QCCP on the performance of the client equals the exposure amount calculated according to paragraph (b)(1) or (2) of this section multiplied by the scaling factor 0.71. If the national bank or Federal savings association determines that a longer period is appropriate, the national bank or Federal savings association must use a larger scaling factor to adjust for a longer holding period as follows:

Where H = the holding period greater than five days. Additionally, the OCC may require the national bank or Federal savings association to set a longer holding period if the OCC determines that a longer period is appropriate due to the nature, structure, or characteristics of the transaction or is commensurate with the risks associated with the transaction.

6. Section 3.35 is amended by adding paragraph (a)(3), revising paragraph (b)(4)(i), and adding paragraph (c)(3)(iii) to read as follows:

§ 3.35

Cleared transactions.

(a) * * *

(3) Alternate requirements. Notwithstanding any other provision of this section, an advanced approaches national bank or Federal savings association or a national bank or Federal savings association that is not an advanced approaches national bank or Federal savings association and that has elected to use SA-CCR under § 3.34(a)(1) must apply § 3.133 to its derivative contracts that are cleared transactions rather than this section.

(b) * * *

(4) * * *

(i) Notwithstanding any other requirements in this section, collateral posted by a clearing member client national bank or Federal savings association that is held by a custodian (in its capacity as custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(3) * * *

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member national bank or Federal savings association may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a CCP where the clearing member national bank or Federal savings association is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 3.3(a), and the clearing member national bank or Federal savings association is not obligated to reimburse the clearing member client in the event of the CCP default.

* * * * *

7. Section 3.37 is amended by revising paragraph (c)(3)(iii) to read as follows:

§ 3.37

Collateralized transactions.

* * * * *

(c) * * *

(3) * * *

(iii) For repo-style transactions and cleared transactions, a national bank or Federal savings association may multiply the standard supervisory haircuts provided in paragraphs (c)(3)(i) and (ii) of this section by the square root of 1/2 (which equals 0.707107).

* * * * *

§§ 3.134, 3.202, and 3.210

[Amended]

8. For each section listed in the following table, the footnote number listed in the “Old footnote number” column is redesignated as the footnote number listed in the “New footnote number” column as follows:

Section	Old footnote No.	New footnote No.
3.134(d)(3)	30	31
3.202, paragraph (1) introductory text of the definition of “Covered position”	31	32
3.202, paragraph (1)(i) of the definition of “Covered position”	32	33
3.210(e)(1)	33	34

9. Section 3.132 is amended by:

a. Revising paragraphs (b)(2)(ii)(A)( 3) through (5);

b. Adding paragraphs (b)(2)(ii)(A)( 6) and (7);

c. Revising paragraphs (c) heading and (c)(1) and (2) and (5) through (8);

d. Adding paragraphs (c)(9) through (12);

e. Removing “Table 3 to § 3.132” and adding in its place “Table 4 to this section” in paragraphs (e)(5)(i)(A) and (H); and

f. Redesignating Table 3 to § 3.132 as Table 4 to § 3.132.

The revisions and additions read as follows:

§ 3.132

Counterparty credit risk of repo-style transactions, eligible margin loans, and OTC derivative contracts.

* * * * *

(b) * * *

(2) * * *

(ii) * * *

(A) * * *

(3) For repo-style transactions and cleared transactions, a national bank or Federal savings association may multiply the supervisory haircuts provided in paragraphs (b)(2)(ii)(A)(1) and (2) of this section by the square root of 1/2 (which equals 0.707107).

(4) A national bank or Federal savings association must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days (for eligible margin loans) or five business days (for repo-style transactions), using the formula provide in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. If the number of trades in a netting set exceeds 5,000 at any time during a quarter, a national bank or Federal savings association must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days for the following quarter (except when a national bank or Federal savings association is calculating EAD for a cleared transaction under § 3.133). If a netting set contains one or more trades involving illiquid collateral, a national bank or Federal savings association must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days. If over the two previous quarters more than two margin disputes on a netting set have occurred that lasted more than the holding period, then the national bank or Federal savings association must adjust the supervisory haircuts upward for that netting set on the basis of a holding period that is at least two times the minimum holding period for that netting set.

(5)(i) A national bank or Federal savings association must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days for collateral associated derivative contracts that are not cleared transactions using the formula provided in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. For collateral associated with a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, a national bank or Federal savings association must use a holding period of twenty business days. If a netting set contains one or more trades involving illiquid collateral or a derivative contract that cannot be easily replaced, a national bank or Federal savings association must use a holding period of twenty business days.

(ii) Notwithstanding paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section, for collateral associated with a derivative contract that is subject to an outstanding dispute over variation margin, the holding period is twice the amount provide under paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section.

(6) A national bank or Federal savings association must adjust the standard supervisory haircuts upward, pursuant to the adjustments provided in paragraphs (b)(2)(ii)(A)(4) and (5) of this section, using the following formula:

Where:

TM equals a holding period of longer than 10 business days for eligible margin loans and derivative contracts or longer than 5 business days for repo-style transactions;

Hs equals the standard supervisory haircut; and

Ts equals 10 business days for eligible margin loans and derivative contracts or 5 business days for repo-style transactions.

(7) If the instrument a national bank or Federal savings association has lent, sold subject to repurchase, or posted as collateral does not meet the definition of financial collateral, the national bank or Federal savings association must use a 25.0 percent haircut for market price volatility (Hs).

* * * * *

(c) EAD for derivative contracts— (1) Options for determining EAD. A national bank or Federal savings association must determine the EAD for a derivative contract using the standardized approach for counterparty credit risk (SA-CCR) under paragraph (c)(5) of this section or using the internal models methodology described in paragraph (d) of this section. If a national bank or Federal savings association elects to use SA-CCR for one or more derivative contracts, the exposure amount determined under SA-CCR is the EAD for the derivative contract or derivatives contracts. A national bank or Federal savings association must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the OCC.

(2) Definitions. For purposes of this paragraph (c), the following definitions apply:

(i) Except as otherwise provided in paragraph (c) of this section, the end date means the last date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references another instrument, by the underlying instrument.

(ii) Except as otherwise provided in paragraph (c) of this section, the start date means the first date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references the value of another instrument, by underlying instrument.

(iii) Hedging set means:

(A) With respect interest rate derivative contracts, all such contracts within a netting set that reference the same reference currency;

(B) With respect to exchange rate derivative contracts, all such contracts within a netting set that reference the same currency pair;

(C) With respect to credit derivative contract, all such contracts within a netting set;

(D) With respect to equity derivative contracts, all such contracts within a netting set;

(E) With respect to a commodity derivative contract, all such contracts within a netting set that reference one of the following commodity classes: Energy, metal, agricultural, or other commodities;

(F) With respect to basis derivative contracts, all such contracts within a netting set that reference the same pair of risk factors and are denominated in the same currency; or

(G) With respect to volatility derivative contracts, all such contracts within a netting set that reference one of interest rate, exchange rate, credit, equity, or commodity risk factors, separated according to the requirements under paragraphs (c)(2)(iii)(A) through (E) of this section.

(H) If the risk of a derivative contract materially depends on more than one of interest rate, exchange rate, credit, equity, or commodity risk factors, the OCC may require a national bank or Federal savings association to include the derivative contract in each appropriate hedging set under paragraphs (c)(2)(iii)(A) through (E) of this section.

* * * * *

(5) Exposure amount. The exposure amount of a netting set, as calculated under paragraph (c) of this section, is equal to 1.4 multiplied by the sum of the replacement cost of the netting set, as calculated under paragraph (c)(6) of this section, and the potential future exposure of the netting set, as calculated under paragraph (c)(7) of this section, except that, notwithstanding the requirements of this paragraph (c)(5):

(i) The exposure amount of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty to the variation margin agreement is not required to post variation margin, is equal to the lesser of the exposure amount of the netting set and the exposure amount of the netting set calculated as if the netting set were not subject to a variation margin agreement; and

(ii) The exposure amount of a netting set that consists of only sold options in which the premiums have been fully paid and that are not subject to a variation margin agreement is zero.

(6) Replacement cost of a netting set—(i) Netting set subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty is not required to post variation margin, is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts;

(B) The sum of the variation margin threshold and the minimum transfer amount applicable to the derivative contracts within the netting set less the net independent collateral amount applicable to such derivative contracts; or

(C) Zero.

(ii) Netting sets not subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set that is not subject to a variation margin agreement under which the counterparty must post variation margin to the national bank or Federal savings association is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the net independent collateral amount and variation margin amount applicable to such derivative contracts; or

(B) Zero.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(i) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(i) of this section.

(7) Potential future exposure of a netting set. The potential future exposure of a netting set is the product of the PFE multiplier and the aggregated amount.

(i) PFE multiplier. The PFE multiplier is calculated according to the following formula:

Where:

V is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

C is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting set; and

A is the aggregated amount of the netting set.

(ii) Aggregated amount. The aggregated amount is the sum of all hedging set amounts, as calculated under paragraph (c)(8) of this section, within a netting set.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under § 3.10(c)(4)(ii)(B), the potential future exposure for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(ii) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under § 3.10(c)(4)(ii)(B), the potential future exposure for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(ii) of this section.

(8) Hedging set amount—(i) Interest rate derivative contracts. To calculate the hedging set amount of an interest rate derivative contract hedging set, a national bank or Federal savings association may use either of the formulas provided in paragraphs (c)(8)(i)(A) and (B) of this section:

(ii) Exchange rate derivative contracts. For an exchange rate derivative contract hedging set, the hedging set amount equals the absolute value of the sum of the adjusted derivative contract amounts, as calculated under paragraph (c)(9) of this section, within the hedging set.

(iii) Credit derivative contracts and equity derivative contracts. The hedging set amount of a credit derivative contract hedging set or equity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each reference entity within the hedging set.

K is the number of reference entities within the hedging set.

AddOn(Ref_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference reference entity k.

r_k equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(iv) Commodity derivative contracts. The hedging set amount of a commodity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each commodity type within the hedging set.

K is the number of commodity types within the hedging set.

AddOn(Type_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference reference commodity type k.

r equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(v) Basis derivative contracts and volatility derivative contracts. Notwithstanding paragraphs (c)(8)(i) through (iv) of this section, a national bank or Federal savings association must calculate a separate hedging set amount for each basis derivative contract hedging set and each volatility derivative contract hedging set. A national bank or Federal savings association must calculate such hedging set amounts using one of the formulas under paragraphs (c)(8)(i) through (iv) that corresponds to the primary risk factor of the hedging set being calculated.

(9) Adjusted derivative contract amount—(i) Summary. To calculate the adjusted derivative contract amount of a derivative contract, a national bank or Federal savings association must determine the adjusted notional amount of derivative contract, pursuant to paragraph (c)(9)(ii) of this section, and multiply the adjusted notional amount by each of the supervisory delta adjustment, pursuant to paragraph (c)(9)(iii) of this section, the maturity factor, pursuant to paragraph (c)(9)(iv) of this section, and the applicable supervisory factor, as provided in Table 2 to this section.

(ii) Adjusted notional amount. (A)(1) For an interest rate derivative contract or a credit derivative contract, the adjusted notional amount equals the product of the notional amount of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation, and the supervisory duration, as calculated by the following formula:

Where:

S is the number of business days from the present day until the start date of the derivative contract, or zero if the start date has already passed; and

E is the number of business days from the present day until the end date of the derivative contract.

(2) For purposes of paragraph (c)(9)(ii)(A)(1) of this section:

(i) For an interest rate derivative contract or credit derivative contract that is a variable notional swap, the notional amount is equal to the time-weighted average of the contractual notional amounts of such a swap over the remaining life of the swap; and

(ii) For an interest rate derivative contract or a credit derivative contract that is a leveraged swap, in which the notional amount of all legs of the derivative contract are divided by a factor and all rates of the derivative contract are multiplied by the same factor, the notional amount is equal to the notional amount of an equivalent unleveraged swap.

(B)(1) For an exchange rate derivative contract, the adjusted notional amount is the notional amount of the non-U.S. denominated currency leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation. If both legs of the exchange rate derivative contract are denominated in currencies other than U.S. dollars, the adjusted notional amount of the derivative contract is the largest leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation.

(2) Notwithstanding paragraph (c)(9)(ii)(B)(1) of this section, for an exchange rate derivative contract with multiple exchanges of principal, the national bank or Federal savings association must set the adjusted notional amount of the derivative contract equal to the notional amount of the derivative contract multiplied by the number of exchanges of principal under the derivative contract.

(C)(1) For an equity derivative contract or a commodity derivative contract, the adjusted notional amount is the product of the fair value of one unit of the reference instrument underlying the derivative contract and the number of such units referenced by the derivative contract.

(2) Notwithstanding paragraph (c)(9)(ii)(C)(1) of this section, when calculating the adjusted notional amount for an equity derivative contract or a commodity derivative contract that is a volatility derivative contract, the national bank or Federal savings association must replace the unit price with the underlying volatility referenced by the volatility derivative contract and replace the number of units with the notional amount of the volatility derivative contract.

(iii) Supervisory delta adjustments. (A) For a derivative contract that is not an option contract or collateralized debt obligation tranche, the supervisory delta adjustment is 1 if the fair value of the derivative contract increases when the value of the primary risk factor increases and −1 if the fair value of the derivative contract decreases when the value of the primary risk factor increases;

(B)(1) For a derivative contract that is an option contract, the supervisory delta adjustment is determined by the following formulas, as applicable:

(2) As used in the formulas in Table 3 to this section:

(i) Φ is the standard normal cumulative distribution function;

(ii) P equals the current fair value of the instrument or risk factor, as applicable, underlying the option;

(iii) K equals the strike price of the option;

(iv) T equals the number of business days until the latest contractual exercise date of the option;

(v) l equals zero for all derivative contracts except interest rate options for the currencies where interest rates have negative values. The same value of l must be used for all interest rate options that are denominated in the same currency. To determine the value of l for a given currency, a national bank or Federal savings association must find the lowest value L of P and K of all interest rate options in a given currency that the national bank or Federal savings association has with all counterparties. Then, l is set according to this formula: l = max{−L + 0.1%, 0}; and

(vi) σ equals the supervisory option volatility, as provided in Table 2 to of this section.

(C)(1) For a derivative contract that is a collateralized debt obligation tranche, the supervisory delta adjustment is determined by the following formula:

(2) As used in the formula in paragraph (c)(9)(iii)(C)(1) of this section:

(i) A is the attachment point, which equals the ratio of the notional amounts of all underlying exposures that are subordinated to the national bank's or Federal savings association's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; ^[30]

(ii) D is the detachment point, which equals one minus the ratio of the notional amounts of all underlying exposures that are senior to the national bank's or Federal savings association's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; and

(iii) The resulting amount is designated with a positive sign if the collateralized debt obligation tranche was purchased by the national bank or Federal savings association and is designated with a negative sign if the collateralized debt obligation tranche was sold by the national bank or Federal savings association.

(iv) Maturity factor. (A)(1) The maturity factor of a derivative contract that is subject to a variation margin agreement, excluding derivative contracts that are subject to a variation margin agreement under which the counterparty is not required to post variation margin, is determined by the following formula:

Where MPOR refers to the period from the most recent exchange of collateral covering a netting set of derivative contracts with a defaulting counterparty until the derivative contracts are closed out and the resulting market risk is re-hedged.

(2) Notwithstanding paragraph (c)(9)(iv)(A)(1) of this section:

(i) For a derivative contract that is not a cleared transaction, MPOR cannot be less than ten business days plus the periodicity of re-margining expressed in business days minus one business day;

(ii) For a derivative contract that is a cleared transaction, MPOR cannot be less than five business days plus the periodicity of re-margining expressed in business days minus one business day; and

(iii) For a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, MPOR cannot be less than twenty business days.

(3) Notwithstanding paragraphs (c)(9)(iv)(A)(1) and (2) of this section, for a derivative contract subject to an outstanding dispute over variation margin, the applicable floor is twice the amount provided in (c)(9)(iv)(A)(1) and (2) of this section.

(B) The maturity factor of a derivative contract that is not subject to a variation margin agreement, or derivative contracts under which the counterparty is not required to post variation margin, is determined by the following formula:

Where M equals the greater of 10 business days and the remaining maturity of the contract, as measured in business days.

(C) For purposes of paragraph (c)(9)(iv) of this section, derivative contracts with daily settlement are treated as derivative contracts not subject to a variation margin agreement and daily settlement does not change the end date of the period referenced by the derivative contract.

(v) Derivative contract as multiple effective derivative contracts. A national bank or Federal savings association must separate a derivative contract into separate derivative contracts, according to the following rules:

(A) For an option where the counterparty pays a predetermined amount if the value of the underlying asset is above or below the strike price and nothing otherwise (binary option), the option must be treated as two separate options. For purposes of paragraph (c)(9)(iii)(B) of this section, a binary option with strike K must be represented as the combination of one bought European option and one sold European option of the same type as the original option (put or call) with the strikes set equal to 0.95*K and 1.05*K so that the payoff of the binary option is reproduced exactly outside the region between the two strikes. The absolute value of the sum of the adjusted derivative contract amounts of the bought and sold options is capped at the payoff amount of the binary option.

(B) For a derivative contract that can be represented as a combination of standard option payoffs (such as collar, butterfly spread, calendar spread, straddle, and strangle), each standard option component must be treated as a separate derivative contract.

(C) For a derivative contract that includes multiple-payment options, (such as interest rate caps and floors) each payment option may be represented as a combination of effective single-payment options (such as interest rate caplets and floorlets).

(10) Multiple netting sets subject to a single variation margin agreement—(i) Calculating replacement cost. Notwithstanding paragraph (c)(6) of this section, a national bank or Federal savings association shall assign a single replacement cost to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin, calculated according to the following formula:

Replacement Cost = max{S_NSmax{V_NS; 0}−max{C_MA; 0}; 0} + max{S_NSmin{V_NS; 0}−min{C_MA; 0}; 0}

Where:

NS is each netting set subject to the variation margin agreement MA.

V_NS is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set NS.

C_MA is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting sets subject to the single variation margin agreement.

(ii) Calculating potential future exposure. Notwithstanding paragraph (c)(5) of this section, a national bank or Federal savings association shall assign a single potential future exposure to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin equal to the sum of the potential future exposure of each such netting set, each calculated according to paragraph (c)(7) of this section as if such nettings sets were not subject to a variation margin agreement.

(11) Netting set subject to multiple variation margin agreements or a hybrid netting set—(i) Calculating replacement cost. To calculate replacement cost for either a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, the calculation for replacement cost is provided under paragraph (c)(6)(ii) of this section, except that the variation margin threshold equals the sum of the variation margin thresholds of all variation margin agreements within the netting set and the minimum transfer amount equals the sum of the minimum transfer amounts of all the variation margin agreements within the netting set.

(ii) Calculating potential future exposure. (A) To calculate potential future exposure for a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty to the derivative contract must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, a national bank or Federal savings association must divide the netting set into sub-netting sets and calculate the aggregated amount for each sub-netting set. The aggregated amount for the netting set is calculated as the sum of the aggregated amounts for the sub-netting sets. The multiplier is calculated for the entire netting set.

(B) For purposes of paragraph (c)(11)(ii)(A) of this section, the netting set must be divided into sub-netting sets as follows:

(1) All derivative contracts within the netting set that are not subject to a variation margin agreement or that are subject to a variation margin agreement under which the counterparty is not required to post variation margin form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is not subject to a variation margin agreement.

(2) All derivative contracts within the netting set that are subject to variation margin agreements in which the counterparty must post variation margin and that share the same value of the MPOR form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is subject to a variation margin agreement, using the MPOR value shared by the derivative contracts within the netting set.

(12) Treatment of cleared transactions. (i) A national bank or Federal savings association must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more cleared transactions.

(ii) A national bank or Federal savings association that is a clearing member must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more exposures, each of which are exposures of the national bank or Federal savings association to its clearing member client where the national bank or Federal savings association is either acting as a financial intermediary and enters into an offsetting transaction with a CCP or where the national bank or Federal savings association provides a guarantee to the CCP on the performance of the client.

(iii)(A) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, MPOR may not be less than 10 business days;

(B) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, the minimum MPOR under paragraph (c)(9)(iv)(A)(3) of this section does not apply if there are no outstanding disputed trades in the netting set, there is no illiquid collateral in the netting set, and there are no exotic derivative contracts in the netting set; and

(C) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(A) and (B) of this section, if the CCP collects and holds variation margin and the variation margin is not bankruptcy remote from the CCP, M_i may not exceed 250 business days.

Table 2 to § 3.132—Supervisory Option Volatility, Supervisory Correlation Parameters, and Supervisory Factors for Derivative Contracts

Asset class	Subclass	Supervisory option volatility (%)	Supervisory correlation factor (%)	Supervisory factor 1 (%)
Interest rate	N/A	50	N/A	0.50
Exchange rate	N/A	15	N/A	4.0
Credit, single name	Investment grade	100	50	0.5
	Speculative grade	100	50	1.3
	Sub-speculative grade	100	50	6.0
Credit, index	Investment Grade	80	80	0.38
	Speculative Grade	80	80	1.06
Equity, single name	N/A	120	50	32
Equity, index	N/A	75	80	20
Commodity	Energy	150	40	40
	Metals	70	40	18
	Agricultural	70	40	18
	Other	70	40	18
1 The applicable supervisory factor for basis derivative contract hedging sets is equal to one-half of the supervisory factor provided in this Table 2, and the applicable supervisory factor for volatility derivative contract hedging sets is equal to 5 times the supervisory factor provided in this Table 2.

* * * * *

10. Section 3.133 is amended by revising paragraphs (a), (b) heading, (b)(1) through (3), (b)(4)(i), (c)(1) thorough (3), (c)(4)(i), and (d) to read as follows:

§ 3.133

Cleared transactions.

(a) General requirements—(1) Clearing member clients. A national bank or Federal savings association that is a clearing member client must use the methodologies described in paragraph (b) of this section to calculate risk-weighted assets for a cleared transaction.

(2) Clearing members. A national bank or Federal savings association that is a clearing member must use the methodologies described in paragraph (c) of this section to calculate its risk-weighted assets for a cleared transaction and paragraph (d) of this section to calculate its risk-weighted assets for its default fund contribution to a CCP.

(b) Clearing member client national bank or Federal savings association—(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a national bank or Federal savings association that is a clearing member client must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (b)(2) of this section, by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (b)(3) of this section.

(ii) A clearing member client national bank's or Federal savings association's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. (i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD for the derivative contract or netting set of derivative contracts calculated using the methodology used to calculate EAD for derivative contracts set forth in § 3.132(c) or (d), plus the fair value of the collateral posted by the clearing member client national bank or Federal savings association and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the national bank or Federal savings association calculates EAD for the cleared transaction using the methodology in § 3.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD for the repo-style transaction calculated using the methodology set forth in § 3.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member client national bank or Federal savings association and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the national bank or Federal savings association calculates EAD for the cleared transaction under § 3.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) For a cleared transaction with a QCCP, a clearing member client national bank or Federal savings association must apply a risk weight of:

(A) 2 percent if the collateral posted by the national bank or Federal savings association to the QCCP or clearing member is subject to an arrangement that prevents any loss to the clearing member client national bank or Federal savings association due to the joint default or a concurrent insolvency, liquidation, or receivership proceeding of the clearing member and any other clearing member clients of the clearing member; and the clearing member client national bank or Federal savings association has conducted sufficient legal review to conclude with a well-founded basis (and maintains sufficient written documentation of that legal review) that in the event of a legal challenge (including one resulting from an event of default or from liquidation, insolvency or receivership proceedings) the relevant court and administrative authorities would find the arrangements to be legal, valid, binding and enforceable under the law of the relevant jurisdictions.

(B) 4 percent, if the requirements of paragraph (b)(3)(i)(A) of this section are not met.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member client national bank or Federal savings association must apply the risk weight applicable to the CCP under § 3.32.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client national bank or Federal savings association that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a clearing member national bank or Federal savings association must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (c)(2) of this section by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (c)(3) of this section.

(ii) A clearing member national bank's or Federal savings association's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. A clearing member national bank or Federal savings association must calculate its trade exposure amount for a cleared transaction as follows:

(i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD calculated using the methodology used to calculate EAD for derivative contracts set forth in § 3.132(c) or (d), plus the fair value of the collateral posted by the clearing member national bank or Federal savings association and held by the CCP in a manner that is not bankruptcy remote. When the clearing member national bank or Federal savings association calculates EAD for the cleared transaction using the methodology in § 3.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD calculated under § 3.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member national bank or Federal savings association and held by the CCP in a manner that is not bankruptcy remote. When the clearing member national bank or Federal savings association calculates EAD for the cleared transaction under § 3.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) A clearing member national bank or Federal savings association must apply a risk weight of 2 percent to the trade exposure amount for a cleared transaction with a QCCP.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member national bank or Federal savings association must apply the risk weight applicable to the CCP according to § 3.32.

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member national bank or Federal savings association may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a QCCP where the clearing member national bank or Federal savings association is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 3.3(a), and the clearing member national bank or Federal savings association is not obligated to reimburse the clearing member client in the event of the QCCP default.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client national bank or Federal savings association that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(d) Default fund contributions—(1) General requirement. A clearing member national bank or Federal savings association must determine the risk-weighted asset amount for a default fund contribution to a CCP at least quarterly, or more frequently if, in the opinion of the national bank or Federal savings association or the OCC, there is a material change in the financial condition of the CCP.

(2) Risk-weighted asset amount for default fund contributions to nonqualifying CCPs. A clearing member national bank's or Federal savings association's risk-weighted asset amount for default fund contributions to CCPs that are not QCCPs equals the sum of such default fund contributions multiplied by 1,250 percent, or an amount determined by the OCC, based on factors such as size, structure and membership characteristics of the CCP and riskiness of its transactions, in cases where such default fund contributions may be unlimited.

(3) Risk-weighted asset amount for default fund contributions to QCCPs. A clearing member national bank's or Federal savings association's risk-weighted asset amount for default fund contributions to QCCPs equals the sum of its capital requirement, K_CM for each QCCP, as calculated under the methodology set forth in paragraph (e)(4) of this section.

(i) EAD must be calculated separately for each clearing member's sub-client accounts and sub-house account (i.e., for the clearing member's propriety activities). If the clearing member's collateral and its client's collateral are held in the same default fund contribution account, then the EAD of that account is the sum of the EAD for the client-related transactions within the account and the EAD of the house-related transactions within the account. For purposes of determining such EADs, the independent collateral of the clearing member and its client must be allocated in proportion to the respective total amount of independent collateral posted by the clearing member to the QCCP.

(ii) If any account or sub-account contains both derivative contracts and repo-style transactions, the EAD of that account is the sum of the EAD for the derivative contracts within the account and the EAD of the repo-style transactions within the account. If independent collateral is held for an account containing both derivative contracts and repo-style transactions, then such collateral must be allocated to the derivative contracts and repo-style transactions in proportion to the respective product specific exposure amounts, calculated, excluding the effects of collateral, according to § 3.132(b) for repo-style transactions and to § 3.132(c)(5) for derivative contracts.

(4) Risk-weighted asset amount for default fund contributions to a QCCP. A clearing member national bank's or Federal savings association's capital requirement for its default fund contribution to a QCCP (K_CM) is equal to:

(5) Hypothetical capital requirement of a QCCP. Where a QCCP has provided its K_CCP, a national bank or Federal savings association must rely on such disclosed figure instead of calculating K_CCP under this paragraph (d)(5), unless the national bank or Federal savings association determines that a more conservative figure is appropriate based on the nature, structure, or characteristics of the QCCP. The hypothetical capital requirement of a QCCP (K_CCP), as determined by the national bank or Federal savings association, is equal to:

K_CCP = Σ_CMi EAD_i * 1.6 percent

Where:

CM_i is each clearing member of the QCCP; and

EAD_i is the exposure amount of each clearing member of the QCCP to the QCCP, as determined under paragraph (d)(6) of this section.

(6) EAD of a clearing member national bank or Federal savings association to a QCCP. (i) The EAD of a clearing member national bank or Federal savings association to a QCCP is equal to the sum of the EAD for derivative contracts determined under paragraph (d)(6)(ii) of this section and the EAD for repo-style transactions determined under paragraph (d)(6)(iii) of this section.

(ii) With respect to any derivative contracts between the national bank or Federal savings association and the CCP that are cleared transactions and any guarantees that the national bank or Federal savings association has provided to the CCP with respect to performance of a clearing member client on a derivative contract, the EAD is equal to the sum of:

(A) The exposure amount for all such derivative contracts and guarantees of derivative contracts calculated under SA-CCR in § 3.132(c) using a value of 10 business days for purposes of § 3.132(c)(9)(iv)(B);

(B) The value of all collateral held by the CCP posted by the clearing member national bank or Federal savings association or a clearing member client of the national bank or Federal savings association in connection with a derivative contract for which the national bank or Federal savings association has provided a guarantee to the CCP; and

(C) The amount of the prefunded default fund contribution of the national bank or Federal savings association to the CCP.

(iii) With respect to any repo-style transactions between the national bank or Federal savings association and the CCP that are cleared transactions, EAD is equal to:

EAD = max{EBRM − IM − DF; 0}

Where:

EBRM is the sum of the exposure amounts of each repo-style transaction between the national bank or Federal savings association and the CCP as determined under § 3.132(b)(2) and without recognition of any collateral securing the repo-style transactions;

IM is the initial margin collateral posted by the national bank or Federal savings association to the CCP with respect to the repo-style transactions; and

DF is the prefunded default fund contribution of the national bank or Federal savings association to the CCP.

11. Section 3.300 is amended by adding paragraph (f) to read as follows:

§ 3.300

Transitions.

* * * * *

(f) SA-CCR. After giving prior notice to the OCC, an advanced approaches national bank or Federal savings association may use CEM rather than SA-CCR to determine the exposure amount for purposes of § 3.34 and the EAD for purposes of § 3.132 for its derivative contracts until July 1, 2020. On July 1, 2020, and thereafter, an advanced approaches national bank or Federal savings association must use SA-CCR for purposes of § 3.34 and must use either SA-CCR or IMM for purposes of § 3.132. Once an advanced approaches national bank or Federal savings association has begun to use SA-CCR, the advanced approaches national bank or Federal savings association may not change to use CEM.

PART 32—LENDING LIMITS

12. The authority citation for part 32 continues to read as follows:

Authority: 12 U.S.C. 1 et seq., 12 U.S.C. 84, 93a, 1462a, 1463, 1464(u), 5412(b)(2)(B), and 15 U.S.C. 1639h.

13. Section 32.9 is amended by revising paragraph (b)(1)(iii) and adding paragraph (b)(1)(iv) to read as follows:

§ 32.9

Credit exposure arising from derivative and securities financing transactions.

* * * * *

(b) * * *

(1) * * *

(iii) Current Exposure Method. The credit exposure arising from a derivative transaction (other than a credit derivative transaction) under the Current Exposure Method shall be calculated pursuant to 12 CFR 3.34(b)(1) and (2) and (c) or 324.34(b)(1) and (2) and (c), as appropriate.

(iv) Standardized Approach for Counterparty Credit Risk Method. The credit exposure arising from a derivative transaction (other than a credit derivative transaction) under the Standardized Approach for Counterparty Credit Risk Method shall be calculated pursuant to 12 CFR 3.132(c)(5) or 324.132(c)(5), as appropriate.

* * * * *

FEDERAL RESERVE SYSTEM

12 CFR Chapter II

Authority and Issuance

For the reasons set forth in the preamble, chapter II of title 12 of the Code of Federal Regulations is proposed to be amended as set forth below:

PART 217—CAPITAL ADEQUACY OF BANK HOLDING COMPANIES, SAVINGS AND LOAN HOLDING COMPANIES, AND STATE MEMBER BANKS (REGULATION Q)

14. The authority citation for part 217 continues to read as follows:

Authority: 12 U.S.C. 248(a), 321-338a, 481-486, 1462a, 1467a, 1818, 1828, 1831n, 1831o, 1831p-l, 1831w, 1835, 1844(b), 1851, 3904, 3906-3909, 4808, 5365, 5368, 5371.

15. Section 217.2 is amended by:

a. Adding the definition of “Basis derivative contract” in alphabetical order;

b. Revising paragraph (2) of the definition of “Financial collateral;”

c. Adding the definitions of “Independent collateral,” “Minimum transfer amount,” and “Net independent collateral amount” in alphabetical order;

d. Revising the definition of “Netting set;”

e. Adding the definitions of “Speculative grade,” “Sub-speculative grade,” “Variation margin,” “Variation margin agreement,” “Variation margin amount,” “Variation margin threshold,” and “Volatility derivative contract” in alphabetical order.

The additions and revisions read as follows:

§ 217.2

Definitions.

* * * * *

Basis derivative contract means a non-foreign-exchange derivative contract (i.e., the contract is denominated in a single currency) in which the cash flows of the derivative contract depend on the difference between two risk factors that are attributable solely to one of the following derivative asset classes: interest rate, credit, equity, or commodity.

* * * * *

Financial collateral * * *

(2) In which the Board-regulated institution has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof, (with the exception of cash on deposit; and notwithstanding the prior security interest of any custodial agent or any priority security interest granted to a CCP in connection with collateral posted to that CCP).

* * * * *

Independent collateral means financial collateral, other than variation margin, that is subject to a collateral agreement, or in which a Board-regulated institution has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; notwithstanding the prior security interest of any custodial agent or any prior security interest granted to a CCP in connection with collateral posted to that CCP), and the amount of which does not change directly in response to the value of the derivative contract or contracts that the financial collateral secures.

* * * * *

Minimum transfer amount means the smallest amount of variation margin that may be transferred between counterparties to a netting set.

* * * * *

Net independent collateral amount means the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 217.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a Board-regulated institution less the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 217.132(b)(2)(ii), as applicable, posted by the Board-regulated institution to the counterparty, excluding such amounts held in a bankruptcy remote manner, or posted to a QCCP and held in conformance with the operational requirements in § 217.3.

Netting set means either one derivative contract between a Board-regulated institution and a single counterparty, or a group of derivative contracts between a Board-regulated institution and a single counterparty, that are subject to a qualifying master netting agreement.

* * * * *

Speculative grade means the reference entity has adequate capacity to meet financial commitments in the near term, but is vulnerable to adverse economic conditions, such that should economic conditions deteriorate, the reference entity would present an elevated default risk.

* * * * *

Sub-speculative grade means the reference entity depends on favorable economic conditions to meet its financial commitments, such that should such economic conditions deteriorate the reference entity likely would default on its financial commitments.

* * * * *

Variation margin means financial collateral that is subject to a collateral agreement provided by one party to its counterparty to meet the performance of the first party's obligations under one or more transactions between the parties as a result of a change in value of such obligations since the last time such financial collateral was provided.

Variation margin agreement means an agreement to collect or post variation margin.

Variation margin amount means the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 217.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a Board-regulated institution less the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 217.132(b)(2)(ii), as applicable, posted by the Board-regulated institution to the counterparty.

Variation margin threshold means the amount of credit exposure of a Board-regulated institution to its counterparty that, if exceeded, would require the counterparty to post variation margin to the Board-regulated institution.

Volatility derivative contract means a derivative contract in which the payoff of the derivative contract explicitly depends on a measure of the volatility of an underlying risk factor to the derivative contract.

* * * * *

16. Section 217.10 is amended by revising paragraphs (c)(4)(ii)(A) through (C) to read as follows:

§ 217.10

Minimum capital requirements.

* * * * *

(c) * * *

(4) * * *

(ii) * * *

(A) The balance sheet carrying value of all the Board-regulated institution's on-balance sheet assets, plus the value of securities sold under a repurchase transaction or a securities lending transaction that qualifies for sales treatment under U.S. GAAP, less amounts deducted from tier 1 capital under § 217.22(a), (c), and (d), less the value of securities received in security-for-security repo-style transactions, where the Board-regulated institution acts as a securities lender and includes the securities received in its on-balance sheet assets but has not sold or re-hypothecated the securities received, and less the fair value of any derivative contracts;

(B) The PFE for each netting set (including cleared transactions except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the Board-regulated institution, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP), as determined under § 217.132(c)(7), in which the term C in § 217.132(c)(7)(i)(B) equals zero, multiplied by 1.4;

(C) The sum of:

(1)(i) 1.4 multiplied by the replacement cost of each derivative contract or single product netting set of derivative contracts to which the Board-regulated institution is a counterparty, calculated according to the following formula:

Replacement Cost = {V − CVM_r + CVM_p; 0}

Where:

V equals the fair value for each derivative contract or each single-product netting set of derivative contracts (including a cleared transaction except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the Board-regulated institution, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP);

CVM_r equals the amount of cash collateral received from a counterparty to a derivative contract and that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section; and

CVM_p equals the amount of cash collateral that is posted to a counterparty to a derivative contract and that has not off-set the fair value of the derivative contract and that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section; and

(ii) Notwithstanding paragraph (c)(4)(ii)(C)(1)(i) of this section, where multiple netting sets are subject to a single variation margin agreement, a Board-regulated institution must apply the formula for replacement cost provided in § 217.132(c)(10), in which the term may only include cash collateral that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(2) The amount of cash collateral that is received from a counterparty to a derivative contract that has off-set the fair value of a derivative contract and that does not satisfy the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(3) For derivative contracts that are not cleared through a QCCP, the cash collateral received by the recipient counterparty is not segregated (by law, regulation or an agreement with the counterparty);

(4) Variation margin is calculated and transferred on a daily basis based on the fair value of the derivative contract;

(5) The variation margin transferred under the derivative contract or the governing rules for a cleared transaction is the full amount that is necessary to fully extinguish the net current credit exposure to the counterparty of the derivative contracts, subject to the threshold and minimum transfer amounts applicable to the counterparty under the terms of the derivative contract or the governing rules for a cleared transaction;

(6) The variation margin is in the form of cash in the same currency as the currency of settlement set forth in the derivative contract, provided that for the purposes of this paragraph (c)(4)(ii)(C)(6), currency of settlement means any currency for settlement specified in the governing qualifying master netting agreement and the credit support annex to the qualifying master netting agreement, or in the governing rules for a cleared transaction; and

(7) The derivative contract and the variation margin are governed by a qualifying master netting agreement between the legal entities that are the counterparties to the derivative contract or by the governing rules for a cleared transaction, and the qualifying master netting agreement or the governing rules for a cleared transaction must explicitly stipulate that the counterparties agree to settle any payment obligations on a net basis, taking into account any variation margin received or provided under the contract if a credit event involving either counterparty occurs;

* * * * *

17. Section 217.32 is amended by revising paragraph (f) to read as follows:

§ 217.32

General risk weights.

* * * * *

(f) Corporate exposures. (1) A Board-regulated institution must assign a 100 percent risk weight to all its corporate exposures, except as provided in paragraph (f)(2) of this section.

(2) A Board-regulated institution must assign a 2 percent risk weight to an exposure to a QCCP arising from the Board-regulated institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 217.35(b)(3)(i)(A) and a 4 percent risk weight to an exposure to a QCCP arising from the Board-regulated institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 217.35(b)(3)(i)(B).

(3) A Board-regulated institution must assign a 2 percent risk weight to an exposure to a QCCP arising from the Board-regulated institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 217.35(c)(3)(i).

* * * * *

18. Section 217.34 is revised to read as follows:

§ 217.34

Derivative contracts.

(a) Exposure amount for derivative contracts—(1) Board-regulated institution that is not an advanced approaches Board-regulated institution. (i) A Board-regulated institution that is not an advanced approaches Board-regulated institution must use the current exposure methodology (CEM) described in paragraph (b) of this section to calculate the exposure amount for all its OTC derivative contracts, unless the Board-regulated institution makes the election provided in paragraph (a)(1)(ii) of this section.

(ii) A Board-regulated institution that is not an advanced approaches Board-regulated institution may elect to calculate the exposure amount for all its OTC derivative contracts under the standardized approach for counterparty credit risk (SA-CCR) in § 217.132(c), rather than calculating the exposure amount for all its derivative contracts using the CEM. A Board-regulated institution that elects under this paragraph (a)(1)(ii) to calculate the exposure amount for its OTC derivative contracts under the SA-CCR must apply the treatment of cleared transactions under § 217.133 to its derivative contracts that are cleared transactions, rather than applying § 217.35. A Board-regulated institution that is not an advanced approaches Board-regulated institution must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the Board.

(2) Advanced approaches Board-regulated institution. An advanced approaches Board-regulated institution must calculate the exposure amount for all its derivative contracts using the SA-CCR in § 217.132(c). An advanced approaches Board-regulated institution must apply the treatment of cleared transactions under § 217.133 to its derivative contracts that are cleared transactions.

(b) Current exposure methodology exposure amount—(1) Single OTC derivative contract. Except as modified by paragraph (c) of this section, the exposure amount for a single OTC derivative contract that is not subject to a qualifying master netting agreement is equal to the sum of the Board-regulated institution's current credit exposure and potential future credit exposure (PFE) on the OTC derivative contract.

(i) Current credit exposure. The current credit exposure for a single OTC derivative contract is the greater of the fair value of the OTC derivative contract or zero.

(ii) PFE. (A) The PFE for a single OTC derivative contract, including an OTC derivative contract with a negative fair value, is calculated by multiplying the notional principal amount of the OTC derivative contract by the appropriate conversion factor in Table 1 to this section.

(B) For purposes of calculating either the PFE under this paragraph (b) or the gross PFE under paragraph (b)(2) of this section for exchange rate contracts and other similar contracts in which the notional principal amount is equivalent to the cash flows, notional principal amount is the net receipts to each party falling due on each value date in each currency.

(C) For an OTC derivative contract that does not fall within one of the specified categories in Table 1 to this section, the PFE must be calculated using the appropriate “other” conversion factor.

(D) A Board-regulated institution must use an OTC derivative contract's effective notional principal amount (that is, the apparent or stated notional principal amount multiplied by any multiplier in the OTC derivative contract) rather than the apparent or stated notional principal amount in calculating PFE.

(E) The PFE of the protection provider of a credit derivative is capped at the net present value of the amount of unpaid premiums.

Table 1 to § 217.34—Conversion Factor Matrix for Derivative Contracts ¹

Remaining maturity 2	Interest rate	Foreign exchange rate and gold	Credit (investment grade reference asset) 3	Credit (non-investment-grade reference asset)	Equity	Precious metals (except gold)	Other
One year or less	0.00	0.01	0.05	0.10	0.06	0.07	0.10
Greater than one year and less than or equal to five years	0.005	0.05	0.05	0.10	0.08	0.07	0.12
Greater than five years	0.015	0.075	0.05	0.10	0.10	0.08	0.15
1 For a derivative contract with multiple exchanges of principal, the conversion factor is multiplied by the number of remaining payments in the derivative contract.
2 For an OTC derivative contract that is structured such that on specified dates any outstanding exposure is settled and the terms are reset so that the fair value of the contract is zero, the remaining maturity equals the time until the next reset date. For an interest rate derivative contract with a remaining maturity of greater than one year that meets these criteria, the minimum conversion factor is 0.005.
3 A Board-regulated institution must use the column labeled “Credit (investment-grade reference asset)” for a credit derivative whose reference asset is an outstanding unsecured long-term debt security without credit enhancement that is investment grade. A Board-regulated institution must use the column labeled “Credit (non-investment-grade reference asset)” for all other credit derivatives.

(2) Multiple OTC derivative contracts subject to a qualifying master netting agreement. Except as modified by paragraph (c) of this section, the exposure amount for multiple OTC derivative contracts subject to a qualifying master netting agreement is equal to the sum of the net current credit exposure and the adjusted sum of the PFE amounts for all OTC derivative contracts subject to the qualifying master netting agreement.

(i) Net current credit exposure. The net current credit exposure is the greater of the net sum of all positive and negative fair values of the individual OTC derivative contracts subject to the qualifying master netting agreement or zero.

(ii) Adjusted sum of the PFE amounts. The adjusted sum of the PFE amounts, Anet, is calculated as Anet = (0.4 × Agross) + (0.6 × NGR × Agross), where:

(A) Agross = the gross PFE (that is, the sum of the PFE amounts as determined under paragraph (b)(1)(ii) of this section for each individual derivative contract subject to the qualifying master netting agreement); and

(B) Net-to-gross Ratio (NGR) = the ratio of the net current credit exposure to the gross current credit exposure. In calculating the NGR, the gross current credit exposure equals the sum of the positive current credit exposures (as determined under paragraph (b)(1)(i) of this section) of all individual derivative contracts subject to the qualifying master netting agreement.

(c) Recognition of credit risk mitigation of collateralized OTC derivative contracts. (1) A Board-regulated institution using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures an OTC derivative contract or multiple OTC derivative contracts subject to a qualifying master netting agreement (netting set) by using the simple approach in § 217.37(b).

(2) As an alternative to the simple approach, a Board-regulated institution using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures such a contract or netting set if the financial collateral is marked-to-fair value on a daily basis and subject to a daily margin maintenance requirement by applying a risk weight to the uncollateralized portion of the exposure, after adjusting the exposure amount calculated under paragraph (b)(1) or (2) of this section using the collateral haircut approach in § 217.37(c). The Board-regulated institution must substitute the exposure amount calculated under paragraph (b)(1) or (2) of this section for ΣE in the equation in § 217.37(c)(2).

(d) Counterparty credit risk for credit derivatives—(1) Protection purchasers. A Board-regulated institution that purchases a credit derivative that is recognized under § 217.36 as a credit risk mitigant for an exposure that is not a covered position under subpart F of this part is not required to compute a separate counterparty credit risk capital requirement under § 217.32 provided that the Board-regulated institution does so consistently for all such credit derivatives. The Board-regulated institution must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure to all relevant counterparties for risk-based capital purposes.

(2) Protection providers. (i) A Board-regulated institution that is the protection provider under a credit derivative must treat the credit derivative as an exposure to the underlying reference asset. The Board-regulated institution is not required to compute a counterparty credit risk capital requirement for the credit derivative under § 217.32, provided that this treatment is applied consistently for all such credit derivatives. The Board-regulated institution must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure.

(ii) The provisions of this paragraph (d)(2) apply to all relevant counterparties for risk-based capital purposes unless the Board-regulated institution is treating the credit derivative as a covered position under subpart F of this part, in which case the Board-regulated institution must compute a supplemental counterparty credit risk capital requirement under this section.

(e) Counterparty credit risk for equity derivatives. (1) A Board-regulated institution must treat an equity derivative contract as an equity exposure and compute a risk-weighted asset amount for the equity derivative contract under §§ 217.51 through 217.53 (unless the Board-regulated institution is treating the contract as a covered position under subpart F of this part).

(2) In addition, the Board-regulated institution must also calculate a risk-based capital requirement for the counterparty credit risk of an equity derivative contract under this section if the Board-regulated institution is treating the contract as a covered position under subpart F of this part.

(3) If the Board-regulated institution risk weights the contract under the Simple Risk-Weight Approach (SRWA) in § 217.52, the Board-regulated institution may choose not to hold risk-based capital against the counterparty credit risk of the equity derivative contract, as long as it does so for all such contracts. Where the equity derivative contracts are subject to a qualified master netting agreement, a Board-regulated institution using the SRWA must either include all or exclude all of the contracts from any measure used to determine counterparty credit risk exposure.

(f) Clearing member Board-regulated institution's exposure amount. The exposure amount of a clearing member Board-regulated institution using the CEM under paragraph (b) of this section for an OTC derivative contract or netting set of OTC derivative contracts where the Board-regulated institution is either acting as a financial intermediary and enters into an offsetting transaction with a QCCP or where the Board-regulated institution provides a guarantee to the QCCP on the performance of the client equals the exposure amount calculated according to paragraph (b)(1) or (2) of this section multiplied by the scaling factor 0.71. If the Board-regulated institution determines that a longer period is appropriate, the Board-regulated institution must use a larger scaling factor to adjust for a longer holding period as follows:

Where H = the holding period greater than five days. Additionally, the Board may require the Board-regulated institution to set a longer holding period if the Board determines that a longer period is appropriate due to the nature, structure, or characteristics of the transaction or is commensurate with the risks associated with the transaction.

19. Section 217.35 is amended by adding paragraph (a)(3), revising paragraph (b)(4)(i), and adding paragraph (c)(3)(iii) to read as follows:

§ 217.35

Cleared transactions.

(a) * * *

(3) Alternate requirements. Notwithstanding any other provision of this section, an advanced approaches Board-regulated institution or a Board-regulated institution that is not an advanced approaches Board-regulated institution and that has elected to use SA-CCR under § 217.34(a)(1) must apply § 217.133 to its derivative contracts that are cleared transactions rather than this section.

(b) * * *

(4) * * *

(i) Notwithstanding any other requirements in this section, collateral posted by a clearing member client Board-regulated institution that is held by a custodian (in its capacity as custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(3) * * *

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member Board-regulated institution may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a CCP where the clearing member Board-regulated institution is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 217.3(a), and the clearing member Board-regulated institution is not obligated to reimburse the clearing member client in the event of the CCP default.

* * * * *

20. Section 217.37 is amended by revising paragraph (c)(3)(iii) to read as follows:

§ 217.37

Collateralized transactions.

* * * * *

(c) * * *

(3) * * *

(iii) For repo-style transactions and cleared transactions, a Board-regulated institution may multiply the standard supervisory haircuts provided in paragraphs (c)(3)(i) and (ii) of this section by the square root of 1/2 (which equals 0.707107).

* * * * *

§§ 217.134, 217.202, and 217.210

[Amended]

21. For each section listed in the following table, the footnote number listed in the “Old footnote number” column is redesignated as the footnote number listed in the “New footnote number” column as follows:

Section	Old footnote No.	New footnote No.
217.134(d)(3)	30	31
217.202, paragraph (1) introductory text of the definition of “Covered position”	31	32
217.202, paragraph (1)(i) of the definition of “Covered position”	32	33
217.210(e)(1)	33	34

22. Section 217.132 is amended by:

a. Revising paragraphs (b)(2)(ii)(A)( 3) through (5);

b. Adding paragraphs (b)(2)(ii)(A)( 6) and (7);

c. Revising paragraphs (c) heading and (c)(1) and (2) and (5) through (8);

d. Adding paragraphs (c)(9) through (12);

e. Removing “Table 3 to § 217.132” and adding in its place “Table 4 to this section” in paragraphs (e)(5)(i)(A) and (H); and

f. Redesignating Table 3 to § 217.132 as Table 4 to § 217.132.

The revisions and additions read as follows:

§ 217.132

Counterparty credit risk of repo-style transactions, eligible margin loans, and OTC derivative contracts.

* * * * *

(b) * * *

(2) * * *

(ii) * * *

(A) * * *

(3) For repo-style transactions and cleared transactions, a Board-regulated institution may multiply the supervisory haircuts provided in paragraphs (b)(2)(ii)(A)(1) and (2) of this section by the square root of 1/2 (which equals 0.707107).

(4) A Board-regulated institution must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days (for eligible margin loans) or five business days (for repo-style transactions), using the formula provide in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. If the number of trades in a netting set exceeds 5,000 at any time during a quarter, a Board-regulated institution must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days for the following quarter (except when a Board-regulated institution is calculating EAD for a cleared transaction under § 217.133). If a netting set contains one or more trades involving illiquid collateral, a Board-regulated institution must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days. If over the two previous quarters more than two margin disputes on a netting set have occurred that lasted more than the holding period, then the Board-regulated institution must adjust the supervisory haircuts upward for that netting set on the basis of a holding period that is at least two times the minimum holding period for that netting set.

(5)(i) A Board-regulated institution must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days for collateral associated derivative contracts that are not cleared transactions using the formula provided in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. For collateral associated with a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, a Board-regulated institution must use a holding period of twenty business days. If a netting set contains one or more trades involving illiquid collateral or a derivative contract that cannot be easily replaced, a Board-regulated institution must use a holding period of twenty business days.

(ii) Notwithstanding paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section, for collateral associated with a derivative contract that is subject to an outstanding dispute over variation margin, the holding period is twice the amount provide under paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section.

(6) A Board-regulated institution must adjust the standard supervisory haircuts upward, pursuant to the adjustments provided in paragraphs (b)(2)(ii)(A)(4) and (5) of this section, using the following formula:

Where:

TM equals a holding period of longer than 10 business days for eligible margin loans and derivative contracts or longer than 5 business days for repo-style transactions;

Hs equals the standard supervisory haircut; and

Ts equals 10 business days for eligible margin loans and derivative contracts or 5 business days for repo-style transactions.

(7) If the instrument a Board-regulated institution has lent, sold subject to repurchase, or posted as collateral does not meet the definition of financial collateral, the Board-regulated institution must use a 25.0 percent haircut for market price volatility (Hs).

* * * * *

(c) EAD for derivative contracts—(1) Options for determining EAD. A Board-regulated institution must determine the EAD for a derivative contract using the standardized approach for counterparty credit risk (SA-CCR) under paragraph (c)(5) of this section or using the internal models methodology described in paragraph (d) of this section. If a Board-regulated institution elects to use SA-CCR for one or more derivative contracts, the exposure amount determined under SA-CCR is the EAD for the derivative contract or derivatives contracts. A Board-regulation institution must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the Board.

(2) Definitions. For purposes of this paragraph (c), the following definitions apply:

(i) Except as otherwise provided in paragraph (c) of this section, the end date means the last date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references another instrument, by the underlying instrument.

(ii) Except as otherwise provided in paragraph (c) of this section, the start date means the first date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references the value of another instrument, by underlying instrument.

(iii) Hedging set means:

(A) With respect interest rate derivative contracts, all such contracts within a netting set that reference the same reference currency;

(B) With respect to exchange rate derivative contracts, all such contracts within a netting set that reference the same currency pair;

(C) With respect to credit derivative contract, all such contracts within a netting set;

(D) With respect to equity derivative contracts, all such contracts within a netting set;

(E) With respect to a commodity derivative contract, all such contracts within a netting set that reference one of the following commodity classes: Energy, metal, agricultural, or other commodities;

(F) With respect to basis derivative contracts, all such contracts within a netting set that reference the same pair of risk factors and are denominated in the same currency; or

(G) With respect to volatility derivative contracts, all such contracts within a netting set that reference one of interest rate, exchange rate, credit, equity, or commodity risk factors, separated according to the requirements under paragraphs (c)(2)(iii)(A) through (E) of this section.

(H) If the risk of a derivative contract materially depends on more than one of interest rate, exchange rate, credit, equity, or commodity risk factors, the Board may require a Board-regulated institution to include the derivative contract in each appropriate hedging set under paragraphs (c)(1)(iii)(A) through (E) of this section.

* * * * *

(5) Exposure amount. The exposure amount of a netting set, as calculated under paragraph (c) of this section, is equal to 1.4 multiplied by the sum of the replacement cost of the netting set, as calculated under paragraph (c)(6) of this section, and the potential future exposure of the netting set, as calculated under paragraph (c)(7) of this section, except that, notwithstanding the requirements of this paragraph (c)(5):

(i) The exposure amount of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty to the variation margin agreement is not required to post variation margin, is equal to the lesser of the exposure amount of the netting set and the exposure amount of the netting set calculated as if the netting set were not subject to a variation margin agreement; and

(ii) The exposure amount of a netting set that consists of only sold options in which the premiums have been fully paid and that are not subject to a variation margin agreement is zero.

(6) Replacement cost of a netting set—(i) Netting set subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty is not required to post variation margin, is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts;

(B) The sum of the variation margin threshold and the minimum transfer amount applicable to the derivative contracts within the netting set less the net independent collateral amount applicable to such derivative contracts; or

(C) Zero.

(ii) Netting sets not subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set that is not subject to a variation margin agreement under which the counterparty must post variation margin to the Board-regulated institution is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the net independent collateral amount and variation margin amount applicable to such derivative contracts; or

(B) Zero.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(i) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(i) of this section.

(7) Potential future exposure of a netting set. The potential future exposure of a netting set is the product of the PFE multiplier and the aggregated amount.

(i) PFE multiplier. The PFE multiplier is calculated according to the following formula:

Where:

V is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

C is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting set; and

A is the aggregated amount of the netting set.

(ii) Aggregated amount. The aggregated amount is the sum of all hedging set amounts, as calculated under paragraph (c)(8) of this section, within a netting set.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under § 217.10(c)(4)(ii)(B), the potential future exposure for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(ii) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under § 217.10(c)(4)(ii)(B), the potential future exposure for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(ii) of this section.

(8) Hedging set amount—(i) Interest rate derivative contracts. To calculate the hedging set amount of an interest rate derivative contract hedging set, a Board-regulated institution may use either of the formulas provided in paragraphs (c)(8)(i)(A) and (B) of this section:

(ii) Exchange rate derivative contracts. For an exchange rate derivative contract hedging set, the hedging set amount equals the absolute value of the sum of the adjusted derivative contract amounts, as calculated under paragraph (c)(9) of this section, within the hedging set.

(iii) Credit derivative contracts and equity derivative contracts. The hedging set amount of a credit derivative contract hedging set or equity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each reference entity within the hedging set.

K is the number of reference entities within the hedging set.

AddOn (Ref_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference reference entity k.

ρ_k equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(iv) Commodity derivative contracts. The hedging set amount of a commodity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each commodity type within the hedging set.

K is the number of commodity types within the hedging set.

AddOn (Type_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference reference commodity type k.

ρ equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(v) Basis derivative contracts and volatility derivative contracts. Notwithstanding paragraphs (c)(8)(i) through (iv) of this section, a Board-regulated institution must calculate a separate hedging set amount for each basis derivative contract hedging set and each volatility derivative contract hedging set. A Board-regulated institution must calculate such hedging set amounts using one of the formulas under paragraphs (c)(8)(i) through (iv) that corresponds to the primary risk factor of the hedging set being calculated.

(9) Adjusted derivative contract amount—(i) Summary. To calculate the adjusted derivative contract amount of a derivative contract, a Board-regulated institution must determine the adjusted notional amount of derivative contract, pursuant to paragraph (c)(9)(ii) of this section, and multiply the adjusted notional amount by each of the supervisory delta adjustment, pursuant to paragraph (c)(9)(iii) of this section, the maturity factor, pursuant to paragraph (c)(9)(iv) of this section, and the applicable supervisory factor, as provided in Table 2 to this section.

(ii) Adjusted notional amount. (A)(1) For an interest rate derivative contract or a credit derivative contract, the adjusted notional amount equals the product of the notional amount of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation, and the supervisory duration, as calculated by the following formula:

Where:

S is the number of business days from the present day until the start date of the derivative contract, or zero if the start date has already passed; and

E is the number of business days from the present day until the end date of the derivative contract.

(2) For purposes of paragraph (c)(9)(ii)(A)(1) of this section:

(i) For an interest rate derivative contract or credit derivative contract that is a variable notional swap, the notional amount is equal to the time-weighted average of the contractual notional amounts of such a swap over the remaining life of the swap; and

(ii) For an interest rate derivative contract or a credit derivative contract that is a leveraged swap, in which the notional amount of all legs of the derivative contract are divided by a factor and all rates of the derivative contract are multiplied by the same factor, the notional amount is equal to the notional amount of an equivalent unleveraged swap.

(B)(1) For an exchange rate derivative contract, the adjusted notional amount is the notional amount of the non-U.S. denominated currency leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation. If both legs of the exchange rate derivative contract are denominated in currencies other than U.S. dollars, the adjusted notional amount of the derivative contract is the largest leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation.

(2) Notwithstanding paragraph (c)(9)(i)(B)(1) of this section, for an exchange rate derivative contract with multiple exchanges of principal, the Board-regulated institution must set the adjusted notional amount of the derivative contract equal to the notional amount of the derivative contract multiplied by the number of exchanges of principal under the derivative contract.

(C)(1) For an equity derivative contract or a commodity derivative contract, the adjusted notional amount is the product of the fair value of one unit of the reference instrument underlying the derivative contract and the number of such units referenced by the derivative contract.

(2) Notwithstanding paragraph (c)(9)(i)(C)(1) of this section, when calculating the adjusted notional amount for an equity derivative contract or a commodity derivative contract that is a volatility derivative contract, the Board-regulated institution must replace the unit price with the underlying volatility referenced by the volatility derivative contract and replace the number of units with the notional amount of the volatility derivative contract.

(iii) Supervisory delta adjustments. (A) For a derivative contract that is not an option contract or collateralized debt obligation tranche, the supervisory delta adjustment is 1 if the fair value of the derivative contract increases when the value of the primary risk factor increases and −1 if the fair value of the derivative contract decreases when the value of the primary risk factor increases;

(B)(1) For a derivative contract that is an option contract, the supervisory delta adjustment is determined by the following formulas, as applicable:

(2) As used in the formulas in Table 3 to this section:

(i) F is the standard normal cumulative distribution function;

(ii) P equals the current fair value of the instrument or risk factor, as applicable, underlying the option;

(iii) K equals the strike price of the option;

(iv) T equals the number of business days until the latest contractual exercise date of the option;

(v) λ equals zero for all derivative contracts except interest rate options for the currencies where interest rates have negative values. The same value of λ must be used for all interest rate options that are denominated in the same currency. To determine the value of λ for a given currency, a Board-regulated institution must find the lowest value L of P and K of all interest rate options in a given currency that the Board-regulated institution has with all counterparties. Then, λ is set according to this formula: λ = max{−L + 0.1%, 0} and

(vi) σ equals the supervisory option volatility, as provided in Table 2 to this section.

(C)(1) For a derivative contract that is a collateralized debt obligation tranche, the supervisory delta adjustment is determined by the following formula:

(2) As used in the formula in paragraph (c)(9)(iii)(C)(1) of this section:

(i) A is the attachment point, which equals the ratio of the notional amounts of all underlying exposures that are subordinated to the Board-regulated institution's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; ^[30]

(ii) D is the detachment point, which equals one minus the ratio of the notional amounts of all underlying exposures that are senior to the Board-regulated institution's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; and

(iii) The resulting amount is designated with a positive sign if the collateralized debt obligation tranche was purchased by the Board-regulated institution and is designated with a negative sign if the collateralized debt obligation tranche was sold by the Board-regulated institution.

(iv) Maturity factor. (A)(1) The maturity factor of a derivative contract that is subject to a variation margin agreement, excluding derivative contracts that are subject to a variation margin agreement under which the counterparty is not required to post variation margin, is determined by the following formula:

Where MPOR refers to the period from the most recent exchange of collateral covering a netting set of derivative contracts with a defaulting counterparty until the derivative contracts are closed out and the resulting market risk is re-hedged.

(2) Notwithstanding paragraph (c)(9)(iv)(A)(1) of this section:

(i) For a derivative contract that is not a cleared transaction, MPOR cannot be less than ten business days plus the periodicity of re-margining expressed in business days minus one business day;

(ii) For a derivative contract that is a cleared transaction, MPOR cannot be less than five business days plus the periodicity of re-margining expressed in business days minus one business day; and

(iii) For a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, MPOR cannot be less than twenty business days.

(3) Notwithstanding paragraphs (c)(9)(iv)(A)(1) and (2) of this section, for a derivative contract subject to an outstanding dispute over variation margin, the applicable floor is twice the amount provided in (c)(9)(iv)(A)(1) and (2) of this section.

(B) The maturity factor of a derivative contract that is not subject to a variation margin agreement, or derivative contracts under which the counterparty is not required to post variation margin, is determined by the following formula:

Where M equals the greater of 10 business days and the remaining maturity of the contract, as measured in business days.

(C) For purposes of paragraph (c)(9)(iv) of this section, derivative contracts with daily settlement are treated as derivative contracts not subject to a variation margin agreement and daily settlement does not change the end date of the period referenced by the derivative contract.

(v) Derivative contract as multiple effective derivative contracts. A Board-regulated institution must separate a derivative contract into separate derivative contracts, according to the following rules:

(A) For an option where the counterparty pays a predetermined amount if the value of the underlying asset is above or below the strike price and nothing otherwise (binary option), the option must be treated as two separate options. For purposes of paragraph (c)(9)(iii)(B) of this section, a binary option with strike K must be represented as the combination of one bought European option and one sold European option of the same type as the original option (put or call) with the strikes set equal to 0.95*K and 1.05*K so that the payoff of the binary option is reproduced exactly outside the region between the two strikes. The absolute value of the sum of the adjusted derivative contract amounts of the bought and sold options is capped at the payoff amount of the binary option.

(B) For a derivative contract that can be represented as a combination of standard option payoffs (such as collar, butterfly spread, calendar spread, straddle, and strangle), each standard option component must be treated as a separate derivative contract.

(C) For a derivative contract that includes multiple-payment options, (such as interest rate caps and floors) each payment option may be represented as a combination of effective single-payment options (such as interest rate caplets and floorlets).

(10) Multiple netting sets subject to a single variation margin agreement—(i) Calculating replacement cost. Notwithstanding paragraph (c)(6) of this section, a Board-regulated institution shall assign a single replacement cost to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin, calculated according to the following formula:

Replacement Cost = max{Σ_NSmax {V _NS; 0} − max {C_MA; 0}; 0} + max{Σ_NSmin {V_NS;0} − min {C_MA 0}; 0}

Where:

NS is each netting set subject to the variation margin agreement MA;

V_NS is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set NS; and

C_MA is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting sets subject to the single variation margin agreement.

(ii) Calculating potential future exposure. Notwithstanding paragraph (c)(5) of this section, a Board-regulated institution shall assign a single potential future exposure to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin equal to the sum of the potential future exposure of each such netting set, each calculated according to paragraph (c)(7) of this section as if such nettings sets were not subject to a variation margin agreement.

(11) Netting set subject to multiple variation margin agreements or a hybrid netting set—(i) Calculating replacement cost. To calculate replacement cost for either a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, the calculation for replacement cost is provided under paragraph (c)(6)(ii) of this section, except that the variation margin threshold equals the sum of the variation margin thresholds of all variation margin agreements within the netting set and the minimum transfer amount equals the sum of the minimum transfer amounts of all the variation margin agreements within the netting set.

(ii) Calculating potential future exposure. (A) To calculate potential future exposure for a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty to the derivative contract must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, a Board-regulated institution must divide the netting set into sub-netting sets and calculate the aggregated amount for each sub-netting set. The aggregated amount for the netting set is calculated as the sum of the aggregated amounts for the sub-netting sets. The multiplier is calculated for the entire netting set.

(B) For purposes of paragraph (c)(11)(ii)(A) of this section, the netting set must be divided into sub-netting sets as follows:

(1) All derivative contracts within the netting set that are not subject to a variation margin agreement or that are subject to a variation margin agreement under which the counterparty is not required to post variation margin form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is not subject to a variation margin agreement.

(2) All derivative contracts within the netting set that are subject to variation margin agreements in which the counterparty must post variation margin and that share the same value of the MPOR form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is subject to a variation margin agreement, using the MPOR value shared by the derivative contracts within the netting set.

(12) Treatment of cleared transactions. (i) A Board-regulated institution must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more cleared transactions.

(ii) A Board-regulated institution that is a clearing member must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more exposures, each of which are exposures of the Board-regulated institution to its clearing member client where the Board-regulated institution is either acting as a financial intermediary and enters into an offsetting transaction with a CCP or where the Board-regulated institution provides a guarantee to the CCP on the performance of the client.

(iii)(A) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, MPOR may not be less than 10 business days;

(B) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, the minimum MPOR under paragraph (c)(9)(iv)(A)(3) of this section does not apply if there are no outstanding disputed trades in the netting set, there is no illiquid collateral in the netting set, and there are no exotic derivative contracts in the netting set; and

(C) For purposes of calculating the maturity factor under paragraphs (c)(9)(iv)(A) and (B) of this section, if the CCP collects and holds variation margin and the variation margin is not bankruptcy remote from the CCP, M_i may not exceed 250 business days.

Table 2 to § 217.132—Supervisory Option Volatility, Supervisory Correlation Parameters, and Supervisory Factors for Derivative Contracts

Asset class	Subclass	Supervisory option volatility (%)	Supervisory correlation factor (%)	Supervisory factor 1 (%)
Interest rate	N/A	50	N/A	0.50
Exchange rate	N/A	15	N/A	4.0
Credit, single name	Investment grade	100	50	0.5
	Speculative grade	100	50	1.3
	Sub-speculative grade	100	50	6.0
Credit, index	Investment Grade	80	80	0.38
	Speculative Grade	80	80	1.06
Equity, single name	N/A	120	50	32
Equity, index	N/A	75	80	20
Commodity	Energy	150	40	40
	Metals	70	40	18
	Agricultural	70	40	18
	Other	70	40	18
1 The applicable supervisory factor for basis derivative contract hedging sets is equal to one-half of the supervisory factor provided in this Table 2, and the applicable supervisory factor for volatility derivative contract hedging sets is equal to 5 times the supervisory factor provided in this Table 2.

* * * * *

23. Section 217.133 is amended by revising paragraphs (a), (b)(1) through (3), (b)(4)(i), (c)(1) through (3), (c)(4)(i), and (d) to read as follows:

§ 217.133

Cleared transactions.

(a) General requirements—(1) Clearing member clients. A Board-regulated institution that is a clearing member client must use the methodologies described in paragraph (b) of this section to calculate risk-weighted assets for a cleared transaction.

(2) Clearing members. A Board-regulated institution that is a clearing member must use the methodologies described in paragraph (c) of this section to calculate its risk-weighted assets for a cleared transaction and paragraph (d) of this section to calculate its risk-weighted assets for its default fund contribution to a CCP.

(b) * * *

(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a Board-regulated institution that is a clearing member client must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (b)(2) of this section, by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (b)(3) of this section.

(ii) A clearing member client Board-regulated institution's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. (i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD for the derivative contract or netting set of derivative contracts calculated using the methodology used to calculate EAD for derivative contracts set forth in § 217.132(c) or (d), plus the fair value of the collateral posted by the clearing member client Board-regulated institution and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the Board-regulated institution calculates EAD for the cleared transaction using the methodology in § 217.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD for the repo-style transaction calculated using the methodology set forth in § 217.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member client Board-regulated institution and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the Board-regulated institution calculates EAD for the cleared transaction under § 217.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) For a cleared transaction with a QCCP, a clearing member client Board-regulated institution must apply a risk weight of:

(A) 2 percent if the collateral posted by the Board-regulated institution to the QCCP or clearing member is subject to an arrangement that prevents any loss to the clearing member client Board-regulated institution due to the joint default or a concurrent insolvency, liquidation, or receivership proceeding of the clearing member and any other clearing member clients of the clearing member; and the clearing member client Board-regulated institution has conducted sufficient legal review to conclude with a well-founded basis (and maintains sufficient written documentation of that legal review) that in the event of a legal challenge (including one resulting from an event of default or from liquidation, insolvency or receivership proceedings) the relevant court and administrative authorities would find the arrangements to be legal, valid, binding and enforceable under the law of the relevant jurisdictions.

(B) 4 percent, if the requirements of paragraph (b)(3)(i)(A) of this section are not met.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member client Board-regulated institution must apply the risk weight applicable to the CCP under § 217.32.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client Board-regulated institution that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a clearing member Board-regulated institution must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (c)(2) of this section by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (c)(3) of this section.

(ii) A clearing member Board-regulated institution's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. A clearing member Board-regulated institution must calculate its trade exposure amount for a cleared transaction as follows:

(i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD calculated using the methodology used to calculate EAD for derivative contracts set forth in § 217.132(c) or (d), plus the fair value of the collateral posted by the clearing member Board-regulated institution and held by the CCP in a manner that is not bankruptcy remote. When the clearing member Board-regulated institution calculates EAD for the cleared transaction using the methodology in § 217.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD calculated under § 217.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member Board-regulated institution and held by the CCP in a manner that is not bankruptcy remote. When the clearing member Board-regulated institution calculates EAD for the cleared transaction under § 217.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) A clearing member Board-regulated institution must apply a risk weight of 2 percent to the trade exposure amount for a cleared transaction with a QCCP.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member Board-regulated institution must apply the risk weight applicable to the CCP according to § 217.32.

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member Board-regulated institution may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a QCCP where the clearing member Board-regulated institution is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 217.3(a), and the clearing member Board-regulated institution is not obligated to reimburse the clearing member client in the event of the QCCP default.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client Board-regulated institution that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(d) Default fund contributions—(1) General requirement. A clearing member Board-regulated institution must determine the risk-weighted asset amount for a default fund contribution to a CCP at least quarterly, or more frequently if, in the opinion of the Board-regulated institution or the Board, there is a material change in the financial condition of the CCP.

(2) Risk-weighted asset amount for default fund contributions to nonqualifying CCPs. A clearing member Board-regulated institution's risk-weighted asset amount for default fund contributions to CCPs that are not QCCPs equals the sum of such default fund contributions multiplied by 1,250 percent, or an amount determined by the Board, based on factors such as size, structure and membership characteristics of the CCP and riskiness of its transactions, in cases where such default fund contributions may be unlimited.

(3) Risk-weighted asset amount for default fund contributions to QCCPs. A clearing member Board-regulated institution's risk-weighted asset amount for default fund contributions to QCCPs equals the sum of its capital requirement, K_CM for each QCCP, as calculated under the methodology set forth in paragraph (e)(4) of this section.

(i) EAD must be calculated separately for each clearing member's sub-client accounts and sub-house account (i.e., for the clearing member's propriety activities). If the clearing member's collateral and its client's collateral are held in the same default fund contribution account, then the EAD of that account is the sum of the EAD for the client-related transactions within the account and the EAD of the house-related transactions within the account. For purposes of determining such EADs, the independent collateral of the clearing member and its client must be allocated in proportion to the respective total amount of independent collateral posted by the clearing member to the QCCP.

(ii) If any account or sub-account contains both derivative contracts and repo-style transactions, the EAD of that account is the sum of the EAD for the derivative contracts within the account and the EAD of the repo-style transactions within the account. If independent collateral is held for an account containing both derivative contracts and repo-style transactions, then such collateral must be allocated to the derivative contracts and repo-style transactions in proportion to the respective product specific exposure amounts, calculated, excluding the effects of collateral, according to § 217.132(b) for repo-style transactions and to § 217.132(c)(5) for derivative contracts.

(4) Risk-weighted asset amount for default fund contributions to a QCCP. A clearing member Board regulated institution's capital requirement for its default fund contribution to a QCCP (K_CM) is equal to:

(5) Hypothetical capital requirement of a QCCP. Where a QCCP has provided its K_CCP, a Board-regulated institution must rely on such disclosed figure instead of calculating K_CCP under this paragraph (d)(5), unless the Board-regulated institution determines that a more conservative figure is appropriate based on the nature, structure, or characteristics of the QCCP. The hypothetical capital requirement of a QCCP (K_CCP), as determined by the Board-regulated institution, is equal to:

K_CCP = S_CMiEAD_i * 1.6 percent

Where:

CM_i is each clearing member of the QCCP; and

EAD_i is the exposure amount of each clearing member of the QCCP to the QCCP, as determined under paragraph (d)(6) of this section.

(6) EAD of a clearing member Board-regulated institution to a QCCP. (i) The EAD of a clearing member Board-regulated institution to a QCCP is equal to the sum of the EAD for derivative contracts determined under paragraph (d)(6)(ii) of this section and the EAD for repo-style transactions determined under paragraph (d)(6)(iii) of this section.

(ii) With respect to any derivative contracts between the Board-regulated institution and the CCP that are cleared transactions and any guarantees that the Board-regulated institution has provided to the CCP with respect to performance of a clearing member client on a derivative contract, the EAD is equal to the sum of:

(A) The exposure amount for all such derivative contracts and guarantees of derivative contracts calculated under SA-CCR in § 217.132(c) using a value of 10 business days for purposes of § 217.132(c)(9)(iv)(B);

(B) The value of all collateral held by the CCP posted by the clearing member Board-regulated institution or a clearing member client of the Board-regulated institution in connection with a derivative contract for which the Board-regulated institution has provided a guarantee to the CCP; and

(C) The amount of the prefunded default fund contribution of the Board-regulated institution to the CCP.

(iii) With respect to any repo-style transactions between the Board-regulated institution and the CCP that are cleared transactions, EAD is equal to:

EAD = max{EBRM−IM−DF; 0}

Where:

EBRM is the sum of the exposure amounts of each repo-style transaction between the Board-regulated institution and the CCP as determined under § 217.132(b)(2) and without recognition of any collateral securing the repo-style transactions;

IM is the initial margin collateral posted by the Board-regulated institution to the CCP with respect to the repo-style transactions; and

DF is the prefunded default fund contribution of the Board-regulation institution to the CCP.

24. Section 217.300 is amended by adding paragraph (g) to read as follows:

§ 217.300

Transitions.

* * * * *

(g) SA-CCR. After giving prior notice to the Board, an advanced approaches Board-regulated institution may use CEM rather than SA-CCR to determine the exposure amount for purposes of § 217.34 and the EAD for purposes of § 217.132 for its derivative contracts until July 1, 2020. On July 1, 2020, and thereafter, an advanced approaches Board-regulated institution must use SA-CCR for purposes of § 217.34 and must use either SA-CCR or IMM for purposes of § 217.132. Once an advanced approaches Board-regulated institution has begun to use SA-CCR, the advanced approaches Board-regulated institution may not change to use CEM.

12 CFR Part 324

Federal Deposit Insurance Corporation

For the reasons forth out in the preamble, 12 CFR part 324 is proposed to be amended as set forth below.

PART 324—CAPITAL ADEQUACY OF FDIC-SUPERVISED INSTITUTIONS

25. The authority citation for part 324 continues to read as follows:

Authority: 12 U.S.C. 1815(a), 1815(b), 1816, 1818(a), 1818(b), 1818(c), 1818(t), 1819(Tenth), 1828(c), 1828(d), 1828(i), 1828(n), 1828(o), 1831o, 1835, 3907, 3909, 4808; 5371; 5412; Pub. L. 102-233, 105 Stat. 1761, 1789, 1790 (12 U.S.C. 1831n note); Pub. L. 102-242, 105 Stat. 2236, 2355, as amended by Pub. L. 103-325, 108 Stat. 2160, 2233 (12 U.S.C. 1828 note); Pub. L. 102-242, 105 Stat. 2236, 2386, as amended by Pub. L. 102-550, 106 Stat. 3672, 4089 (12 U.S.C. 1828 note); Pub. L. 111-203, 124 Stat. 1376, 1887 (15 U.S.C. 78o-7 note).

26. Section 324.2 is amended by:

a. Adding the definition of “Basis derivative contract” in alphabetical order;

b. Revising paragraph (2) of the definition of “Financial collateral;”

c. Adding the definitions of “Independent collateral,” “Minimum transfer amount,” and “Net independent collateral amount” in alphabetical order.

d. Revising the definition of “Netting set;” and

e. Adding the definitions of “Speculative grade,” “Sub-speculative grade,” “Variation margin,” “Variation margin agreement,” “Variation margin amount,” “Variation margin threshold,” and “Volatility derivative contract” in alphabetical order.

The additions and revisions read as follows:

§ 324.2

Definitions.

* * * * *

Basis derivative contract means a non-foreign-exchange derivative contract (i.e., the contract is denominated in a single currency) in which the cash flows of the derivative contract depend on the difference between two risk factors that are attributable solely to one of the following derivative asset classes: Interest rate, credit, equity, or commodity.

* * * * *

Financial collateral * * *

(2) In which the FDIC-supervised institution has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; and notwithstanding the prior security interest of any custodial agent or any priority security interest granted to a CCP in connection with collateral posted to that CCP).

* * * * *

Independent collateral means financial collateral, other than variation margin that is subject to a collateral agreement, or in which a FDIC-supervised institution has a perfected, first-priority security interest or, outside of the United States, the legal equivalent thereof (with the exception of cash on deposit; notwithstanding the prior security interest of any custodial agent or any prior security interest granted to a CCP in connection with collateral posted to that CCP), and the amount of which does not change directly in response to the value of the derivative contract or contracts that the financial collateral secures.

* * * * *

Minimum transfer amount means the smallest amount of variation margin that may be transferred between counterparties to a netting set.

* * * * *

Net independent collateral amount means the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 324.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a FDIC-supervised institution less the fair value amount of the independent collateral, as adjusted by the standard supervisory haircuts under § 324.132(b)(2)(ii), as applicable, posted by the FDIC-supervised institution to the counterparty, excluding such amounts held in a bankruptcy remote manner, or posted to a QCCP and held in conformance with the operational requirements in § 324.3.

Netting set means either one derivative contract between a FDIC-supervised institution and a single counterparty, or a group of derivative contracts between a FDIC-supervised institution and a single counterparty, that are subject to a qualifying master netting agreement.

* * * * *

Speculative grade means the reference entity has adequate capacity to meet financial commitments in the near term, but is vulnerable to adverse economic conditions, such that should economic conditions deteriorate, the reference entity would present an elevated default risk.

* * * * *

Sub-speculative grade means the reference entity depends on favorable economic conditions to meet its financial commitments, such that should such economic conditions deteriorate the reference entity likely would default on its financial commitments.

* * * * *

Variation margin means financial collateral that is subject to a collateral agreement provided by one party to its counterparty to meet the performance of the first party's obligations under one or more transactions between the parties as a result of a change in value of such obligations since the last time such financial collateral was provided.

Variation margin agreement means an agreement to collect or post variation margin.

Variation margin amount means the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 324.132(b)(2)(ii), as applicable, that a counterparty to a netting set has posted to a FDIC-supervised institution less the fair value amount of the variation margin, as adjusted by the standard supervisory haircuts under § 324.132(b)(2)(ii), as applicable, posted by the FDIC-supervised institution to the counterparty.

Variation margin threshold means the amount of credit exposure of a FDIC-supervised institution to its counterparty that, if exceeded, would require the counterparty to post variation margin to the FDIC-supervised institution.

Volatility derivative contract means a derivative contract in which the payoff of the derivative contract explicitly depends on a measure of the volatility of an underlying risk factor to the derivative contract.

* * * * *

27. Section 324.10 is amended by revising paragraphs (c)(4)(ii)(A) through (C) to read as follows:

§ 324.10

Minimum capital requirements.

* * * * *

(c) * * *

(4) * * *

(ii) * * *

(A) The balance sheet carrying value of all the FDIC-supervised institution's on-balance sheet assets, plus the value of securities sold under a repurchase transaction or a securities lending transaction that qualifies for sales treatment under U.S. GAAP, less amounts deducted from tier 1 capital under § 324.22(a), (c), and (d), less the value of securities received in security-for-security repo-style transactions, where the FDIC-supervised institution acts as a securities lender and includes the securities received in its on-balance sheet assets but has not sold or re-hypothecated the securities received, and less the fair value of any derivative contracts;

(B) The PFE for each netting set (including cleared transactions except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the FDIC-supervised institution, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP), as determined under § 324.132(c)(7), in which the term C in § 324.132(c)(7)(i)(B) equals zero, multiplied by 1.4;

(C) The sum of:

(1)(i) 1.4 multiplied by the replacement cost of each derivative contract or single product netting set of derivative contracts to which the FDIC-supervised institution is a counterparty, calculated according to the following formula:

Replacement Cost = max{V − CVM_r + CVM_p; 0}

Where:

V equals the fair value for each derivative contract or each single-product netting set of derivative contracts (including a cleared transaction except as provided in paragraph (c)(4)(ii)(I) of this section and, at the discretion of the FDIC-supervised institution, excluding a forward agreement treated as a derivative contract that is part of a repurchase or reverse repurchase or a securities borrowing or lending transaction that qualifies for sales treatment under U.S. GAAP);

CVM_r equals the amount of cash collateral received from a counterparty to a derivative contract and that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7); and

CVM_p equals the amount of cash collateral that is posted to a counterparty to a derivative contract and that has not off-set the fair value of the derivative contract and that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section; and

(ii) Notwithstanding paragraph (c)(4)(ii)(C)(1)(i) of this section, where multiple netting sets are subject to a single variation margin agreement, a FDIC-supervised institution must apply the formula for replacement cost provided in § 324.132(c)(10), in which the term may only include cash collateral that satisfies the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(2) The amount of cash collateral that is received from a counterparty to a derivative contract that has off-set the fair value of a derivative contract and that does not satisfy the conditions in paragraphs (c)(4)(ii)(C)(3) through (7) of this section;

(3) For derivative contracts that are not cleared through a QCCP, the cash collateral received by the recipient counterparty is not segregated (by law, regulation or an agreement with the counterparty);

(4) Variation margin is calculated and transferred on a daily basis based on the fair value of the derivative contract;

(5) The variation margin transferred under the derivative contract or the governing rules for a cleared transaction is the full amount that is necessary to fully extinguish the net current credit exposure to the counterparty of the derivative contracts, subject to the threshold and minimum transfer amounts applicable to the counterparty under the terms of the derivative contract or the governing rules for a cleared transaction;

(6) The variation margin is in the form of cash in the same currency as the currency of settlement set forth in the derivative contract, provided that for the purposes of this paragraph, currency of settlement means any currency for settlement specified in the governing qualifying master netting agreement and the credit support annex to the qualifying master netting agreement, or in the governing rules for a cleared transaction; and

(7) The derivative contract and the variation margin are governed by a qualifying master netting agreement between the legal entities that are the counterparties to the derivative contract or by the governing rules for a cleared transaction, and the qualifying master netting agreement or the governing rules for a cleared transaction must explicitly stipulate that the counterparties agree to settle any payment obligations on a net basis, taking into account any variation margin received or provided under the contract if a credit event involving either counterparty occurs;

* * * * *

28. Section 324.32 is amended by revising paragraph (f) to read as follows:

§ 324.32

General risk weights.

* * * * *

(f) Corporate exposures. (1) A FDIC-supervised institution must assign a 100 percent risk weight to all its corporate exposures, except as provided in paragraph (f)(2) of this section.

(2) A FDIC-supervised institution must assign a 2 percent risk weight to an exposure to a QCCP arising from the FDIC-supervised institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 324.35(b)(3)(i)(A) and a 4 percent risk weight to an exposure to a QCCP arising from the FDIC-supervised institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 324.35(b)(3)(i)(B).

(3) A FDIC-supervised institution must assign a 2 percent risk weight to an exposure to a QCCP arising from the FDIC-supervised institution posting cash collateral to the QCCP in connection with a cleared transaction that meets the requirements of § 324.35(c)(3)(i).

* * * * *

29. Section 324.34 is revised to read as follows:

§ 324.34

Derivative contracts.

(a) Exposure amount for derivative contracts—(1) FDIC-supervised institution that is not an advanced approaches FDIC-supervised institution. (i) A FDIC-supervised institution that is not an advanced approaches FDIC-supervised institution must use the current exposure methodology (CEM) described in paragraph (b) of this section to calculate the exposure amount for all its OTC derivative contracts, unless the FDIC-supervised institution makes the election provided in paragraph (a)(1)(ii) of this section.

(ii) A FDIC-supervised institution that is not an advanced approaches FDIC-supervised institution may elect to calculate the exposure amount for all its OTC derivative contracts under the standardized approach for counterparty credit risk (SA-CCR) in § 324.132(c), rather than calculating the exposure amount for all its derivative contracts using the CEM. A FDIC-supervised institution that elects under this paragraph (a)(1)(ii) to calculate the exposure amount for its OTC derivative contracts under the SA-CCR must apply the treatment of cleared transactions under § 324.133 to its derivative contracts that are cleared transactions, rather than applying § 324.35. A FDIC-supervised institution that is not an advanced approaches FDIC-supervised institution must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the FDIC.

(2) Advanced approaches FDIC-supervised institution. An advanced approaches FDIC-supervised institution must calculate the exposure amount for all its derivative contracts using the SA-CCR in § 324.132(c). An advanced approaches FDIC-supervised institution must apply the treatment of cleared transactions under § 324.133 to its derivative contracts that are cleared transactions.

(b) Current exposure methodology exposure amount—(1) Single OTC derivative contract. Except as modified by paragraph (c) of this section, the exposure amount for a single OTC derivative contract that is not subject to a qualifying master netting agreement is equal to the sum of the FDIC-supervised institution's current credit exposure and potential future credit exposure (PFE) on the OTC derivative contract.

(i) Current credit exposure. The current credit exposure for a single OTC derivative contract is the greater of the fair value of the OTC derivative contract or zero.

(ii) PFE. (A) The PFE for a single OTC derivative contract, including an OTC derivative contract with a negative fair value, is calculated by multiplying the notional principal amount of the OTC derivative contract by the appropriate conversion factor in Table 1 to of this section.

(B) For purposes of calculating either the PFE under this paragraph (b) or the gross PFE under paragraph (b)(2) of this section for exchange rate contracts and other similar contracts in which the notional principal amount is equivalent to the cash flows, notional principal amount is the net receipts to each party falling due on each value date in each currency.

(C) For an OTC derivative contract that does not fall within one of the specified categories in Table 1 to this section, the PFE must be calculated using the appropriate “other” conversion factor.

(D) A FDIC-supervised institution must use an OTC derivative contract's effective notional principal amount (that is, the apparent or stated notional principal amount multiplied by any multiplier in the OTC derivative contract) rather than the apparent or stated notional principal amount in calculating PFE.

(E) The PFE of the protection provider of a credit derivative is capped at the net present value of the amount of unpaid premiums.

Table 1 to § 324.34—Conversion Factor Matrix for Derivative Contracts ¹

Remaining maturity 2	Interest rate	Foreign exchange rate and gold	Credit (investment grade reference asset) 3	Credit (non-investment-grade reference asset)	Equity	Precious metals (except gold)	Other
One year or less	0.00	0.01	0.05	0.10	0.06	0.07	0.10
Greater than one year and less than or equal to five years	0.005	0.05	0.05	0.10	0.08	0.07	0.12
Greater than five years	0.015	0.075	0.05	0.10	0.10	0.08	0.15
1 For a derivative contract with multiple exchanges of principal, the conversion factor is multiplied by the number of remaining payments in the derivative contract.
2 For an OTC derivative contract that is structured such that on specified dates any outstanding exposure is settled and the terms are reset so that the fair value of the contract is zero, the remaining maturity equals the time until the next reset date. For an interest rate derivative contract with a remaining maturity of greater than one year that meets these criteria, the minimum conversion factor is 0.005.
3 A FDIC-supervised institution must use the column labeled “Credit (investment-grade reference asset)” for a credit derivative whose reference asset is an outstanding unsecured long-term debt security without credit enhancement that is investment grade. A FDIC-supervised institution must use the column labeled “Credit (non-investment-grade reference asset)” for all other credit derivatives.

(2) Multiple OTC derivative contracts subject to a qualifying master netting agreement. Except as modified by paragraph (c) of this section, the exposure amount for multiple OTC derivative contracts subject to a qualifying master netting agreement is equal to the sum of the net current credit exposure and the adjusted sum of the PFE amounts for all OTC derivative contracts subject to the qualifying master netting agreement.

(i) Net current credit exposure. The net current credit exposure is the greater of the net sum of all positive and negative fair values of the individual OTC derivative contracts subject to the qualifying master netting agreement or zero.

(ii) Adjusted sum of the PFE amounts. The adjusted sum of the PFE amounts, Anet, is calculated as Anet = (0.4 × Agross) + (0.6 × NGR × Agross), where:

(A) Agross = the gross PFE (that is, the sum of the PFE amounts as determined under paragraph (b)(1)(ii) of this section for each individual derivative contract subject to the qualifying master netting agreement); and

(B) Net-to-gross Ratio (NGR) = the ratio of the net current credit exposure to the gross current credit exposure. In calculating the NGR, the gross current credit exposure equals the sum of the positive current credit exposures (as determined under paragraph (b)(1)(i) of this section) of all individual derivative contracts subject to the qualifying master netting agreement.

(c) Recognition of credit risk mitigation of collateralized OTC derivative contracts. (1) A FDIC-supervised institution using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures an OTC derivative contract or multiple OTC derivative contracts subject to a qualifying master netting agreement (netting set) by using the simple approach in § 324.37(b).

(2) As an alternative to the simple approach, a FDIC-supervised institution using the CEM under paragraph (b) of this section may recognize the credit risk mitigation benefits of financial collateral that secures such a contract or netting set if the financial collateral is marked-to-fair value on a daily basis and subject to a daily margin maintenance requirement by applying a risk weight to the uncollateralized portion of the exposure, after adjusting the exposure amount calculated under paragraph (b)(1) or (2) of this section using the collateral haircut approach in § 324.37(c). The FDIC-supervised institution must substitute the exposure amount calculated under paragraph (b)(1) or (2) of this section for ΣE in the equation in § 324.37(c)(2).

(d) Counterparty credit risk for credit derivatives—(1) Protection purchasers. A FDIC-supervised institution that purchases a credit derivative that is recognized under § 324.36 as a credit risk mitigant for an exposure that is not a covered position under subpart F of this part is not required to compute a separate counterparty credit risk capital requirement under § 324.32 provided that the FDIC-supervised institution does so consistently for all such credit derivatives. The FDIC-supervised institution must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure to all relevant counterparties for risk-based capital purposes.

(2) Protection providers. (i) A FDIC-supervised institution that is the protection provider under a credit derivative must treat the credit derivative as an exposure to the underlying reference asset. The FDIC-supervised institution is not required to compute a counterparty credit risk capital requirement for the credit derivative under § 324.32, provided that this treatment is applied consistently for all such credit derivatives. The FDIC-supervised institution must either include all or exclude all such credit derivatives that are subject to a qualifying master netting agreement from any measure used to determine counterparty credit risk exposure.

(ii) The provisions of this paragraph (d)(2) apply to all relevant counterparties for risk-based capital purposes unless the FDIC-supervised institution is treating the credit derivative as a covered position under subpart F of this part, in which case the FDIC-supervised institution must compute a supplemental counterparty credit risk capital requirement under this section.

(e) Counterparty credit risk for equity derivatives. (1) A FDIC-supervised institution must treat an equity derivative contract as an equity exposure and compute a risk-weighted asset amount for the equity derivative contract under §§ 324.51 through 324.53 (unless the FDIC-supervised institution is treating the contract as a covered position under subpart F of this part).

(2) In addition, the FDIC-supervised institution must also calculate a risk-based capital requirement for the counterparty credit risk of an equity derivative contract under this section if the FDIC-supervised institution is treating the contract as a covered position under subpart F of this part.

(3) If the FDIC-supervised institution risk weights the contract under the Simple Risk-Weight Approach (SRWA) in § 324.52, the FDIC-supervised institution may choose not to hold risk-based capital against the counterparty credit risk of the equity derivative contract, as long as it does so for all such contracts. Where the equity derivative contracts are subject to a qualified master netting agreement, a FDIC-supervised institution using the SRWA must either include all or exclude all of the contracts from any measure used to determine counterparty credit risk exposure.

(f) Clearing member FDIC-supervised institution's exposure amount. The exposure amount of a clearing member FDIC-supervised institution using the CEM under paragraph (b) of this section for an OTC derivative contract or netting set of OTC derivative contracts where the FDIC-supervised institution is either acting as a financial intermediary and enters into an offsetting transaction with a QCCP or where the FDIC-supervised institution provides a guarantee to the QCCP on the performance of the client equals the exposure amount calculated according to paragraph (b)(1) or (2) of this section multiplied by the scaling factor 0.71. If the FDIC-supervised institution determines that a longer period is appropriate, the FDIC-supervised institution must use a larger scaling factor to adjust for a longer holding period as follows:

Where H = the holding period greater than five days. Additionally, the FDIC may require the FDIC-supervised institution to set a longer holding period if the FDIC determines that a longer period is appropriate due to the nature, structure, or characteristics of the transaction or is commensurate with the risks associated with the transaction.

30. Section 324.35 is amended by adding paragraph (a)(3), revising paragraph (b)(4)(i), and adding paragraph (c)(3)(iii) to read as follows:

§ 324.35

Cleared transactions.

(a) * * *

(3) Alternate requirements. Notwithstanding any other provision of this section, an advanced approaches FDIC-supervised institution or a FDIC-supervised institution that is not an advanced approaches FDIC-supervised institution and that has elected to use SA-CCR under § 324.34(a)(1) must apply § 324.133 to its derivative contracts that are cleared transactions rather than this section § 324.35.

(b) * * *

(4) * * *

(i) Notwithstanding any other requirements in this section, collateral posted by a clearing member client FDIC-supervised institution that is held by a custodian (in its capacity as custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(3) * * *

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member FDIC-supervised institution may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a CCP where the clearing member FDIC-supervised institution is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 324.3(a), and the clearing member FDIC-supervised institution is not obligated to reimburse the clearing member client in the event of the CCP default.

* * * * *

31. Section 324.37 is amended by revising paragraph (c)(3)(iii) to read as follows:

§ 324.37

Collateralized transactions.

* * * * *

(c) * * *

(3) * * *

(iii) For repo-style transactions and cleared transactions, a FDIC-supervised institution may multiply the standard supervisory haircuts provided in paragraphs (c)(3)(i) and (ii) of this section by the square root of 1/2 (which equals 0.707107).

* * * * *

§§ 324.134, 324.202, and 324.210

[Amended]

32. For each section listed in the following table, the footnote number listed in the “Old footnote number” column is redesignated as the footnote number listed in the “New footnote number” column as follows:

Section	Old footnote No.	New footnote No.
324.134(d)(3)	30	31
324.202, paragraph (1) introductory text of the definition of “Covered position”	31	32
324.202, paragraph (1)(i) of the definition of “Covered position”	32	33
324.210(e)(1)	33	34

33. Section 324.132 is amended by:

a. Revising paragraphs (b)(2)(ii)(A)( 3) through (5);

b. Adding paragraphs (b)(2)(ii)(A)( 6) and (7);

c. Revising paragraphs (c) heading and (c)(1) and (2) and (5) through (8);

d. Adding paragraphs (c)(9) through (12);

e. Removing “Table 3 to § 324.132” and adding in its place “Table 4 to this section” in paragraphs (e)(5)(i)(A) and (H); and

f. Redesignating Table 3 to § 324.132 as Table 4 to § 324.132.

The revisions and additions read as follows:

§ 324.132

Counterparty credit risk of repo-style transactions, eligible margin loans, and OTC derivative contracts.

* * * * *

(b) * * *

(2) * * *

(ii) * * *

(A) * * *

(3) For repo-style transactions and cleared transactions, a FDIC-supervised institution may multiply the supervisory haircuts provided in paragraphs (b)(2)(ii)(A)(1) and (2) of this section by the square root of 1/2 (which equals 0.707107).

(4) A FDIC-supervised institution must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days (for eligible margin loans) or five business days (for repo-style transactions), using the formula provide in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. If the number of trades in a netting set exceeds 5,000 at any time during a quarter, a FDIC-supervised institution must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days for the following quarter (except when a FDIC-supervised institution is calculating EAD for a cleared transaction under § 324.133). If a netting set contains one or more trades involving illiquid collateral, a FDIC-supervised institution must adjust the supervisory haircuts upward on the basis of a holding period of twenty business days. If over the two previous quarters more than two margin disputes on a netting set have occurred that lasted more than the holding period, then the FDIC-supervised institution must adjust the supervisory haircuts upward for that netting set on the basis of a holding period that is at least two times the minimum holding period for that netting set.

(5)(i) A FDIC-supervised institution must adjust the supervisory haircuts upward on the basis of a holding period longer than ten business days for collateral associated derivative contracts that are not cleared transactions using the formula provided in paragraph (b)(2)(ii)(A)(6) of this section where the following conditions apply. For collateral associated with a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, a FDIC-supervised institution must use a holding period of twenty business days. If a netting set contains one or more trades involving illiquid collateral or a derivative contract that cannot be easily replaced, a FDIC-supervised institution must use a holding period of twenty business days.

(ii) Notwithstanding paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section, for collateral associated with a derivative contract that is subject to an outstanding dispute over variation margin, the holding period is twice the amount provide under paragraph (b)(2)(ii)(A)(1) or (3) or (b)(2)(ii)(A)(5)(i) of this section.

(6) A FDIC-supervised institution must adjust the standard supervisory haircuts upward, pursuant to the adjustments provided in paragraphs (b)(2)(ii)(A)(4) and (5) of this section, using the following formula:

Where:

TM equals a holding period of longer than 10 business days for eligible margin loans and derivative contracts or longer than 5 business days for repo-style transactions;

Hs equals the standard supervisory haircut; and

Ts equals 10 business days for eligible margin loans and derivative contracts or 5 business days for repo-style transactions.

(7) If the instrument a FDIC-supervised institution has lent, sold subject to repurchase, or posted as collateral does not meet the definition of financial collateral, the FDIC-supervised institution must use a 25.0 percent haircut for market price volatility (Hs).

* * * * *

(c) EAD for derivative contracts—(1) Options for determining EAD. A FDIC-supervised institution must determine the EAD for a derivative contract using SA-CCR under paragraph (c)(5) of this section or using the internal models methodology described in paragraph (d) of this section. If a FDIC-supervised institution elects to use SA-CCR for one or more derivative contracts, the exposure amount determined under SA-CCR is the EAD for the derivative contract or derivatives contracts. A FDIC-supervised institution must use the same methodology to calculate the exposure amount for all its derivative contracts and may change its election only with prior approval of the FDIC.

(2) Definitions. For purposes of this paragraph (c), the following definitions apply:

(i) Except as otherwise provided in paragraph (c) of this section, the end date means the last date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references another instrument, by the underlying instrument.

(ii) Except as otherwise provided in paragraph (c) of this section, the start date means the first date of the period referenced by an interest rate or credit derivative contract or, if the derivative contract references the value of another instrument, by underlying instrument.

(iii) Hedging set means:

(A) With respect interest rate derivative contracts, all such contracts within a netting set that reference the same reference currency;

(B) With respect to exchange rate derivative contracts, all such contracts within a netting set that reference the same currency pair;

(C) With respect to credit derivative contract, all such contracts within a netting set;

(D) With respect to equity derivative contracts, all such contracts within a netting set;

(E) With respect to a commodity derivative contract, all such contracts within a netting set that reference one of the following commodity classes: Energy, metal, agricultural, or other commodities;

(F) With respect to basis derivative contracts, all such contracts within a netting set that reference the same pair of risk factors and are denominated in the same currency; or

(G) With respect to volatility derivative contracts, all such contracts within a netting set that reference one of interest rate, exchange rate, credit, equity, or commodity risk factors, separated according to the requirements under paragraphs (c)(2)(iii)(A) through (E) of this section.

(H) If the risk of a derivative contract materially depends on more than one of interest rate, exchange rate, credit, equity, or commodity risk factors, the FDIC may require a FDIC-supervised institution to include the derivative contract in each appropriate hedging set under paragraph (c)(2)(iii)(A) through (E) of this section.

* * * * *

(5) Exposure amount. The exposure amount of a netting set, as calculated under paragraph (c) of this section, is equal to 1.4 multiplied by the sum of the replacement cost of the netting set, as calculated under paragraph (c)(6) of this section, and the potential future exposure of the netting set, as calculated under paragraph (c)(7) of this section, except that, notwithstanding the requirements of this paragraph (c)(5):

(i) The exposure amount of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty to the variation margin agreement is not required to post variation margin, is equal to the lesser of the exposure amount of the netting set and the exposure amount of the netting set calculated as if the netting set were not subject to a variation margin agreement; and

(ii) The exposure amount of a netting set that consists of only sold options in which the premiums have been fully paid and that are not subject to a variation margin agreement is zero.

(6) Replacement cost of a netting set—(i) Netting set subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set subject to a variation margin agreement, excluding a netting set that is subject to a variation margin agreement under which the counterparty is not required to post variation margin, is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the sum of the net independent collateral amount and the variation margin amount applicable to such derivative contracts;

(B) The sum of the variation margin threshold and the minimum transfer amount applicable to the derivative contracts within the netting set less the net independent collateral amount applicable to such derivative contracts; or

(C) Zero.

(ii) Netting sets not subject to a variation margin agreement under which the counterparty must post variation margin. The replacement cost of a netting set that is not subject to a variation margin agreement under which the counterparty must post variation margin to the FDIC-supervised institution is the greater of:

(A) The sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set less the net independent collateral amount and variation margin amount applicable to such derivative contracts; or

(B) Zero.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(i) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(6)(i) and (ii) of this section, the replacement cost for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(i) of this section.

(7) Potential future exposure of a netting set. The potential future exposure of a netting set is the product of the PFE multiplier and the aggregated amount.

(i) PFE multiplier. The PFE multiplier is calculated according to the following formula:

Where:

V is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set;

C is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting set; and

A is the aggregated amount of the netting set.

(ii) Aggregated amount. The aggregated amount is the sum of all hedging set amounts, as calculated under paragraph (c)(8) of this section, within a netting set.

(iii) Multiple netting sets subject to a single variation margin agreement. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under § 324.10(c)(4)(ii)(B), the potential future exposure for multiple netting sets subject to a single variation margin agreement must be calculated according to paragraph (c)(10)(ii) of this section.

(iv) Multiple netting sets subject to multiple variation margin agreements or a hybrid netting set. Notwithstanding paragraphs (c)(7)(i) and (ii) of this section and when calculating the PFE amount for purposes of total leverage exposure under section 324.10(c)(4)(ii)(B), the potential future exposure for a netting set subject to multiple variation margin agreements or a hybrid netting set must be calculated according to paragraph (c)(11)(ii) of this section.

(8) Hedging set amount—(i) Interest rate derivative contracts. To calculate the hedging set amount of an interest rate derivative contract hedging set, a FDIC-supervised institution may use either of the formulas provided in paragraphs (c)(8)(i)(A) and (B) of this section:

(ii) Exchange rate derivative contracts. For an exchange rate derivative contract hedging set, the hedging set amount equals the absolute value of the sum of the adjusted derivative contract amounts, as calculated under paragraph (c)(9) of this section, within the hedging set.

(iii) Credit derivative contracts and equity derivative contracts. The hedging set amount of a credit derivative contract hedging set or equity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each reference entity within the hedging set.

K is the number of reference entities within the hedging set.

AddOn (Ref_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference reference entity k; and

ρ_k equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(iv) Commodity derivative contracts. The hedging set amount of a commodity derivative contract hedging set within a netting set is calculated according to the following formula:

Where:

k is each commodity type within the hedging set.

K is the number of commodity types within the hedging set.

AddOn (Type_k) equals the sum of the adjusted derivative contract amounts, as determined under paragraph (c)(9) of this section, for all derivative contracts within the hedging set that reference commodity type k.

ρ equals the applicable supervisory correlation factor, as provided in Table 2 to this section.

(v) Basis derivative contracts and volatility derivative contracts. Notwithstanding paragraphs (c)(8)(i) through (iv) of this section, a FDIC-supervised institution must calculate a separate hedging set amount for each basis derivative contract hedging set and each volatility derivative contract hedging set. A FDIC-supervised institution must calculate such hedging set amounts using one of the formulas under paragraphs (c)(8)(i) through (iv) that corresponds to the primary risk factor of the hedging set being calculated.

(9) Adjusted derivative contract amount—(i) Summary. To calculate the adjusted derivative contract amount of a derivative contract, a FDIC-supervised institution must determine the adjusted notional amount of derivative contract, pursuant to paragraph (c)(9)(ii) of this section, and multiply the adjusted notional amount by each of the supervisory delta adjustment, pursuant to paragraph (c)(9)(iii) of this section, the maturity factor, pursuant to paragraph (c)(9)(iv) of this section, and the applicable supervisory factor, as provided in Table 2 to this section.

(ii) Adjusted notional amount. (A)(1) For an interest rate derivative contract or a credit derivative contract, the adjusted notional amount equals the product of the notional amount of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation, and the supervisory duration, as calculated by the following formula:

Where:

S is the number of business days from the present day until the start date of the derivative contract, or zero if the start date has already passed; and

E is the number of business days from the present day until the end date of the derivative contract.

(2) For purposes of paragraph (c)(9)(ii)(A)(1) of this section:

(i) For an interest rate derivative contract or credit derivative contract that is a variable notional swap, the notional amount is equal to the time-weighted average of the contractual notional amounts of such a swap over the remaining life of the swap; and

(ii) For an interest rate derivative contract or a credit derivative contract that is a leveraged swap, in which the notional amount of all legs of the derivative contract are divided by a factor and all rates of the derivative contract are multiplied by the same factor, the notional amount is equal to the notional amount of an equivalent unleveraged swap.

(B)(1) For an exchange rate derivative contract, the adjusted notional amount is the notional amount of the non-U.S. denominated currency leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation. If both legs of the exchange rate derivative contract are denominated in currencies other than U.S. dollars, the adjusted notional amount of the derivative contract is the largest leg of the derivative contract, as measured in U.S. dollars using the exchange rate on the date of the calculation.

(2) Notwithstanding paragraph (c)(9)(i)(B)(1) of this section, for an exchange rate derivative contract with multiple exchanges of principal, the FDIC-supervised institution must set the adjusted notional amount of the derivative contract equal to the notional amount of the derivative contract multiplied by the number of exchanges of principal under the derivative contract.

(C)(1) For an equity derivative contract or a commodity derivative contract, the adjusted notional amount is the product of the fair value of one unit of the reference instrument underlying the derivative contract and the number of such units referenced by the derivative contract.

(2) Notwithstanding paragraph (c)(9)(i)(C)(1) of this section, when calculating the adjusted notional amount for an equity derivative contract or a commodity derivative contract that is a volatility derivative contract, the FDIC-supervised institution must replace the unit price with the underlying volatility referenced by the volatility derivative contract and replace the number of units with the notional amount of the volatility derivative contract.

(iii) Supervisory delta adjustments. (A) For a derivative contract that is not an option contract or collateralized debt obligation tranche, the supervisory delta adjustment is 1 if the fair value of the derivative contract increases when the value of the primary risk factor increases and −1 if the fair value of the derivative contract decreases when the value of the primary risk factor increases;

(B)(1) For a derivative contract that is an option contract, the supervisory delta adjustment is determined by the following formulas, as applicable:

(2) As used in the formulas in Table 3 to this section:

(i) φ is the standard normal cumulative distribution function;

(ii) P equals the current fair value of the instrument or risk factor, as applicable, underlying the option;

(iii) K equals the strike price of the option;

(iv) T equals the number of business days until the latest contractual exercise date of the option;

(v) l equals zero for all derivative contracts except interest rate options for the currencies where interest rates have negative values. The same value of l must be used for all interest rate options that are denominated in the same currency. To determine the value of l for a given currency, a FDIC-supervised institution must find the lowest value L of P and K of all interest rate options in a given currency that the FDIC-supervised institution has with all counterparties. Then, l is set according to this formula: λ = max{−L + 0.1%, 0}; and

(vi) σ equals the supervisory option volatility, as provided in Table 2 to this section; and

(C)(1) For a derivative contract that is a collateralized debt obligation tranche, the supervisory delta adjustment is determined by the following formula:

(2) As used in the formula in paragraph (c)(9)(iii)(C)(1) of this section:

(i) A is the attachment point, which equals the ratio of the notional amounts of all underlying exposures that are subordinated to the FDIC-supervised institution's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; ^[30]

(ii) D is the detachment point, which equals one minus the ratio of the notional amounts of all underlying exposures that are senior to the FDIC-supervised institution's exposure to the total notional amount of all underlying exposures, expressed as a decimal value between zero and one; and

(iii) The resulting amount is designated with a positive sign if the collateralized debt obligation tranche was purchased by the FDIC-supervised institution and is designated with a negative sign if the collateralized debt obligation tranche was sold by the FDIC-supervised institution.

(iv) Maturity factor. (A)(1) The maturity factor of a derivative contract that is subject to a variation margin agreement, excluding derivative contracts that are subject to a variation margin agreement under which the counterparty is not required to post variation margin, is determined by the following formula:

Where MPOR refers to the period from the most recent exchange of collateral covering a netting set of derivative contracts with a defaulting counterparty until the derivative contracts are closed out and the resulting market risk is re-hedged.

(2) Notwithstanding paragraph (c)(9)(iv)(A)(1) of this section:

(i) For a derivative contract that is not a cleared transaction, MPOR cannot be less than ten business days plus the periodicity of re-margining expressed in business days minus one business day;

(ii) For a derivative contract that is a cleared transaction, MPOR cannot be less than five business days plus the periodicity of re-margining expressed in business days minus one business day; and

(iii) For a derivative contract that is within a netting set that is composed of more than 5,000 derivative contracts that are not cleared transactions, MPOR cannot be less than twenty business days.

(3) Notwithstanding paragraphs (c)(9)(iv)(A)(1) and (2) of this section, for a derivative contract subject to an outstanding dispute over variation margin, the applicable floor is twice the amount provided in (c)(9)(iv)(A)(1) and (2) of this section.

(B) The maturity factor of a derivative contract that is not subject to a variation margin agreement, or derivative contracts under which the counterparty is not required to post variation margin, is determined by the following formula:

Where M equals the greater of 10 business days and the remaining maturity of the contract, as measured in business days.

(C) For purposes of paragraph (c)(9)(iv) of this section, derivative contracts with daily settlement are treated as derivative contracts not subject to a variation margin agreement and daily settlement does not change the end date of the period referenced by the derivative contract.

(v) Derivative contract as multiple effective derivative contracts. A FDIC-supervised institution must separate a derivative contract into separate derivative contracts, according to the following rules:

(A) For an option where the counterparty pays a predetermined amount if the value of the underlying asset is above or below the strike price and nothing otherwise (binary option), the option must be treated as two separate options. For purposes of paragraph (c)(9)(iii)(B) of this section, a binary option with strike K must be represented as the combination of one bought European option and one sold European option of the same type as the original option (put or call) with the strikes set equal to 0.95*K and 1.05*K so that the payoff of the binary option is reproduced exactly outside the region between the two strikes. The absolute value of the sum of the adjusted derivative contract amounts of the bought and sold options is capped at the payoff amount of the binary option.

(B) For a derivative contract that can be represented as a combination of standard option payoffs (such as collar, butterfly spread, calendar spread, straddle, and strangle), each standard option component must be treated as a separate derivative contract.

(C) For a derivative contract that includes multiple-payment options, (such as interest rate caps and floors) each payment option may be represented as a combination of effective single-payment options (such as interest rate caplets and floorlets).

(10) Multiple netting sets subject to a single variation margin agreement—(i) Calculating replacement cost. Notwithstanding paragraph (c)(6) of this section, a FDIC-supervised institution shall assign a single replacement cost to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin, calculated according to the following formula:

Replacement Cost = max{Σ_NSmax{V_NS; 0} − max{C_MA; 0}; 0} + max{Σ_NSmin{V_NS; 0} − min{C_MA; 0}; 0}

Where:

NS is each netting set subject to the variation margin agreement MA;

V _NS is the sum of the fair values (after excluding any valuation adjustments) of the derivative contracts within the netting set NS;

C _MA is the sum of the net independent collateral amount and the variation margin amount applicable to the derivative contracts within the netting sets subject to the single variation margin agreement.

(ii) Calculating potential future exposure. Notwithstanding paragraph (c)(5) of this section, a FDIC-supervised institution shall assign a single potential future exposure to multiple netting sets that are subject to a single variation margin agreement under which the counterparty must post variation margin equal to the sum of the potential future exposure of each such netting set, each calculated according to paragraph (c)(7) of this section as if such nettings sets were not subject to a variation margin agreement.

(11) Netting set subject to multiple variation margin agreements or a hybrid netting set—(i) Calculating replacement cost. To calculate replacement cost for either a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, the calculation for replacement cost is provided under paragraph (c)(6)(ii) of this section, except that the variation margin threshold equals the sum of the variation margin thresholds of all variation margin agreements within the netting set and the minimum transfer amount equals the sum of the minimum transfer amounts of all the variation margin agreements within the netting set.

(ii) Calculating potential future exposure. (A) To calculate potential future exposure for a netting set subject to multiple variation margin agreements under which the counterparty to each variation margin agreement must post variation margin, or a netting set composed of at least one derivative contract subject to variation margin agreement under which the counterparty to the derivative contract must post variation margin and at least one derivative contract that is not subject to such a variation margin agreement, a FDIC-supervised institution must divide the netting set into sub-netting sets and calculate the aggregated amount for each sub-netting set. The aggregated amount for the netting set is calculated as the sum of the aggregated amounts for the sub-netting sets. The multiplier is calculated for the entire netting set.

(B) For purposes of paragraph (c)(11)(ii)(A) of this section, the netting set must be divided into sub-netting sets as follows:

(1) All derivative contracts within the netting set that are not subject to a variation margin agreement or that are subject to a variation margin agreement under which the counterparty is not required to post variation margin form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is not subject to a variation margin agreement.

(2) All derivative contracts within the netting set that are subject to variation margin agreements in which the counterparty must post variation margin and that share the same value of the MPOR form a single sub-netting set. The aggregated amount for this sub-netting set is calculated as if the netting set is subject to a variation margin agreement, using the MPOR value shared by the derivative contracts within the netting set.

(12) Treatment of cleared transactions. (i) A FDIC-supervised institution must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more cleared transactions.

(ii) A FDIC-supervised institution that is a clearing member must apply the adjustments in paragraph (c)(12)(iii) of this section to the calculation of exposure amount under this paragraph (c) for a netting set that is composed solely of one or more exposures, each of which are exposures of the FDIC-supervised institution to its clearing member client where the FDIC-supervised institution is either acting as a financial intermediary and enters into an offsetting transaction with a CCP or where the FDIC-supervised institution provides a guarantee to the CCP on the performance of the client.

(iii)(A) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, MPOR may not be less than 10 business days;

(B) For purposes of calculating the maturity factor under paragraph (c)(9)(iv)(B) of this section, the minimum MPOR under paragraph (c)(9)(iv)(A)(3) of this section does not apply if there are no outstanding disputed trades in the netting set, there is no illiquid collateral in the netting set, and there are no exotic derivative contracts in the netting set; and

(C) For purposes of calculating the maturity factor under paragraphs (c)(9)(iv)(A) and (B) of this section, if the CCP collects and holds variation margin and the variation margin is not bankruptcy remote from the CCP, M_i may not exceed 250 business days.

Table 2 to § 324.132—Supervisory Option Volatility, Supervisory Correlation Parameters, and Supervisory Factors for Derivative Contracts

Asset class	Subclass	Supervisory option volatility (%)	Supervisory correlation factor (%)	Supervisory factor 1 (%)
Interest rate	N/A	50	N/A	0.50
Exchange rate	N/A	15	N/A	4.0
Credit, single name	Investment grade	100	50	0.5
	Speculative grade	100	50	1.3
	Sub-speculative grade	100	50	6.0
Credit, index	Investment Grade	80	80	0.38
	Speculative Grade	80	80	1.06
Equity, single name	N/A	120	50	32
Equity, index	N/A	75	80	20
Commodity	Energy	150	40	40
	Metals	70	40	18
	Agricultural	70	40	18
	Other	70	40	18
1 The applicable supervisory factor for basis derivative contract hedging sets is equal to one-half of the supervisory factor provided in this Table 2, and the applicable supervisory factor for volatility derivative contract hedging sets is equal to 5 times the supervisory factor provided in this Table 2.

* * * * *

34. Section 324.133 amended by revising paragraphs (a), (b)(1) through (3), (b)(4)(i), (c)(1) through (3), (c)(4)(i), and (d) to read as follows:

§ 324.133

Cleared transactions.

(a) General requirements—(1) Clearing member clients. A FDIC-supervised institution that is a clearing member client must use the methodologies described in paragraph (b) of this section to calculate risk-weighted assets for a cleared transaction.

(2) Clearing members. A FDIC-supervised institution that is a clearing member must use the methodologies described in paragraph (c) of this section to calculate its risk-weighted assets for a cleared transaction and paragraph (d) of this section to calculate its risk-weighted assets for its default fund contribution to a CCP.

(b) * * *

(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a FDIC-supervised institution that is a clearing member client must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (b)(2) of this section, by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (b)(3) of this section.

(ii) A clearing member client FDIC-supervised institution's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. (i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD for the derivative contract or netting set of derivative contracts calculated using the methodology used to calculate EAD for derivative contracts set forth in § 324.132(c) or (d), plus the fair value of the collateral posted by the clearing member client FDIC-supervised institution and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the FDIC-supervised institution calculates EAD for the cleared transaction using the methodology in § 324.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD for the repo-style transaction calculated using the methodology set forth in § 324.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member client FDIC-supervised institution and held by the CCP or a clearing member in a manner that is not bankruptcy remote. When the FDIC-supervised institution calculates EAD for the cleared transaction under § 324.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) For a cleared transaction with a QCCP, a clearing member client FDIC-supervised institution must apply a risk weight of:

(A) 2 percent if the collateral posted by the FDIC-supervised institution to the QCCP or clearing member is subject to an arrangement that prevents any loss to the clearing member client FDIC-supervised institution due to the joint default or a concurrent insolvency, liquidation, or receivership proceeding of the clearing member and any other clearing member clients of the clearing member; and the clearing member client FDIC-supervised institution has conducted sufficient legal review to conclude with a well-founded basis (and maintains sufficient written documentation of that legal review) that in the event of a legal challenge (including one resulting from an event of default or from liquidation, insolvency or receivership proceedings) the relevant court and administrative authorities would find the arrangements to be legal, valid, binding and enforceable under the law of the relevant jurisdictions.

(B) 4 percent, if the requirements of paragraph (b)(3)(i)(A) of this section are not met.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member client FDIC-supervised institution must apply the risk weight applicable to the CCP under § 324.32.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client FDIC-supervised institution that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(c) * * *

(1) Risk-weighted assets for cleared transactions. (i) To determine the risk-weighted asset amount for a cleared transaction, a clearing member FDIC-supervised institution must multiply the trade exposure amount for the cleared transaction, calculated in accordance with paragraph (c)(2) of this section by the risk weight appropriate for the cleared transaction, determined in accordance with paragraph (c)(3) of this section.

(ii) A clearing member FDIC-supervised institution's total risk-weighted assets for cleared transactions is the sum of the risk-weighted asset amounts for all of its cleared transactions.

(2) Trade exposure amount. A clearing member FDIC-supervised institution must calculate its trade exposure amount for a cleared transaction as follows:

(i) For a cleared transaction that is a derivative contract or a netting set of derivative contracts, trade exposure amount equals the EAD calculated using the methodology used to calculate EAD for derivative contracts set forth in § 324.132(c) or (d), plus the fair value of the collateral posted by the clearing member FDIC-supervised institution and held by the CCP in a manner that is not bankruptcy remote. When the clearing member FDIC-supervised institution calculates EAD for the cleared transaction using the methodology in § 324.132(d), EAD equals EAD_unstressed.

(ii) For a cleared transaction that is a repo-style transaction or netting set of repo-style transactions, trade exposure amount equals the EAD calculated under § 324.132(b)(2) or (3) or (d), plus the fair value of the collateral posted by the clearing member FDIC-supervised institution and held by the CCP in a manner that is not bankruptcy remote. When the clearing member FDIC-supervised institution calculates EAD for the cleared transaction under § 324.132(d), EAD equals EAD_unstressed.

(3) Cleared transaction risk weights. (i) A clearing member FDIC-supervised institution must apply a risk weight of 2 percent to the trade exposure amount for a cleared transaction with a QCCP.

(ii) For a cleared transaction with a CCP that is not a QCCP, a clearing member FDIC-supervised institution must apply the risk weight applicable to the CCP according to § 324.32.

(iii) Notwithstanding paragraphs (c)(3)(i) and (ii) of this section, a clearing member FDIC-supervised institution may apply a risk weight of zero percent to the trade exposure amount for a cleared transaction with a QCCP where the clearing member FDIC-supervised institution is acting as a financial intermediary on behalf of a clearing member client, the transaction offsets another transaction that satisfies the requirements set forth in § 324.3(a), and the clearing member FDIC-supervised institution is not obligated to reimburse the clearing member client in the event of the QCCP default.

(4) * * *

(i) Notwithstanding any other requirement of this section, collateral posted by a clearing member client FDIC-supervised institution that is held by a custodian (in its capacity as a custodian) in a manner that is bankruptcy remote from the CCP, clearing member, and other clearing member clients of the clearing member, is not subject to a capital requirement under this section.

* * * * *

(d) Default fund contributions—(1) General requirement. A clearing member FDIC-supervised institution must determine the risk-weighted asset amount for a default fund contribution to a CCP at least quarterly, or more frequently if, in the opinion of the FDIC-supervised institution or the FDIC, there is a material change in the financial condition of the CCP.

(2) Risk-weighted asset amount for default fund contributions to nonqualifying CCPs. A clearing member FDIC-supervised institution's risk-weighted asset amount for default fund contributions to CCPs that are not QCCPs equals the sum of such default fund contributions multiplied by 1,250 percent, or an amount determined by the FDIC, based on factors such as size, structure and membership characteristics of the CCP and riskiness of its transactions, in cases where such default fund contributions may be unlimited.

(3) Risk-weighted asset amount for default fund contributions to QCCPs. A clearing member FDIC-supervised institution's risk-weighted asset amount for default fund contributions to QCCPs equals the sum of its capital requirement, K_CM for each QCCP, as calculated under the methodology set forth in paragraph (e)(4) of this section.

(i) EAD must be calculated separately for each clearing member's sub-client accounts and sub-house account (i.e., for the clearing member's propriety activities). If the clearing member's collateral and its client's collateral are held in the same default fund contribution account, then the EAD of that account is the sum of the EAD for the client-related transactions within the account and the EAD of the house-related transactions within the account. For purposes of determining such EADs, the independent collateral of the clearing member and its client must be allocated in proportion to the respective total amount of independent collateral posted by the clearing member to the QCCP.

(ii) If any account or sub-account contains both derivative contracts and repo-style transactions, the EAD of that account is the sum of the EAD for the derivative contracts within the account and the EAD of the repo-style transactions within the account. If independent collateral is held for an account containing both derivative contracts and repo-style transactions, then such collateral must be allocated to the derivative contracts and repo-style transactions in proportion to the respective product specific exposure amounts, calculated, excluding the effects of collateral, according to § 324.132(b) for repo-style transactions and to § 324.132(c)(5) for derivative contracts.

(4) Risk-weighted asset amount for default fund contributions to a QCCP. A clearing member FDIC-supervised institution's capital requirement for its default fund contribution to a QCCP (K_CM) is equal to:

(5) Hypothetical capital requirement of a QCCP. Where a QCCP has provided its K_CCP, a FDIC-supervised institution must rely on such disclosed figure instead of calculating K_CCP under this paragraph (d)(5), unless the FDIC-supervised institution determines that a more conservative figure is appropriate based on the nature, structure, or characteristics of the QCCP. The hypothetical capital requirement of a QCCP (K_CCP), as determined by the FDIC-supervised institution, is equal to:

K_CCP = Σ_CMi EAD_i * 1.6 percent

Where:

CM_i is each clearing member of the QCCP; and

EAD_i is the exposure amount of each clearing member of the QCCP to the QCCP, as determined under paragraph (d)(6) of this section.

(6) EAD of a clearing member FDIC-supervised institution to a QCCP. (i) The EAD of a clearing member FDIC-supervised institution to a QCCP is equal to the sum of the EAD for derivative contracts determined under paragraph (d)(6)(ii) of this section and the EAD for repo-style transactions determined under paragraph (d)(6)(iii) of this section.

(ii) With respect to any derivative contracts between the FDIC-supervised institution and the CCP that are cleared transactions and any guarantees that the FDIC-supervised institution has provided to the CCP with respect to performance of a clearing member client on a derivative contract, the EAD is equal to the sum of:

(A) The exposure amount for all such derivative contracts and guarantees of derivative contracts calculated under SA-CCR in § 324.132(c) using a value of 10 business days for purposes of § 324.132(c)(9)(iv)(B);

(B) The value of all collateral held by the CCP posted by the clearing member FDIC-supervised institution or a clearing member client of the FDIC-supervised institution in connection with a derivative contract for which the FDIC-supervised institution has provided a guarantee to the CCP; and

(C) The amount of the prefunded default fund contribution of the FDIC-supervised institution to the CCP.

(iii) With respect to any repo-style transactions between the FDIC-supervised institution and the CCP that are cleared transactions, EAD is equal to:

EAD = max{EBRM−IM−DF; 0}

Where:

EBRM is the sum of the exposure amounts of each repo-style transaction between the FDIC-supervised institution and the CCP as determined under § 324.132(b)(2) and without recognition of any collateral securing the repo-style transactions;

IM is the initial margin collateral posted by the FDIC-supervised institution to the CCP with respect to the repo-style transactions; and

DF is the prefunded default fund contribution of the FDIC-supervised institution to the CCP.

35. Section 324.300 is amended by adding paragraph (f) to read as follows:

§ 324.300

Transitions.

* * * * *

(f) SA-CCR. After giving prior notice to the FDIC, an advanced approaches FDIC-supervised institution may use CEM rather than SA-CCR to determine the exposure amount for purposes of § 324.34 and the EAD for purposes of § 324.132 for its derivative contracts until July 1, 2020. On July 1, 2020, and thereafter, an advanced approaches FDIC-supervised institution must use SA-CCR for purposes of § 324.34 and must use either SA-CCR or IMM for purposes of § 324.132. Once an advanced approaches FDIC-supervised institution has begun to use SA-CCR, the advanced approaches FDIC-supervised institution may not change to use CEM.

Dated: November 7, 2018.

Joseph M. Otting,

Comptroller of the Currency.

By order of the Board of Governors of the Federal Reserve System, November 6, 2018.

Ann E. Misback,

Secretary of the Board.

Dated at Washington, DC, on October 17, 2018.

By order of the Board of Directors.

Federal Deposit Insurance Corporation.

Robert E. Feldman,

Executive Secretary.

Footnotes

1.  See 12 CFR part 3 (OCC); 12 CFR part 217 (Board); 12 CFR part 324 (FDIC). The agencies have codified the capital rule in different parts of title 12 of the CFR (part 3 (OCC); part 217 (Board); and part 324 (FDIC)), but the internal structure of the sections within each agency's rule are identical. All references to sections in the capital rule or the proposal are intended to refer to the corresponding sections in the capital rule of each agency.

2.  Banking organizations subject to the agencies' capital rule include national banks, state member banks, insured state nonmember banks, savings associations, and top-tier bank holding companies and savings and loan holding companies domiciled in the United States, but exclude banking organizations subject to the Board's Small Bank Holding Company Policy Statement (12 CFR part 225, appendix C), and certain savings and loan holding companies that are substantially engaged in insurance underwriting or commercial activities or that are estate trusts, and bank holding companies and savings and loan holding companies that are employee stock ownership plans.

3.  A banking organization is an advanced approaches banking organization if it has at least $250 billion in total consolidated assets or if it has consolidated on-balance sheet foreign exposures of at least $10 billion, or if it is a subsidiary of a depository institution, bank holding company, savings and loan holding company or intermediate holding company that is an advanced approaches banking organization. See 12 CFR 3.100(b) (OCC); 12 CFR 217.100(b) (Board); and 12 CFR 324.100(b) (FDIC).

4.  12 CFR 3.10(c) (OCC); 12 CFR 217.10(c) (Board); and 12 CFR 324.10(c) (FDIC). For example, an advanced approaches banking organization's tier 1 capital ratio is the lower of the ratio of the banking organization's common equity tier 1 capital to standardized total risk-weighted assets and the ratio of the banking organization's common equity tier 1 capital to advanced approaches total risk-weighted assets.

5.  See generally 12 CFR 3.132 (OCC); 12 CFR 217.132 (Board); and 12 CFR 324.132 (FDIC).

6.  83 FR 38460 (August 6, 2018).

7.  Many of the Board's other regulations rely on amounts determined under the capital rule, and the introduction of SA-CCR therefore could indirectly effect all such rules.

8.  See 81 FR 35124 (June 1, 2016).

9.  See, e.g., Swap Margin Rule, 12 CFR part 45 (OCC); 12 CFR part 237 (Board); 12 CFR part 349 (FDIC).

10.  See 12 CFR 3.34 (OCC); 12 CFR 217.34 (Board); 12 CFR 324.34 (FDIC).

11.  12 CFR 3.34(a)(1)(i) (OCC); 12 CFR 217.34(a)(1)(i) (Board); 12 CFR 324.34(a)(1)(i) (FDIC).

12.  12 CFR 3.34(a)(1)(ii) (OCC); 12 CFR 217.34(a)(1)(ii) (Board); 12 CFR 324.34(a)(1)(ii) (FDIC).

13.  12 CFR 3.34, Table 1 to § 3.34 (OCC); 12 CFR 217.34, Table 1 to § 217.34 (Board); 12 CFR 324.34, Table 1 to § 324.34 (FDIC). The derivative contract types are interest rate, exchange rate, investment grade credit, non-investment grade credit, equity, gold, precious metals except gold, and other. The maturities are one year or less, greater than one year and less than or equal to five years, and greater than five years.

14.  See 12 CFR 3.2 (OCC); 12 CFR 217.2 (Board); and 12 CFR 324.2 (FDIC). In 2017, the agencies adopted a final rule that requires U.S. global systemically important banking institutions (GSIBs) and the U.S. operations of foreign GSIBs to amend their qualified financial contracts to prevent their immediate cancellation or termination if such a firm enters bankruptcy or a resolution process. Qualified financial contracts include derivative contracts, securities lending, and short-term funding transactions such as repurchase agreements. The 2017 rulemaking would have invalidated the ability of derivative contracts to be subject to a QMNA. Therefore, as part of the 2017 rulemaking, the agencies revised the definition of QMNA under the capital rule such that qualified financial contracts could be subject to a QMNA (notwithstanding other operational requirements). See 82 FR 42882 (September 2017).

15.  See Definition of “qualifying master netting agreement,” 12 CFR 3.3 (OCC); 12 CFR 217.3 (Board); and 12 CFR 324.3 (FDIC).

16.  12 CFR 3.34(a)(2) (OCC); 12 CFR 217.34(a)(2) (Board); 12 CFR 324.34(a)(2) (FDIC).

17.  12 CFR 3.34(a)(2)(i) (OCC); 12 CFR 217.34(a)(2)(i) (Board); 12 CFR 324.34(a)(2)(i) (FDIC).

18.  12 CFR 3.34(a)(2)(ii) (OCC); 12 CFR 217.34(a)(2)(ii) (Board); 12 CFR 324.34(a)(2)(ii) (FDIC).

19.  12 CFR 3.34(b) (referencing 12 CFR 3.37) (OCC); 12 CFR 217.34(b) (referencing 12 CFR 217.37) (Board); 12 CFR 324.34(b) (referencing 12 CFR 324.37) (FDIC).

20.  A banking organization arrives at the exposure amount by first determining the EE profile for each netting set. In general, EE profile is determined by computing exposure distributions over a set of future dates using Monte Carlo simulations, and the expectation of exposure at each date is the simple average of all Monte Carlo simulations for each date. The expiration of short-term trades can cause the EE profile to decrease, even though a banking organization is likely to replace short-term trades with new trades (i.e., rollover). To account for rollover, a banking organization converts the EE profile for each netting set into an effective EE profile by applying a nondecreasing constraint to the corresponding EE profile over the first year. The nondecreasing constraint prevents the effective EE profile from declining with time by replacing the EE amount at a given future date with the maximum of the EE amounts across this and all prior simulation dates. The EEPE for a netting set is the time-weighted average of the effective EE profile over a one-year horizon. EEPE would be the appropriate loan equivalent exposure in a credit risk capital calculation if the following assumptions were true: There is no concentration risk, systematic market risk, and wrong-way risk (i.e., the size of an exposure is positively correlated with the counterparty's probability of default). However, these conditions nearly never exist with respect to a derivative contract. Thus, to account for these risks, IMM requires a banking organization to multiply EEPE by 1.4.

21.  The agencies initially adopted CEM in 1989. 54 FR 4168 (January 27, 1989) (OCC); 54 FR 4186 (January 27, 1989) (Board); 54 FR 11500 (March 21, 1989) (FDIC). The last significant update to CEM was in 1995. 60 FR 46170 (September 5, 1995).

22.  See supra n. 9.

23.  See 12 CFR 3.122 (OCC); 12 CFR 217.122 (Board); 12 CFR 324.122 (FDIC).

24.  “The standardized approach for measuring counterparty credit risk exposures,” Basel Committee on Banking Supervision, March 2014 (rev. April 2014), https://www.bis.org/​publ/​bcbs279.pdf. See “Foundations of the standardised approach for measuring counterparty credit risk exposures” (August 2014, rev. June 2017), https://www.bis.org/​publ/​bcbs_​wp26.pdf.

25.  For an unmargined netting set, IMM's EE profile starts at t=0, which is the date at which replacement cost under SA-CCR is calculated. For a deep in-the-money netting set, PFE would be much smaller than replacement cost, while IMM's EE profile would not increase significantly above replacement cost before declining (due to cash flow payments and trade expiration), because IMM volatilities typically are smaller than the volatilities implied by SA-CCR's PFE. The nondecreasing constraint would not allow the effective EE profile to drop below the replacement cost level, resulting in IMM's EEPE being slightly above replacement cost. Thus, both IMM's EEPE and SA-CCR's replacement cost plus PFE would be slightly above replacement cost and, therefore, close to each other.

26.  As described in section V of this preamble, the agencies are proposing to apply a five-day holding period to all derivative contracts that are cleared transactions, regardless whether the method the banking organization uses to calculate the exposure amount of the derivative contract.

27.  “Bankruptcy remote” is defined in § _.2 of the capital rule. See 12 CFR 3.2 (OCC); 12 CFR 217.2 (Board); and 12 CFR 324.2 (FDIC).

28.  There could be a situation unrelated to the value of the variation margin threshold in which the exposure amount of a margined netting set would be greater than the exposure amount of an equivalent unmargined netting set. For example, in the case of a margined netting set composed of short-term transactions with a residual maturity of 10 business days or less, the risk horizon would be the MPOR, which the proposal would floor at 10 business days. The risk horizon for an equivalent unmargined netting set also would be equal to 10 business days because this would be the floor for the remaining maturity of such a netting set. However, the maturity factor for the margined netting set would be greater than the one for the equivalent unmargined netting set because of the application of a factor of 1.5 to margined derivative contracts. In such an instance, the exposure amount of a margined netting set would be more than the exposure amount of an equivalent unmargined netting set by a factor of 1.5, thus triggering the cap. In addition, in the case of disputes, the MPOR of a margined netting set would be doubled, which could further increase the exposure amount of a margined netting set composed of short-term transactions with a residual maturity of 10 business days or less above an equivalent unmargined netting set. The agencies believe, however, that such instances rarely occur and thus would have minimal effect on banking organizations' regulatory capital.

29.  For the capital rule, the Board is the primary federal regulator for all bank and savings and loan holding companies, intermediate holding companies of foreign banks, and state member banks; the OCC is the primary federal regulator for all national banks and federal thrifts; and the FDIC is the primary federal regulatory for all state nonmember banks.

30.  See “Foundations of the standardised approach for measuring counterparty credit risk exposures.”

31.  The dependence between N random variables can be described by an NxN correlation matrix. In the most general case, such a correlation matrix requires estimation of N*(N-1)/2 individual correlation parameters. Estimating these correlations is problematic when N is large. Factor models are a popular means of reducing the number of independent correlation parameters by assuming that each random variable is driven by a combination of a small number of systematic factors (which are the same for all N random variables) and an idiosyncratic factor (which is unique to each random variable and is independent from all other factors). The simplest factor model is a single-factor model that assumes that a single systematic factor drives all N random variables.

32.  A higher correlation factor means that the underlying risk factors are more closely aligned. For a directional portfolio, more alignment between the risk factors would result in a more concentrated risk, leading to a higher exposure amount. For a balanced portfolio, more alignment between the risk factors would result in more offsetting of risk, leading to a lower exposure amount.

33.  For example, consider a variation margin agreement with a zero threshold amount that covers two netting sets, one with a market value of 100 and the other with a market value of negative 100. The aggregate market value of the netting sets would be zero and thus no variation margin would be exchanged. However, the banking organization's aggregate exposure amount for these netting sets would be equal to 100 because the negative market value of the second netting set would not be available to offset the positive market value of the first netting set. In the event of default of the counterparty, the banking organization would pay the counterparty 100 for the second netting set and would be exposed to a loss of 100 on the first netting set.

34.  For a derivative contract that can be represented as a combination of standard option payoffs (such as collar, butterfly spread, calendar spread, straddle, and strangle), each standard option component would be treated as a separate derivative contract. For a derivative contract that includes multiple-payment options, (such as interest rate caps and floors) each payment option could be represented as a combination of effective single-payment options (such as interest rate caplets and floorlets). Linear derivative contracts (such as swaps) would not be decomposed into components.

35.  Specifically, the supervisory factors are intended to reflect the EEPE of a single at-the-money linear trade of unit size, zero market value and one-year maturity referencing a given risk factor in the absence of collateral.

36.  Sensitivity of a derivative contract to a risk factor is the ratio of the change in the market value of the derivative contract caused by a small change in the risk factor to the value of the change in the risk factor. In a linear derivative contract, the payoff of the derivative contract moves at a constant rate with the change in the value of the underlying risk factor. In a nonlinear contract, the payoff of the derivative contract does not move at a constant rate with the change in the value of the underlying risk factor. The sensitivity is positive if the derivative contract is long the risk factor and negative if the derivative contract is short the risk factor.

37.  Specifically, the BCBS supervisory factors are as follow (in percent): AAA and AA—0.38, A—0.42; BBB—0.54; BB—1.06; B—1.6; CCC—6.0.

38.  Public Law 11-203, 124 Stat. 1376 (2010), § 939A. This provision is codified as part of the Securities Exchange Act of 1934 at 15 U.S.C. 78o-7.

39.  See 12 CFR 3.2 (OCC); 12 CFR 217.2 (Board); and 12 CFR 324.2 (FDIC).

40.  Markit North America, Inc., accessed via Wharton Research Data Services (WRDS), wrds-web.wharton.upenn.edu/wrds/about/databaselist.cfm.

41.  A banking organization would be required to represent binary options with strike K as the combination of one bought European option and one sold European option of the same type as the original option (put or call) with the strike prices set equal to 0.95 * K and 1.05 * K. The size of the position in the European options must be such that the payoff of the binary option is reproduced exactly outside the region between the two strikes. The absolute value of the sum of the adjusted derivative contract amounts of the bought and sold options is capped at the payoff amount of the binary option.

42.  The same value l_i of must be used for all interest rate options that are denominated in the same currency. The value of l_i for a given currency would be equal to the lowest value L of P_i and K_i of all interest rate options in a given currency that the banking organization has with all counterparties.

43.  In the case of a first-to-default credit derivative, there are no underlying exposures that are subordinated to the banking organization's exposure and A=0. In the case of a second-or-subsequent-to-default credit derivative, the smallest (n-1) notional amounts of the underlying exposures are subordinated to the banking organization's exposure.

44.  In general, a party will not have violated its obligation to collect or post variation margin from or to a counterparty if the counterparty has refused or otherwise failed to provide or accept the required variation margin to or from the party; and the party has made the necessary efforts to collect or post the required variation margin, including the timely initiation and continued pursuit of formal dispute resolution mechanisms; or has otherwise demonstrated that it has made appropriate efforts to collect or post the required variation margin; or commenced termination of the derivative contract with the counterparty promptly following the applicable cure period and notification requirements.

45.  See “Regulatory Capital Treatment of Certain Centrally-cleared Derivative Contracts Under Regulatory Capital Rules” (August 14, 2017), OCC Bulletin: 2017-27; FDIC Letter FIL-33-2017; and Board SR letter 07-17.

46.  This example is intended only for use as an illustrative guide. The calculation mechanics may vary based on a variety of factors, including for example, the number of hedging sets, the frequency at which variation margin is exchanged, and certain terms of the derivative contracts and underlying reference assets. SA-CCR considers a number of risk attributes to determine the exposure amount of a derivative contract, or netting set thereof, and not all of those attributes are captured in this example.

47.  See the definition of “qualifying central counterparty” in 12 CFR 3.2 (OCC); 12 CFR 217.2 (Board); and 12 CFR 324.2 (FDIC). The requirements are consistent with the principles developed by the Committee on Payment and Settlement Systems and Technical Committee of the International Organization of Securities Commissions. See “Principles for financial market infrastructure,” Committee on Payment and Settlement Systems and Technical Committee of the International Organization of Securities Commissions, (April 2012), available at https://www.bis.org/​cpmi/​publ/​d101a.pdf.

48.  “Capital requirements for bank exposures to central counterparties,” Basel Committee on Banking Supervision, April 2014, https://www.bis.org/​publ/​bcbs282.pdf.

49.  12 CFR 3.3 (OCC); 12 CFR 217.3 (Board); 12 CFR 324.3 (FDIC).

50.  The definition of default fund contribution includes fund commitments made by a clearing member to a CCP's mutualized loss sharing arrangements. The references to the commitments could include terms such as assessments, special assessments, guarantee commitments, and contingent capital commitments, among other terms.

51.  The agencies are not proposing to make revisions to the calculations to determine the exposure amount of repo-style transactions for purposes of determining the risk-weighted asset amount of a banking organization's default fund contributions.

52.  See 3.10(c)(4)(ii) (OCC); 12 CFR 217.10(c)(4)(ii) (Board); 324.10(c)(4)(ii) (FDIC).

53.  To determine the carrying value of derivative contracts, U.S. generally accepted accounting principles (GAAP) provide a banking organization with the option to reduce any positive fair value of a derivative contract by the amount of any cash collateral received from the counterparty, provided the relevant GAAP criteria for offsetting are met (the GAAP offset option). Similarly, under the GAAP offset option, a banking organization has the option to offset the negative mark-to-fair value of a derivative contract with a counterparty. See Accounting Standards Codification paragraphs 815-10-45-1 through 7 and 210-20-45-1. Under the capital rule, a banking organization that applies the GAAP offset option to determine the carrying value of its derivative contracts would be required to reverse the effect of the GAAP offset option for purposes of determining total leverage exposure, unless the collateral is cash variation margin recognized as settled with the derivative contract as a single unit of account for balance sheet presentation and satisfies the conditions under § _.10(c)(4)(ii)(C)(1)-(7) of the capital rule.

54.  Written options create an exposure to the derivative contact reference asset and thus must be included in total leverage exposure even though the proposal would allow certain written options to receive an exposure amount of zero for risk-based capital purposes.

55.  See 79 FR 57725, 57736 (Sept. 26, 2014).

56.  “Basel III: Finalising post-crisis reforms,” Basel Committee on Banking Supervision, December 2017, https://www.bis.org/​bcbs/​publ/​d424.pdf.

57.  Accordingly, a banking organization would not use § _.132(c)(7)(iii)-(iv) for purposes of calculating the PFE amount for the supplementary leverage ratio.

58.  See, e.g ., G-20 Pittsburgh Summit: Leaders Statement (September 2009); see also Consultative Document, “Leverage ratio treatment of client cleared derivatives,” Basel Committee on Banking Supervision, October 2018, https://www.bis.org/​bcbs/​publ/​d451.pdf.

59.  See Dodd-Frank Wall Street Reform and Consumer Protection Act, section 802(b).

60.  See 80 FR 41411 (July 15, 2015).

61.  See 12 CFR 3.35(b)(4) and 3.133(b)(4) (OCC); 12 CFR 217.35(b)(4) and 217.133(b)(4) (Board); 12 CFR 324.35(b)(4) and 324.133(b)(4) (FDIC).

62.  The agencies estimate that, on aggregate, exposure amounts under SA-CCR would equal approximately 170 percent of the exposure amounts for identical derivative contracts under IMM. Thus, firms that use IMM currently would likely continue to use IMM to determine the exposure amount of their derivative contracts to determine advanced approaches total risk-weighted assets. However, the standardized approach serves as a floor on advanced approaches banking organizations' total risk-weighted assets. Thus, a firm would only receive the benefit of IMM if the firm is not bound by standardized total risk-weighted assets.

63.  Total risk-weighted assets are a function of the exposure amount of the netting set and the applicable risk-weight of the counterparty. Total risk-weighted assets increase under the analysis while exposure amounts decrease because higher applicable risk-weights amplify increases in the exposure amount of certain derivative contracts, which outweighs decreases in the exposure amount of other derivative contracts.

64.  According to data from the Consolidated Reports of Condition and Income for a Bank with Domestic and Foreign Offices (FFIEC report forms 031, 041, and 051), as of March 31, 2018.

65.  5 U.S.C. 601 et seq.

66.  The SBA defines a small banking organization as having $550 million or less in assets, where an organization's “assets are determined by averaging the assets reported on its four quarterly financial statements for the preceding year.” See 13 CFR 121.201 (as amended, effective December 2, 2014). In its determination, the “SBA counts the receipts, employees, or other measure of size of the concern whose size is at issue and all of its domestic and foreign affiliates.” See 13 CFR 121.103. Following these regulations, the FDIC uses a covered entity's affiliated and acquired assets, averaged over the preceding four quarters, to determine whether the covered entity is “small” for the purposes of RFA.

67.  FDIC Call Report, March 31, 2018.

68.  Id.

69.  Under regulations issued by the Small Business Administration, a small entity includes a depository institution, bank holding company, or savings and loan holding company with total assets of $550 million or less and trust companies with total assets of $38.5 million or less. As of June 30, 2018, there were approximately 3,304 small bank holding companies, 216 small savings and loan holding companies, and [541] small state member banks.

70.  12 U.S.C. 3901-3911.

71.  12 U.S.C. 1831o.

72.  12 U.S.C. 3907(a)(1).

73.  12 U.S.C. 1831o(c)(2).

74.  See 12 U.S.C. 1467a, 1844, 5365, 5371.

75.  12 U.S.C. 4802(a).

76.  12 U.S.C. 4802.

30.  In the case of a first-to-default credit derivative, there are no underlying exposures that are subordinated to the national bank's or Federal savings association's exposure. In the case of a second-or-subsequent-to-default credit derivative, the smallest (n-1) notional amounts of the underlying exposures are subordinated to the national bank's or Federal savings association's exposure.

30.  In the case of a first-to-default credit derivative, there are no underlying exposures that are subordinated to the Board-regulated institution's exposure. In the case of a second-or-subsequent-to-default credit derivative, the smallest (n-1) notional amounts of the underlying exposures are subordinated to the Board-regulated institution's exposure.

30.  In the case of a first-to-default credit derivative, there are no underlying exposures that are subordinated to the FDIC-supervised institution's exposure. In the case of a second-or-subsequent-to-default credit derivative, the smallest (n-1) notional amounts of the underlying exposures are subordinated to the FDIC-supervised institution's exposure.