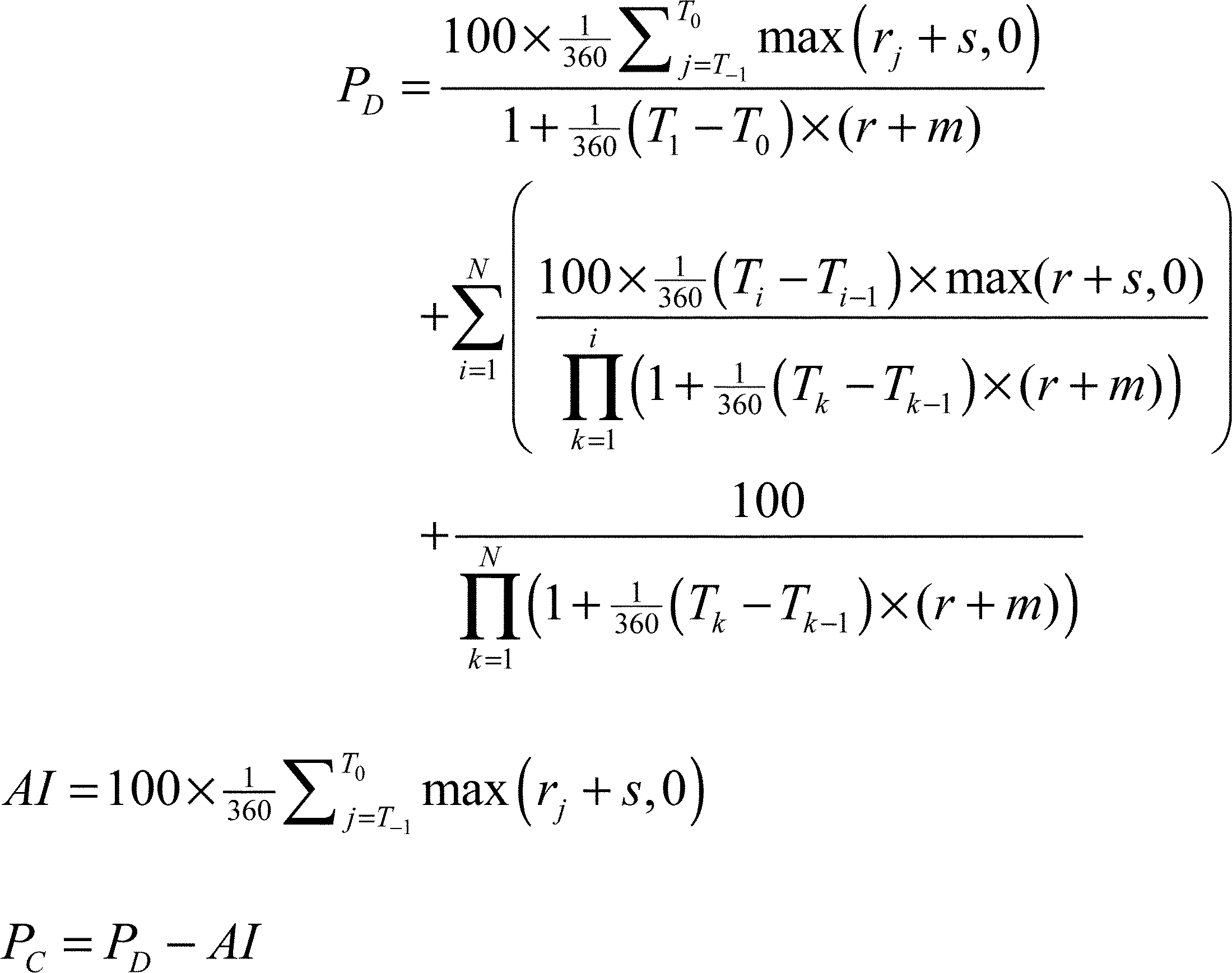2013-18178. Sale and Issue of Marketable Book-Entry Treasury Bills, Notes, and Bonds

AGENCY:

Fiscal Service, Treasury.

ACTION:

Final rule.

SUMMARY:

This final rule amends Treasury's marketable securities auction rules to accommodate the public offering of a new type of marketable security with a floating rate interest payment. In addition, the amendment makes certain technical clarifications and conforming changes.

DATES:

Effective July 31, 2013.

ADDRESSES:

Treasury has established a docket for this action under Docket ID Number Fiscal-BPD-2013-0001 in the www.regulations.gov Web site. This final rule is available for downloading from www.treasurydirect.gov. It is also available for public inspection and copying at the Treasury Library, 1500 Pennsylvania Avenue NW., Annex, Room 1020, Washington, DC 20220. To visit the library, call [phone] for an appointment.

FOR FURTHER INFORMATION CONTACT:

Lori Santamorena, Executive Director, or Chuck Andreatta, Associate Director, Government Securities Regulations Staff, Bureau of the Fiscal Service, Department of the Treasury, [phone].

SUPPLEMENTARY INFORMATION:

I. Background

The Department of the Treasury (“Treasury”) is issuing an amendment to 31 CFR part 356 ^[1] (the “Uniform Offering Circular”) to accommodate offerings of a new type of marketable security, referred to as a Treasury floating rate note, whose index rate will be indexed to 13-week Treasury bill auction rates. Treasury views issuance of floating rate notes as consistent with its mission to borrow at the lowest cost over time, manage the maturity profile of our marketable debt outstanding, expand the Treasury investor base, and provide a financing tool that gives debt managers additional flexibility. Treasury decided to establish a floating rate note program after carefully considering the long-term supply and demand dynamics for these securities and with significant consultation with market participants.

Treasury floating rate notes will be indexed to the most recent 13-week Treasury bill auction High Rate ^[2] (stop out rate), and converted to a simple-interest money market yield computed on an actual/360 basis, subject to an appropriate lockout period,^[3] which initially will be two business days (see appendix D). In its May 2013 Quarterly Refunding Statement, Treasury announced its intention to begin auctioning floating rate notes in either the fourth quarter of 2013 or the first quarter of 2014.^[4] Treasury's initial auction will be of two-year floating rate notes. Treasury will announce specific terms and conditions of each issue, such as the auction date, issue date, and public offering amount, prior to each auction. Over time, Treasury may consider offering additional maturities of floating rate notes.

II. Consultation and Request for Comments

Treasury announced at its February 2012 Quarterly Refunding that it was studying the possibility of issuing a floating rate note with an interest rate that is indexed and periodically reset.^[5] In determining the final terms and conditions for a floating rate note, Treasury sought input from a wide range of participants, particularly concerning the demand for the product, how the security should be structured, its liquidity, the most appropriate index, and operational issues that should be considered related to the issuance of this type of debt.

On March 19, 2012, Treasury issued a Notice and Request for Information (RFI) to the public with a closing date for comments of April 18, 2012.^[6] Treasury received 14 comment letters in response to the RFI.^[7] Commenters broadly supported issuance of this type of security. Based on the response to the RFI and additional feedback, Treasury announced in its August 2012 Quarterly Refunding Statement that it planned to develop a floating rate note program to complement the existing suite of securities issued and to support its broader debt management objectives.^[8]

On December 5, 2012, Treasury issued an Advance Notice of Proposed Rulemaking (ANPR) to invite public comment on the design details, terms and conditions, and other features relevant to the sale and issuance of this new type of security.^[9] The closing date for comments was January 22, 2013.

III. Comments Received in Response to the Advance Notice of Proposed Rulemaking

Treasury received 16 comment letters in response to the ANPR ^[10] —one from a securities industry trade association, eight from primary dealers, two from private citizens, and one each from a non-primary dealer, a derivatives clearing house, a derivatives exchange, an investment manager, and an advisory service. Overall, there was a consensus on many features of the security as proposed in the ANPR, including the reset frequency, frequency of interest payments, interest rate determination, initial maturity range, and auction technique. There was also an expressed belief that, if appropriately structured, a Treasury floating rate note would be an attractive investment for a broad base of institutional investors including money market funds, securities lenders, corporations, and foreign central banks.

Regarding the index rate, the ANPR specifically requested comments on the use of either (1) the 13-week Treasury bill auction High Rate (stop out rate) converted into a simple actual/360 interest rate, or (2) a Treasury general collateral overnight repurchase agreement rate (the “Treasury GC Rate”). All but one of the commenters addressed this issue, with nine favoring some form of repurchase agreement rate, and six preferring an index based on 13-week Treasury bills. Commenters preferring the Treasury bill index also preferred the actual/360 basis over any other method for converting the auction High Rate.

Most commenters preferred that the index rate be reset daily, and that interest payments be made quarterly. Commenters also widely supported having a new issue of floating rate notes every quarter with two subsequent monthly reopenings. Regarding the timing of settlement, a large majority who expressed a preference favored mid-month settlement over end-of-month settlement. There was also general consensus that the interest rate should be floored at zero percent.

In the ANPR, Treasury stated that it intends to start the floating rate note program with a two-year maturity. Most commenters agreed that this was a good maturity to start with, and suggested eventual expansion to longer maturities of up to 10 years.

Regarding the lockout periods, the ANPR noted that the current convention in the floating rate note market is for interest payments to be set five business days in advance of their payment dates. This standard practice dates from the late 1980s and was put in place for operational reasons. The ANPR stated that, given technological advancements, Treasury believes that one-business-day notice of interest payments should suffice. Four commenters stated that one business day was sufficient. One commenter stated that no lockout period was needed. Two commenters said that two business days was the most beneficial, while another commenter suggested two to three days “for maximum operational clarity.” One commenter advocated seven business days.

A commenter stated that, “at least initially, a two-day lockout period would be optimal for operational efficiency. The benefit of an initial two-day lockout period is that it would accommodate both the firms that are currently able to absorb a shorter lockout period in their current operational flow, as well as firms that would have to make operational adjustments. In addition, buyside members also indicated that a two-day lockout period would be optimal to achieve operational efficiency.”

IV. Summary of Terms, Conditions, and Features

After taking into consideration the comments received, Treasury is adopting as a final rule this amendment to the Uniform Offering Circular setting out the terms, conditions, and features of Treasury floating rate notes.

Floating rate notes will be issued with maturities of at least one year, but not more than ten years. Floating rate notes may be sold at discount, par, or premium, and will pay interest quarterly on the last calendar day of the month.

Auctions of Treasury floating rate notes will generally be conducted in the same manner as other marketable Treasury securities auctions. The auctions will be conducted as single-price auctions in which competitive bidders will bid in terms of a desired discount margin (positive, negative, or zero), expressed as a percentage with three decimals, e.g., 1.230 percent. The spread on the first issuance of a particular floating rate note will be set at the highest accepted discount margin in that auction. Auctions will include both competitive and noncompetitive bidding, a minimum purchase amount of $100, a maximum noncompetitive bid amount of $5 million, and a 35-percent maximum award limitation. The award methodology will be the same as for other Treasury marketable securities auctions.^[11]

Reopening auctions will be conducted in the same manner as new issuances, except that the spread on a floating rate note offered in a reopening auction will be the spread determined in the first auction of that security. Bidders in reopening auctions will bid on a discount margin basis and those who are awarded securities will be required to pay accrued interest from the dated date, or last interest payment date, to the reopening issue date.

The index for floating rate notes will be the weekly High Rate (stop out rate) of 13-week Treasury bill auctions. The interest rate will be the spread plus the index rate, which will reset daily based on the most recent auction of 13-week bills and will be subject to a minimum daily interest accrual rate of zero percent. After analyzing the comments received, Treasury determined that a minimum spread was unnecessary. The use of a zero-percent minimum daily interest accrual rate will prevent floating rate note investors from having to remit an interest payment to Treasury during unusual interest rate environments, including those with expectations for deeply negative interest rates.

Treasury carefully considered the ANPR responses related to the selection of an index rate. While a majority of respondents favored using a repurchase agreement rate, Treasury weighed that input against the benefits of indexing to the established, well-understood, and highly liquid 13-week Treasury bill market. At this time, Treasury believes that using the 13-week Treasury bill auction rate as the index will best achieve the goal of funding the government at the lowest possible cost over time. However, the selection of the 13-week Treasury bill auction rate as the index does not preclude Treasury from amending the Uniform Offering Circular in the future to provide for a floating rate note issuance that uses an alternative index.

Although the index rate will reset daily, given the current 13-week Treasury bill auction schedule, the rate will effectively change once a week. The index rate will change on the day following a 13-week bill auction regardless of whether that day is a business day or a non-business day.

Interest on floating rate notes will accrue daily throughout the interest payment period. In general, the interest accrual for a particular calendar day in an accrual period will be the spread determined at the time of a new floating rate note auction plus the index rate.

The index rate is computed from the most recent 13-week Treasury bill auction High Rate that has been translated into a simple-interest money market yield computed on an actual/360 basis and rounded to nine decimal places. If, however, the most recent 13-week bill auction occurred during a lockout period for the applicable floating rate note, then the index rate is computed from the most recent 13-week bill auction that occurred prior to the lockout period. As previously mentioned, the minimum daily interest accrual rate will be zero percent.

Treasury will provide notice of interest payments two business days prior to each interest payment date. For purposes of calculating auction settlement amounts and quarterly interest payments, floating rate notes will initially have a two-business-day lockout period prior to their auction settlement date or an interest payment date. Therefore, a 13-week Treasury bill auction that takes place during the lockout period will be excluded from the calculation of accrued interest for purposes of determining that settlement amount or interest payment. Any changes in the index rate that would otherwise have occurred during the lockout period will occur on the first calendar day following the end of the lockout period. We will provide sufficient notice if we change the length of the lockout period for future floating rate note issuances.

Although most commenters preferred mid-month settlement, the issue date for newly issued Treasury floating rate notes will normally be on the last calendar day of a month because this timing better accommodates Treasury's financing needs. Reopening issuances of floating rate notes will occur on the last Friday of a month. In both cases, if the regular issue day is a non-business day, issuance will occur on the next business day. The auction announcement for each floating rate note will contain the specific details of that offering.

Floating rate notes will not be eligible for stripping.^[12] The notes will be eligible, however, to serve as collateral for Treasury's Fiscal Service collateral programs.

This final rule makes the necessary revisions to accommodate the sale and issuance of floating rate notes. Accordingly, Treasury is amending sections 356.2; 356.5; 356.12; 356.14; 356.15; 356.20; 356.21; 356.23; 356.30, 356.31, 356.32; Appendix A, Section II; Appendix B, Sections I and IV; Appendix C, Section II; and Appendix D, Section II of 31 CFR 356.

V. Section by Section Summary

Section 356.2 has been amended by adding definitions of 13-week bill, Discount margin, Index rate, and Spread. The definition of Index has been amended to add that, in addition to the term meaning the Consumer Price Index for inflation protected securities, Index also means the High Rate on auctions of 13-week Treasury bills for floating rate notes. The definition of Interest rate has been expanded to define how the interest rate is determined for floating rate notes. Conforming changes have also been made to the definitions of Competitive bid, Multiple-price auction, Noncompetitive bid, Single-price auction, and Weighted-average to add discount margin as an allowable basis for bidding in addition to discount rate and yield.

Section 356.5 has been amended by adding a new paragraph (b)(3) to add floating rate notes as a new type of security that Treasury auctions. The footnote to this section has also been amended by changing the term “fixed-principal” to “non-indexed” to distinguish regular Treasury notes and bonds from inflation-protected securities and floating rate notes. The term “fixed-principal” has been changed to “non-indexed” throughout this entire part.

Section 356.12 has been amended by adding a new subparagraph (c)(1)(iv) to provide the competitive bidding format for floating rate notes.

Section 356.20 has been amended to create a new paragraph (c) that explains how interest rates for floating rate notes are determined.

Section 356.30 has been amended to allow for quarterly interest payments, since all other Treasury notes, bonds, and inflation-protected securities pay interest semiannually.

Section 356.31 has been amended to make it clear that floating rate notes are not eligible for stripping.

Section 356.32 has been amended by adding a new paragraph (c) to provide a brief mention of special federal income tax rules for floating rate notes.

Appendix B, Section I has been reorganized to add a new subsection C that describes the indexing and interest payment processes for floating rate notes, how the interest rate is determined, how interest accrues, and various floating rate index contingencies. New subsection D has been amended to add a new paragraph 6 that directs readers to section IV, paragraphs C and D of the appendix for discussion of how accrued interest is calculated for floating rate notes. A new Section IV has been added that provides the formulas for converting discount margins to equivalent prices for floating rate notes.

A new Section II has been added to Appendix C to address various investment considerations for Treasury floating rate notes. Specifically, Section II discusses interest variability, secondary market trading, tax considerations, and indexing issues.

Appendix D has been amended to revise the title, designate the current text as Section I, and add a new Section II that adds a description of the floating rate note index.

Conforming changes are also made to paragraphs 356.12(c)(2); 356.14(d); 356.15(e); 356.20(a)(1) and (a)(2) and new paragraphs (d)(1) and (d)(2); 356.21(a) and (b); 356.23(b)(2); and Appendix A, Section II, paragraph (d)(1) to add discount margin as an allowable basis for bidding.

VI. Procedural Requirements

Executive Order 12866. This final rule is not a “significant regulatory action” pursuant to Executive Order 12866.

Administrative Procedure Act (APA). Because this rule relates to public contracts and procedures for United States securities, the notice, public comment, and delayed effective date provisions of the Administrative Procedure Act are inapplicable, pursuant to 5 U.S.C. 553(a)(2).

Regulatory Flexibility Act. As no notice of proposed rulemaking is required, the provisions of the Regulatory Flexibility Act (5 U.S.C. 601, et seq.) do not apply.

Paperwork Reduction Act. There is no new collection of information contained in this final rule, and, therefore, the Paperwork Reduction Act does not apply. The Office of Management and Budget has approved the collections of information already contained in 31 CFR part 356, under control number 1535-0112. Under the Paperwork Reduction Act, an agency may not conduct or sponsor, and a person is not required to respond to, a collection of information unless it displays a valid OMB control number.

List of Subjects in 31 CFR Part 356

• Bonds
• Federal Reserve System
• Government Securities
• Securities

For the reasons set forth in the preamble, amend 31 CFR part 356 as follows:

PART 356—SALE AND ISSUE OF MARKETABLE BOOK-ENTRY TREASURY BILLS, NOTES, AND BONDS (DEPARTMENT OF THE TREASURY CIRCULAR, PUBLIC DEBT SERIES NO. 1-93)

1. The authority citation for part 356 continues to read as follows:

Authority: 5 U.S.C. 301; 31 U.S.C. 3102, et seq.; 12 U.S.C. 391.

2. In 31 CFR part 356, wherever it appears:

a. Remove `fixed-principal' and add in its place `non-indexed';

b. Remove `Fixed-principal' and add in its place `Non-indexed'; and

c. Remove `FIXED-PRINCIPAL' and add in its place `NON-INDEXED'.

Subpart A—General Information.

3. Amend § 356.2 by:

a. Adding definitions in alphabetical order for 13-week bill, Discount margin, Index rate, and Spread; and

b. Revising the definitions of Competitive bid, Index, Multiple-price auction, Noncompetitive bid, Single-price auction, and Weighted-average.

The additions and revisions read as follows:

§ 356.2

What definitions do I need to know to understand this part?

13-week bill means a Treasury bill where the security description is “13-Week Bill” as referenced on the Treasury auction announcement.

* * * * *

Competitive bid means a bid to purchase a stated par amount of securities at a specified yield, discount rate, or discount margin.

* * * * *

Discount margin means the margin over the index that equates the present values of the assumed cash flows on a floating rate note to the sum of the price of and accrued interest on the floating rate note. The assumed cash flows are calculated based upon the index rate applicable to the dated date. Bidders in floating rate note auctions bid on the basis of discount margin. (See appendix B.)

* * * * *

Index means the Consumer Price Index for inflation-protected securities. For floating rate notes, the index is the highest accepted discount rate on 13-week bills determined by Treasury auctions of those securities.

Index rate means the simple-interest money market yield, computed on an actual/360 basis and rounded to nine decimal places, from the highest accepted discount rate of a 13-week bill auction as announced in the Treasury auction results press release. (See appendix B for methods and examples for computing the index rate.)

* * * * *

Interest rate means the annual percentage rate of interest paid on the par amount (or the inflation-adjusted principal) of a specific issue of notes or bonds. For floating rate notes, the interest rate is the spread plus the index rate, which resets daily based on the most recent auction of 13-week bills, and is subject to a minimum daily interest accrual rate of zero percent. (See appendix B for methods and examples of interest calculations.)

* * * * *

Multiple-price auction means an auction in which each successful competitive bidder pays the price equivalent to the yield, discount rate, or discount margin that it bid.

Noncompetitive bid means, for a single-price auction, a bid to purchase a stated par amount of securities at the highest yield, discount rate, or discount margin awarded to competitive bidders. For a multiple-price auction, a noncompetitive bid means a bid to purchase securities at the weighted average yield, discount rate, or discount margin of awards to competitive bidders.

* * * * *

Single-price auction means an auction in which all successful bidders pay the same price regardless of the yields, discount rates, or discount margins they each bid.

Spread means the fixed amount over the life of a floating rate note that is added to the index rate in order to determine the interest rate of the floating rate note. The spread will be determined in the auction of a new floating rate note and is expressed in tenths of a basis point (i.e., to three decimals). Additionally, the spread will be equal to the high discount margin at the time a new floating rate note is auctioned.

* * * * *

Weighted-average means the average of the yields, discount rates, or discount margins at which we award securities to competitive bidders in multiple-price auctions weighted by the par amount of securities allotted at each yield, discount rate, or discount margin.

* * * * *

4. In § 356.5, in paragraph (b)(1), revise referenced footnote  ¹ and add paragraph (b)(3) to read as follows:

§ 356.5

What types of securities does the Treasury auction?

* * * * *

(b) * * *

(1) * * *

¹ We use the term “non-indexed” in this part to distinguish such notes and bonds from “inflation-protected securities” and “floating rate notes.” We refer to non-indexed notes and non-indexed bonds as “notes” and “bonds” in official Treasury publications, such as auction announcements and auction results press releases, as well as in auction systems.

* * * * *

(3) Treasury floating rate notes. (i) Are issued with a stated spread to be added to the index rate for daily interest accrual throughout each interest payment period;

(ii) Have a zero-percent minimum daily interest accrual rate;

(iii) Have interest payable quarterly;

(iv) Are redeemed at their par amount at maturity;

(v) Are sold at discount, par, or premium depending on the auction results (See appendix B for price and interest payment calculations and appendix C for Investment Considerations.); and

(vi) Have maturities of at least one year, but not more than ten years.

* * * * *

Subpart B—Bidding, Certifications, and Payment.

5. In § 356.12, add paragraph (c)(1)(iv) and revise paragraph (c)(2) to read as follows:

§ 356.12

What are the different types of bids and do they have specific requirements or restrictions?

* * * * *

(c)(1) * * *

(iv) Treasury floating rate notes. A competitive bid must show the discount margin bid, expressed as a percentage with three decimals, for example, 0.290 percent. We will treat any missing decimals as zero, for example, a bid of 0.29 will be treated as 0.290. The discount margin bid may be positive, negative, or zero.

(2) Maximum recognized bid. There is no limit on the maximum dollar amount that you may bid for competitively, either at a single yield, discount rate, or discount margin, or at different yields, discount rates, or discount margins. However, a competitive bid at a single yield, discount rate, or discount margin that exceeds 35 percent of the offering amount will be reduced to that amount. For example, if the offering amount is $10 billion, the maximum bid amount we will recognize at any one yield, discount rate, or discount margin from any bidder is $3.5 billion. (See § 356.22 for award limitations.)

* * * * *

6. In § 356.14, revise the first sentence of paragraph (d) to read as follows:

§ 356.14

What are the requirements for submitting bids for customers?

* * * * *

(d) Competitive customer bids. For each customer competitive bid, the submitter must provide the customer's name, the amount bid, and the yield, discount rate, or discount margin. * * *

* * * * *

7. In § 356.15, revise the first sentence of paragraph (e) to read as follows:

§ 356.15

What rules apply to bids submitted by investment advisors?

* * * * *

(e) Proration of awards. Investment advisers that submit competitive bids in the names of controlled accounts are responsible for prorating any awards at the highest accepted yield, discount rate, or discount margin using the same percentage that we announce. * * *

* * * * *

Subpart C—Determination of Auction Awards; Settlement.

8. In § 356.20, revise paragraph (a)(1) and (2), redesignate paragraph (c) as paragraph (d), add a new paragraph (c), and revise newly redesignated paragraphs (d)(1) and (2) to read as follows:

§ 356.20

How does the Treasury determine auction awards?

(a) Determining the range and amount of accepted competitive bids—(1) Accepting bids. First we accept in full all non-competitive bids that were submitted by the noncompetitive bidding deadline. After the closing time for receipt of competitive bids we start accepting those at the lowest yields, discount rates, or discount margins, through successively higher yields, discount rates, or discount margins, up to the amount required to meet the offering amount. When necessary, we prorate bids at the highest accepted yield, discount rate, or discount margin as described below. If the amount of noncompetitive bids would absorb all or most of the offering amount, we will accept competitive bids in an amount sufficient to provide a fair determination of the yield, discount rate, or discount margin for the securities we are auctioning.

(2) Accepting bids at the high yield, discount rate, or discount margin. Generally, the total amount of bids at the highest accepted yield, discount rate, or discount margin exceeds the offering amount remaining after we accept the noncompetitive bids and the competitive bids at the lower yields, discount rates, or discount margins. In order to keep the total amount of awards as close as possible to the announced offering amount, we award a percentage of the bids at the highest accepted yield, discount rate, or discount margin. We derive the percentage by dividing the remaining par amount needed to fill the offering amount by the par amount of the bids at the high yield, discount rate, or discount margin and rounding up to the next hundredth of a whole percentage point, for example, 17.13%.

* * * * *

(c) Determining the interest rate for floating rate notes. The interest rate will be the spread plus the index rate (as it may be adjusted on the calendar day following each auction of 13-week bills) subject to a minimum daily interest accrual rate of zero percent.

(d) * * *

(1) Single-price auctions. We award securities to both noncompetitive and competitive bidders at the price equivalent to the highest accepted yield, discount rate, or discount margin at which bids were accepted. For inflation-protected securities, the price for awarded securities is the price equivalent to the highest accepted real yield.

(2) Multiple-price auctions—(i) Competitive bids. We award securities to competitive bidders at the price equivalent to each yield, discount rate, or discount margin at which their bids were accepted.

(ii) Noncompetitive bids. We award securities to noncompetitive bidders at the price equivalent to the weighted average yield, discount rate, or discount margin of accepted competitive bids.

9. In § 356.21, revise the section heading, the first three sentences of paragraph (a), and the last sentence of paragraph (b) to read as follows:

§ 356.21

How are awards at the high yield, discount rate, or discount margin calculated?

(a) Awards to submitters. We generally prorate bids at the highest accepted yield, discount rate, or discount margin under § 356.20(a)(2) of this part. For example, if 80.15% is the announced percentage at the highest yield, discount rate, or discount margin, we award 80.15% of the amount of each bid at that yield, discount rate, or discount margin. A bid for $100 million at the highest accepted yield, discount rate, or discount margin would be awarded $80,150,000 in this example. * * *

(b) Awards to customers. * * * For example, if 80.15% is the announced percentage at the highest yield, discount rate, or discount margin, then each customer bid at that yield, discount rate, or discount margin must be awarded 80.15%.

10. In § 356.23, revise paragraph (b)(2) to read as follows:

§ 356.23

How are the auction results announced?

* * * * *

(b) * * *

(2) The range of accepted yields, discount rates, or discount margins.

* * * * *

Subpart D—Miscellaneous Provisions.

11. In § 356.30, revise the fourth sentence of paragraph (a) to read as follows:

§ 356.30

When does the Treasury pay principal and interest on securities?

(a) * * * Interest is payable on a semiannual or quarterly basis on the interest payment dates specified in the auction announcement through the maturity date. * * *

* * * * *

12. In § 356.31, revise the first sentence of paragraph (a) and the paragraph (b) heading to read as follows:

§ 356.31

How does the STRIPS program work?

(a) General. Notes or bonds (other than Treasury floating rate notes) may be “stripped”—divided into separate principal and interest components. * * *

(b) Treasury non-indexed securities (notes and bonds other than Treasury inflation-protected securities or Treasury floating rate notes) * * *

13. In § 356.32, add paragraph (c) to read as follows:

§ 356.32

What tax rules apply?

* * * * *

(c) Treasury floating rate notes. Special federal income tax rules for floating rate notes are set forth in Internal Revenue Service regulations.

14. In Appendix A to Part 356, Section II, revise paragraph (d)(1) to read as follows:

Appendix A to Part 356—Bidder Categories

* * * * *

II. How to Obtain Separate Bidder Recognition

* * * * *

(d) * * *

(1) Exchanging any of the following information with any other part of the corporate [partnership] structure: (a) Yields, discount rates, or discount margins at which it plans to bid; (b) amounts of securities for which it plans to bid; (c) positions that it holds or plans to acquire in a security being auctioned; and (d) investment strategies that it plans to follow regarding the security being auctioned, or

* * * * *

15. In Appendix B to Part 356:

a. Amend the introductory listing of sections by redesignating sections IV and V as sections V and VI, and adding new section IV;

b. In section I., redesignate subsection C as subsection D and add new subsection C;

c. In newly redesignated subsection D, add paragraph 6;

d. Redesignate sections IV and V as sections V and VI; and

e. Add new section IV.

The additions read as follows:

Appendix B to Part 356—Formulas and Tables

* * * * *

IV. Formulas for Conversion of Floating Rate Note Discount Margins to Equivalent Prices

* * * * *

I. Computation of Interest on Treasury Bonds and Notes

* * * * *

C. Treasury Floating Rate Notes

1. Indexing and Interest Payment Process. We issue floating rate notes with a daily interest accrual feature. This means that the interest rate “floats” based on changes in the representative index rate. We pay interest on a quarterly basis. The index rate is the High Rate of the 13-week Treasury bill auction announced on the auction results press release that has been converted into a simple-interest money market yield computed on an actual/360 basis and rounded to nine decimal places. Interest payments are based on the floating rate note's variable interest rate from, and including, the dated date or last interest payment date to, but excluding, the next interest payment or maturity date. We make quarterly interest payments by accruing the daily interest amounts and adding those amounts together for the interest payment period.

2. Interest Rate. The interest rate on floating rate notes will be the spread plus the index rate (as it may be adjusted on the calendar day following each auction of 13-week bills).

3. Interest Accrual. In general, accrued interest for a particular calendar day in an accrual period is calculated by using the index rate from the most recent auction of 13-week bills that took place before the accrual day, plus the spread determined at the time of a new floating rate note auction, divided by 360, subject to a zero-percent minimum daily interest accrual rate. However, a 13-week bill auction that takes place in the two-business-day period prior to a settlement date or interest payment date will be excluded from the calculation of accrued interest for purposes of the settlement amount or interest payment. Any changes in the index rate that would otherwise have occurred during this two-business-day period will occur on the first calendar day following the end of the period.

4. Index Contingencies.

(i) If Treasury were to discontinue auctions of 13-week bills, the Secretary has authority to determine and announce a new index for outstanding floating rate notes.

(ii) If Treasury were to not conduct a 13-week bill auction in a particular week, then the interest rate in effect for the notes at the time of the last 13-week bill auction results announcement will remain in effect until such time, if any, as the results of a 13-week Treasury auction are again announced by Treasury. Treasury reserves the right to change the index rate for any newly issued floating rate note.

* * * * *

D. Accrued Interest

* * * * *

6. For a floating rate note, if accrued interest covers a portion of a full quarterly interest payment period, we calculate accrued interest as shown in section IV, paragraphs C and D of this appendix.

* * * * *

IV. Formulas for Conversion of Floating Rate Note Discount Margins to Equivalent Prices

Definitions for Newly Issued Floating Rate Notes

P = the price per $100 par value.

T₀ = the issue date.

N = the total number of quarterly interest payments.

i and k = indexes that identify the sequence of interest payment dates.

T_i = the i^th quarterly interest payment date.

T_i − T_i-1 = the number of days between the interest payment date T_i and the preceding interest payment date.

T _N = the maturity date.

r = the index rate applicable to the issue date.

s = the spread.

m = the discount margin.

A. For newly issued floating rate notes issued at par:

Formula:

Example:

The purpose of this example is to demonstrate how a floating rate note price is derived at the time of original issuance. Additionally, this example depicts the association of the July 31, 2012 issue date and the two-business-day lockout period. For a new two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, and an interest accrual rate on the issue date of 0.215022819% (index rate of 0.095022819% plus a spread of 0.120%), solve for the price per 100 (P). This interest accrual rate is used for each daily interest accrual over the life of the security for the purposes of this example. In a new issuance (not a reopening) of a floating rate note, the discount margin determined at auction will be equal to the spread.

Definitions:

T₀ = July 31, 2012.

N = 8.

T _N = July 31, 2014.

r = 0.095022819%.

s = 0.120%.

m = 0.120%.

As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:

Table 1—13-Week Bill Auction Data

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate	Index rate
7/23/2012	7/26/2012	10/25/2012	99.975986	0.095%	0.095022819%

The rationale for using a 13-week bill auction that has occurred at least two days prior to the issue date is due to the two-business-day lockout period. This lockout period applies only to the issue date and interest payment dates, thus any 13-week bill auction that occurs during the two-day lockout period is not used for calculations related to the issue date and interest payment dates. The following sample calendar depicts this relationship for the floating rate note issue date.

Computing the Projected Cash Flows

The following table presents the future interest payment dates and the number of days between them.

Table 2—Payment Dates

Dates	Days between dates
Issue Date: T0 = 7/31/2012
1st Interest Date: T1 = 10/31/2012	T1 − T0 = 92
2nd Interest Date: T2 = 1/31/2013	T2 − T1 = 92
3rd Interest Date: T3 = 4/30/2013	T3 − T2 = 89
4th Interest Date: T4 = 7/31/2013	T4 − T3 = 92
5th Interest Date: T5 = 10/31/2013	T5 − T4 = 92
6th Interest Date: T6 = 1/31/2014	T6 − T5 = 92
7th Interest Date: T7 = 4/30/2014	T7 − T6 = 89
8th Interest & Maturity Dates: T8 = 7/31/2014	T8 − T7 = 92

Let

a_i = 100 × max(r + s, 0)/360

and

A_i = a_i × (T_i − T_i−1) + 100 × 1_{i=8}

a_i represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates T_i−1 and T_i, where i = 1,2, . . . ,8. a_i's are computed using the spread s = 0.120% obtained at the auction, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:

a₁ = 100 × max(0.00095022819 + 0.00120,0)/360 = 0.000597286

A_i represents the projected cash flow the floating rate note holder will receive, for a $100 par value, at the future interest payment date T_i, where i = 1,2, . . . ,8. T_i − T_i−1 is the number of days between the future interest payment dates T_i−1 and T_i. To account for the payback of the par value, the variable 1_{i=8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:

A_i = 92 × 0.000597286 = 0.054950312

and

A₈ = 92 × 0.000597286 + 100 = 100.054950312

Let

B_i = 1 + (r + m) × (T_i − T_{i − 1})/360

B_i represents the projected compound factor between the future dates T_i−1 and T_i, where i = 1,2, . . . ,8. All B_i's are computed using the discount margin m = 0.120% (equals the spread determined at the auction), and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:

B₃ = 1 + (0.00095022819 + 0.00120) × 89/360 = 1.000531584.

The following table shows the projected daily accrued interest values for $100 par value (a_i's), cash flows at interest payment dates (A_i's), and the compound factors between payment dates (B_i's).

Table 3—Projected Cash Flows and Compound Factors

i	ai	Ai	Bi
1	0.000597286	0.054950312	1.000549503
2	0.000597286	0.054950312	1.000549503
3	0.000597286	0.053158454	1.000531584
4	0.000597286	0.054950312	1.000549503
5	0.000597286	0.054950312	1.000549503
6	0.000597286	0.054950312	1.000549503
7	0.000597286	0.053158454	1.000531584
8	0.000597286	100.054950312	1.000549503

Computing the Price

The price is computed as follows:

B. For newly issued floating rate notes issued at a premium:

Formula:

Example:

The purpose of this example is to demonstrate how a floating rate note auction can result in a price at a premium given a negative discount margin and spread at auction. For a new two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, solve for the price per 100 (P). In a new issue (not a reopening) of a floating rate note, the discount margin established at auction will be equal to the spread. In this example, the discount margin determined at auction is −0.150%, but the floating rate note is subject to a daily interest rate accrual minimum of 0.000%.

Definitions:

T₀ = July 31, 2012.

N = 8.

T _N = July 31, 2014.

r = 0.095022819%.

s = −0.150%.

m = −0.150%.

As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:

Table 1—13-Week Bill Auction Data

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate	Index rate
7/23/2012	7/26/2012	10/25/2012	99.975986	0.095%	0.095022819%

Computing the Projected Cash Flows

The following table presents the future interest payment dates and the number of days between them.

Table 2—Payment Dates

Dates	Days between dates
Issue Date: T0 = 7/31/2012
1st Interest Date: T1 = 10/31/2012	T1 − T0 = 92
2nd Interest Date: T2 = 1/31/2013	T2 − T1 = 92
3rd Interest Date: T3 = 4/30/2013	T3 − T2 = 89
4th Interest Date: T4 = 7/31/2013	T4 − T3 = 92
5th Interest Date: T5 = 10/31/2013	T5 − T4 = 92
6th Interest Date: T6 = 1/31/2014	T6 − T5 = 92
7th Interest Date: T7 = 4/30/2014	T7 − T6 = 89
8th Interest & Maturity Dates: T8 = 7/31/2014	T8 − T7 = 92

Let

a_i = 100 × max(r + s, 0)/360

and

A_i = a_i × (T_i−T_{i− 1}) + 100×1_{i=8}

a_i Represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates T_{i− 1} and T_i where i = 1,2, . . . ,8. a_i's are computed using the spread s = − 0.150%, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:

a_i = 100 × max(0.00095022819−0.00150,0)/360 = 100 × 0/360 = 0.000000000

A_i represents the projected cash flow the floating rate note holder will receive, for a $100 par value, at the future interest payment date T_i, where i = 1,2, . . ., 8. T_i - T_i−1 is the number of days between the future interest payment dates T_i−1 and T_i. To account for the payback of the par value, the variable 1_{i=_8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:

A₁ = 92 × 0.000000000 = 0.000000000

and

A₈ = 92 × 0.000000000 + 100 = 100.000000000

Let

B_i = 1 + (r + m) × (T_i-T_i−1)/360

B_i represents the projected compound factor between the future dates T_i−1 and T_i, where i = 1,2, . . ., 8. All B_i's are computed using the discount margin m = −0.150% (equals the spread obtained at the auction), and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:

B₃ = 1 + (0.00095022819−0.00150) × 89/360 = 0.999864084.

The following table shows the projected daily accrued interests for $100 par value (a_i's), cash flows at interest payment dates (A_i's), and the compound factors between payment dates (B_i's).

Table 3—Projected Cash Flows and Compound Factors

i	ai	Ai	Bi
1	0.000000000	0.000000000	0.999859503
2	0.000000000	0.000000000	0.999859503
3	0.000000000	0.000000000	0.999864084
4	0.000000000	0.000000000	0.999859503
5	0.000000000	0.000000000	0.999859503
6	0.000000000	0.000000000	0.999859503
7	0.000000000	0.000000000	0.999864084
8	0.000000000	100.000000000	0.999859503

Computing the Price

The price is computed as follows:

Definitions for Reopenings of Floating Rate Notes and Calculation of Interest Payments

IP_i = the quarterly interest payment at date T_i.

P _D = the price that includes the accrued interest per $100 par value as of the reopening issue date.

AI = accrued interest per $100 par value as of the reopening issue date.

P _C = the price without accrued interest per $100 par value as of the reopening issue date.

T₋₁ = the dated date if the reopening occurs before the first interest payment date, or, otherwise, the latest interest payment date prior to the reopening issue date.

T₀ = the reopening issue date.

N = the total number of remaining quarterly interest payments as of the reopening issue date.

i and k = indexes that identify the sequence of interest payment dates relative to the issue date. For example T₁, T₂, and T₃ represent the first, second, and the third interest payment dates after the issue date respectively, while T₋₁ represents the preceding interest payment date before the issue date.

j = an index that identifies days between consecutive interest payment dates.

T_i = the i^th remaining quarterly interest payment date.

T_i − T_i−1 = the number of days between the interest payment date T_i and the preceding interest payment date.

T _N = the maturity date.

r_j's = the effective index rates for days between the last interest payment date and the reopening issue date.

r = the index rate applicable to the reopening issue date.

s = the spread.

m = the discount margin.

C. Pricing and accrued interest for reopened floating rate notes

Formula:

Example:

The purpose of this example is to determine the floating rate note prices with and without accrued interest at the time of the reopening auction. For a two-year floating rate note that was originally auctioned on July 25, 2012, with an issue date of July 31, 2012, reopened in an auction on August 30, 2012 and issued on August 31, 2012, with a maturity date of July 31, 2014, solve for accrued interest per 100 (AI), the price with accrued interest per 100 (P_D) and the price without accrued interest per 100 (P_C). Since this is a reopening of an original issue from the prior month, Table 2 as shown in the example is used for accrued interest calculations. In the case of floating rate note reopenings, the spread on the security remains equal to the spread that was established at the original auction of the floating rate notes.

Definitions:

T₋₁ = July 31, 2012.

T₀ = August 31, 2012.

N = 8.

T _N = July 31, 2014.

r = 0.105027876%.

s = 0.120%.

m = 0.100%.

The following table shows the past results for the 13-week bill auction.

Table 1—13-Week Bill Auction Data

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate (percent)	Index rate (percent)
7/23/2012	7/26/2012	10/25/2012	99.975986	0.095	0.095022819
7/30/2012	8/2/2012	11/1/2012	99.972194	0.110	0.110030595
8/6/2012	8/9/2012	11/8/2012	99.974722	0.100	0.100025284
8/13/2012	8/16/2012	11/15/2012	99.972194	0.110	0.110030595
8/20/2012	8/23/2012	11/23/2012	99.973167	0.105	0.105028183
8/27/2012	8/30/2012	11/29/2012	99.973458	0.105	0.105027876

The following table shows the index rates applicable for the accrued interest.

Table 2—Applicable Index Rate

Accrual starts	Accrual ends	Number of days in accrual period	Applicable floating rate
			Auction date	Index rate (percent)
7/31/2012	7/31/2012	1	7/23/2012	0.095022819
8/1/2012	8/6/2012	6	7/30/2012	0.110030595
8/7/2012	8/13/2012	7	8/6/2012	0.100025284
8/14/2012	8/20/2012	7	8/13/2012	0.110030595
8/21/2012	8/27/2012	7	8/20/2012	0.105028183
8/28/2012	8/30/2012	3	8/27/2012	0.105027876

Computing the Accrued Interest

The accrued interest as of the new issue date (8/31/2012) for a $100 par value is:

AI = 1 × 100 × max (0.00095022819 + 0.00120,0)/360

+ 6 × 100 × max (0.00110030595 + 0.00120,0)/360

+ 7 × 100 × max (0.00100025284 + 0.00120,0)/360

+ 7 × 100 × max (0.00110030595 + 0.00120,0)/360

+ 7 × 100 × max (0.00105028183 + 0.00120,0)/360

+ 3 × 100 × max (0.00105027876 + 0.00120,0)/360

AI = 1×0.000597286

+ 6×0.000638974

+ 7×0.000611181

+ 7×0.000638974

+ 7×0.000625078

+ 3×0.000625077

AI = 0.000597286 + 0.003833844 + 0.004278267 + 0.00472818 + 0.004375546 + 0.001875231

AI = 0.019432992 = $0.019433

Computing the Projected Cash Flows

The following table presents the future interest payment dates and the number of days between them.

Table 3—Payment Dates

Dates	Days between dates
Original Issue Date: T−1 = 7/31/2012
New Issue Date: T0 = 8/31/2012	T0 − T−1 = 31
1st Interest Date: T1 = 10/31/2012	T1 − T0 = 61
2nd Interest Date: T2 = 1/31/2013	T2 − T1 = 92
3rd Interest Date: T3 = 4/30/2013	T3 − T2 = 89
4th Interest Date: T4 = 7/31/2013	T4 − T3 = 92
5th Interest Date: T5 = 10/31/2013	T5 − T4 = 92
6th Interest Date: T6 = 1/31/2014	T6 − T5 = 92
7th Interest Date: T7 = 4/30/2014	T7 − T6 = 89
8th Interest & Maturity Dates: T8 = 7/31/2014	T8 − T7 = 92

Let

a₁ = 100 × max(r + s, 0)/360

and

A_i = a_i × (T_i − T_i−1) + 100×1_{i=8}

a₁ represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates T_i−1 and T₁, where i=1,2,...,8. a_i's are computed using the spread s = 0.120% obtained at the original auction, and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012). For example:

a_i = 100 × max(0.00105027876 + 0.00120,0)/360 = 0.000625077

A_i represents the projected cash flow the floating rate note holder will receive, less accrued interest, for a $100 par value, at the future interest payment date T_i, where i=1,2,...,8. T_i−1 is the number of days between the future interest payment dates T_i−1 and T_i. To account for the payback of the par value, the variable 1_{i=8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:

A_i = 61×0.000625077 = 0.038129697

and

A₈ = 92×0.000625077 + 100 = 100.057507084

Let

B_i = 1 + (r + m)×(T_i−1)/360

B_i represents the projected compound factor between the future dates T_i−1 and T_i, where i=1,2,...,8. All B_i's are computed using the discount margin m = 0.100% obtained at the reopening auction, and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012). For example:

B₃ = 1 + (0.00105027876 + 0.00100)×89/360 = 1.000506874

The following table shows the projected daily accrued interests for $100 par value (a_i's), cash flows at interest payment dates (A_i's), and the compound factors between payment dates (B_i's).

Table 4—Projected Cash Flows and Compound Factors

i	ai	Ai	Bi
1	0.000625077	0.038129697	1.000347408
2	0.000625077	0.057507084	1.000523960
3	0.000625077	0.055631853	1.000506874
4	0.000625077	0.057507084	1.000523960
5	0.000625077	0.057507084	1.000523960
6	0.000625077	0.057507084	1.000523960
7	0.000625077	0.055631853	1.000506874
8	0.000625077	100.057507084	1.000523960

Computing the Price

The price with accrued interest is computed as follows:

D. For calculating interest payments:

Example:

For a new issue of a two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, and a first interest payment date of October 31, 2012, calculate the quarterly interest payments (IP_I) per 100. In a new issuance (not a reopening) of a new floating rate note, the discount margin determined at auction will be equal to the spread. The interest accrual rate used for this floating rate note on the issue date is 0.215022819% (index rate of 0.095022819% plus a spread of 0.120%) and this rate is used for each daily interest accrual over the life of the security for the purposes of this example.

Example 1: Projected interest payment as of the original issue date.

T₀ = July 31, 2012.

N = 8.

T _N = July 31, 2014.

r = 0.095022819%.

s = 0.120%.

m = 0.120%.

As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:

Table 1—13-Week Bill Auction Data

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate	Index rate
7/23/2012	7/26/2012	10/25/2012	99.975986	0.095%	0.095022819%

Computing the Projected Cash Flows

The following table presents the future interest payment dates and the number of days between them.

Table 2—Payment Dates

Dates	Days between dates
Issue Date: T0 = 7/31/2012
1st Interest Date: T1 = 10/31/2012	T1 − T0 = 92
2nd Interest Date: T2 = 1/31/2013	T2 − T1 = 92
3rd Interest Date: T3 = 4/30/2013	T3 − T2 = 89
4th Interest Date: T4 = 7/31/2013	T4 − T3 = 92
5th Interest Date: T5 = 10/31/2013	T5 − T4 = 92
6th Interest Date: T6 = 1/31/2014	T6 − T5 = 92
7th Interest Date: T7 = 4/30/2014	T7 − T6 = 89
8th Interest & Maturity Dates: T8 = 7/31/2014	T8 − T7 = 92

Using the spread s = 0.120%, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012), the first and seventh projected interest payments are computed as follows:

IP₁ = 92×[100×max(0.00095022819 + 0.00120,0)/360]

IP₁ = 92×0.000597286 = 0.054950312

IP₇ = 89×[100×max(0.00095022819 + 0.00120,0)/360]

IP₇ = 89×0.000597286 = 0.053158454

The following table shows all projected interest payments as of the issue date.

Table 3—Projected Interest Payments

i	Dates	IPi
1	10/31/2012	0.054950312
2	1/31/2013	0.054950312
3	4/30/2013	0.053158454
4	7/31/2013	0.054950312
5	10/31/2013	0.054950312
6	1/31/2014	0.054950312
7	4/30/2014	0.053158454
8	7/31/2014	0.054950312

Example 2: Projected interest payment as of the reopening issue date (intermediate values, including rates in percentage terms, are rounded to nine decimal places).

This example demonstrates the calculations required to determine the interest payment due when the reopened floating rate note is issued. This example also demonstrates the need to calculate accrued interest at the time of a floating rate reopening auction. Since this is a reopening of an original issue from 31 days prior, Table 5 as shown in the example is used for accrued interest calculations. For a two-year floating rate note originally auctioned on July 25, 2012 with an original issue date of July 31, 2012, reopened by an auction on August 30, 2012 and issued on August 31, 2012, with a maturity date of July 31, 2014, calculate the quarterly interest payments (IP_I) per 100. T₋₁ is the dated date if the reopening occurs before the first interest payment date, or otherwise the latest interest payment date prior to the new issue date.

T₋₁ = July 31, 2012.

T₀ = August 31, 2012.

N = 8.

T _N = July 31, 2014.

r = 0.105027876%.

s = 0.120%.

m = 0.100%.

The following table shows the past results for the 13-week bill auction.

Table 4—13-Week Bill Auction Data

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate (percent)	Index rate (percent)
7/23/2012	7/26/2012	10/25/2012	99.975986	0.095	0.095022819
7/30/2012	8/2/2012	11/1/2012	99.972194	0.110	0.110030595
8/6/2012	8/9/2012	11/8/2012	99.974722	0.100	0.100025284
8/13/2012	8/16/2012	11/15/2012	99.972194	0.110	0.110030595
8/20/2012	8/23/2012	11/23/2012	99.973167	0.105	0.105028183
8/27/2012	8/30/2012	11/29/2012	99.973458	0.105	0.105027876

The following table shows the index rates applicable for the accrued interest.

Table 5—Applicable Index Rate

Accrual starts	Accrual ends	Number of days in accrual period	Applicable floating rate
			Auction date	Index rate (percent)
7/31/2012	7/31/2012	1	7/23/2012	0.095022819
8/1/2012	8/6/2012	6	7/30/2012	0.110030595
8/7/2012	8/13/2012	7	8/6/2012	0.100025284
8/14/2012	8/20/2012	7	8/13/2012	0.110030595
8/21/2012	8/27/2012	7	8/20/2012	0.105028183
8/28/2012	8/30/2012	3	8/27/2012	0.105027876

Computing the accrued interest

The accrued interest as of 8/31/2012 for a $100 par value is:

AI = 1 × 100 × max (0.00095022819 + 0.00120,0)/360

+ 6 × 100 × max (0.00110030595 + 0.00120,0)/360

+ 7 × 100 × max (0.00100025284 + 0.00120,0)/360

+ 7 × 100 × max (0.00110030595 + 0.00120,0)/360

+ 7 × 100 × max (0.00105028183 + 0.00120,0)/360

+ 3 × 100 × max (0.00105027876 + 0.00120,0)/360

AI = 1 × 0.000597286

+ 6 × 0.000638974

+ 7 × 0.000611181

+ 7 × 0.000638974

+ 7 × 0.000625078

+ 3 × 0.000625077

AI = 0.000597286 + 0.003833844 + 0.004278267 + 0.004472818 + 0.004375546 + 0.001875231

AI = 0.019432992 = $0.019433

The following table presents the future interest payment dates and the number of days between them.

Table 6—Payment Dates

Dates	Days between dates
Original Issue Date: T−1 = 7/31/2012
New Issue Date: T0 = 8/31/2012	T0 − T−1 = 31
1st Interest Date: T1 = 10/31/2012	T1 − T0 = 61
2nd Interest Date: T2 = 1/31/2013	T2 − T1 = 92
3rd Interest Date: T3 = 4/30/2013	T3 − T2 = 89
4th Interest Date: T4 = 7/31/2013	T4 − T3 = 92
5th Interest Date: T5 = 10/31/2013	T5 − T4 = 92
6th Interest Date: T6 = 1/31/2014	T6 − T5 = 92
7th Interest Date: T7 = 4/30/2014	T7 − T6 = 89
8th Interest & Maturity Dates: T8 = 7/31/2014	T8 − T7 = 92

Using the original spread s = 0.120% (obtained on 7/25/2012), and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012), the first and eighth projected interest payments are computed as follows:

IP₁ = 0.019432992 + 61 × [100 × max (0.00105027876 + 0.00120,0)/360]

IP₁ = 0.019432992 + 61 × 0.000625077

IP₁ = 0.019432992 + 0.038129697 = 0.057562689

and

IP₈ = 92 × [100 × max (0.00105027876 + 0.00120,0)/360]

IP₈ = 92 × 0.000625077 = 0.057507084

The following table shows all projected interest payments as of the new issue date.

Table 7—Projected Interest Payments

i	Dates	IPi
1	10/31/2012	0.057562689
2	1/31/2013	0.057507084
3	4/30/2013	0.055631853
4	7/31/2013	0.057507084
5	10/31/2013	0.057507084
6	1/31/2014	0.057507084
7	4/30/2014	0.055631853
8	7/31/2014	0.057507084

Definitions for Newly Issued Floating Rate Notes with an Issue Date that Occurs after the Dated Date

P_D = the price that includes accrued interest from the dated date to the issue date per $100 par value as of the issue date.

AI = the accrued interest per $100 par value as of the issue date.

P_C = the price without accrued interest per $100 par value as of the issue date.

T₋₁ = the dated date.

T₀ = the issue date.

N = the total number of remaining quarterly interest payments as of the new issue date.

i and k = indexes that identify the sequence of interest payment dates.

j = an index that identifies days between the dated date and the issue date.

T_i = the i^th quarterly future interest payment date.

T_i − T_i−1 = the number of days between the interest payment date T_i and the preceding interest payment date.

T_N = the maturity date.

r_j's = the effective index rates for days between the dated date and the issue date.

r = the index rate applicable to the issue date.

s = the spread.

m = the discount margin.

E. Pricing and accrued interest for new issue floating rate notes with an issue date that occurs after the dated date

Formula:

Example:

The purpose of this example is to demonstrate how a floating rate note can have a price without accrued interest of less than $100 par value when the issue date occurs after the dated date. An original issue two-year floating rate note is auctioned on December 29, 2011, with a dated date of December 31, 2011, an issue date of January 3, 2012, and a maturity date of December 31, 2013.

Definitions:

Dated date = 12/31/2011.

Issue date = 1/3/2012.

Maturity date = 12/31/2013.

Spread = 1.000% at auction.

Discount margin = 1.000%.

As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:

Table 1—13-WEEK BILL AUCTION DATA

Auction date	Issue date	Maturity date	Auction clearing price	Auction high rate	Index rate
12/27/2011	12/29/2011	3/29/2012	99.993681	0.025%	0.025001580%

The following table shows the index rates applicable for the accrued interest.

Table 2—Applicable Index Rate

Accrual starts	Accrual ends	Number of days in accrual period	Applicable floating rate
			Auction date	Index rate
12/31/2011	1/2/2012	3	12/27/2011	0.025001580%

Computing the accrued interest

The accrued interest as of the new issue date (1/3/2012) for a $100 par value is:

AI = 3 × 100 × max (0.00025001580 + 0.01000,0)/360

AI = 3 × 0.002847227

AI = 0.008541681 = $0.008542

Computing the Projected Cash Flows

The following table presents the future interest payment dates and the number of days between them.

Table 3—Payment Dates

Dates	Days between dates
Dated Date: = T−1 = 12/31/2011
Issue Date: T0 = 1/3/2012	T0 − T−1 = 3
1st Interest Date: T1 = 3/31/2012	T1 − T0 = 88
2nd Interest Date: T2 = 6/30/2012	T2 − T1 = 91
3rd Interest Date: T3 = 9/30/2012	T3 − T2 = 92
4th Interest Date: T4 = 12/31/2012	T4 − T3 = 92
5th Interest Date: T5 = 3/31/2013	T5 − T4 = 90
6th Interest Date: T6 = 6/30/2013	T6 − T5 = 91
7th Interest Date: T7 = 9/30/2013	T7 − T6 = 92
8th Interest & Maturity Dates: T8 = 12/31/2013	T8 − T7 = 92

Let

a_i = 100 × max(r + s, 0)/360

and

A_i = a_i × (T_i − T_i−1) + 100 × 1_{i=8}

a_i represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates T_i−1 and T_i, where i = 1,2,...,8. a_i's are computed using the spread s = 1.000% obtained at the auction, and the fixed index rate of r = 0.025001580% applicable to the issue date (1/3/2012). For example:

a₁ = 100 × max(0.00025001580 + 0.01000,0)/360 = 0.002847227

A_i represents the projected cash flow the floating rate note holder will receive, less accrued interest, for a $100 par value, at the future interest payment date T_i, where i = 1,2,...,8. T_i − T_i−1 is the number of days between the future interest payment dates T_i−1 and T₁. To account for the payback of the par value, the variable 1_{i=8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:

A₁ = 88 × 0.002847227 = 0.250555976

and

A₈ = 92 × 0.002847227 + 100 = 100.261944884

Let

B_i = 1 + (r + m) × (T _i − T_i−1)/360

B_i represents the projected compound factor between the future dates T_i−1 and T_i, where i = 1,2,...,8. All B_i's are computed using the discount margin m = 1.000% (equals the spread obtained at the auction), and the fixed index rate of r = 0.025001580% applicable to the issue date (1/3/2012). For example:

B₃ = 1 + (0.00025001580 + 0.01000) × 92/360 = 1.002619448

The following table shows the projected daily accrued interests for $100 par value (a_i 's), cash flows at interest payment dates (A_i 's), and the compound factors between payment dates (B_i's).

Table 4—Projected Cash Flows and Compound Factors

i	ai	Ai	Bi
1	0.002847227	0.250555976	1.002505559
2	0.002847227	0.259097657	1.002590976
3	0.002847227	0.261944884	1.002619448
4	0.002847227	0.261944884	1.002619448
5	0.002847227	0.256250430	1.002562504
6	0.002847227	0.259097657	1.002590976
7	0.002847227	0.261944884	1.002619448
8	0.002847227	100.261944884	1.002619448

Computing the price

The price with accrued interest is computed as follows:

* * * * *

16. In Appendix C, add Section II to read as follows:

Appendix C to Part 356—Investment Considerations

* * * * *

II. Floating Rate Notes

A. Interest Variability

An investment in securities with interest determined by reference to a 13-week Treasury bill index involves risks not associated with an investment in a fixed interest rate security. Such risks include the possibility that:

• Changes in the index may or may not correlate to changes in interest rates generally or with changes in other indexes;
• any given interest payment may be more or less than the amount paid on prior interest payment dates;
• the resulting interest payments may be greater or less than those payable on other securities of similar maturities, and
• in the event of sustained falling interest rates, the amount of the quarterly interest payments will decrease.

B. Trading in the Secondary Market

The Treasury securities market is the largest and most liquid securities market in the world. The market for Treasury floating rate notes, however, may not be as active or liquid as the market for Treasury non-indexed securities or Treasury inflation-protected securities. In addition, Treasury floating rate notes may not be as widely traded or as well understood as these other types of Treasury marketable securities. Prices for floating rate notes may not fluctuate in reaction to interest rate movements in the same manner as other Treasury securities. Lesser liquidity and fewer market participants may result in larger spreads between bid and asked prices for Treasury floating rate notes than the bid-asked spreads for other Treasury marketable securities with the same time to maturity. Larger bid-asked spreads normally result in higher transaction costs and/or lower overall returns. The liquidity of a Treasury floating rate note may be enhanced over time as we issue additional amounts or more entities participate in the market.

C. Tax Considerations

Treasury floating rate notes are subject to specific tax rules provided by Treasury regulations issued under section 1275(d) of the Internal Revenue Code of 1986, as amended.

D. Indexing Issues

The Bureau of the Fiscal Service publishes the High Rate immediately following a 13-week bill auction as part of the auction results. The 13-week bill is generally auctioned once per week. Treasury retains the flexibility to increase or decrease the frequency of 13-week bill auctions, which would affect the frequency of index rate resets. The High Rate is subject to various interest rate and market environments over which Treasury has no control. For a discussion of actions that Treasury would take in the event auctions of 13-week bills are discontinued or delayed, see appendix B, section I, paragraph C.4 of this part.

17. In Appendix D, revise the heading, designate the current text as section I. Consumer Price Index, and add section II to read as follows:

Appendix D to Part 356—Description of the Indexes

I. Consumer Price Index

* * * * *

II. Floating Rate Note Index

The floating rate note index is the 13-week Treasury bill auction High Rate (stop out rate), and converted to the simple-interest money market yield computed on an actual/360 basis.

Richard L. Gregg,

Fiscal Assistant Secretary.

Footnotes

1.  31 CFR part 356 is generally referred to as the Uniform Offering Circular (UOC). The UOC, together with the auction announcement for each Treasury securities auction, sets out the terms and conditions for the sale and issuance by Treasury to the public of marketable Treasury bills, notes, and bonds.

2.  The High Rate is the highest accepted discount rate in a marketable Treasury bill auction and is announced on the auction results press release. Treasury awards securities in Treasury bill auctions at the price that corresponds to the High Rate.

3.  A lockout period for floating rate notes is a period of time prior to the auction settlement or payment of interest. Any 13-week Treasury bill auction that takes place during this period will be excluded from the calculation of accrued interest for determining the settlement or interest payment amount.

4.  The May 2013 Quarterly Refunding Statement, dated May 1, 2013, can be accessed at: http://www.treasury.gov/​press-center/​press-releases/​Pages/​jl1921.aspx.

5.  The February 2012 Quarterly Refunding Statement, dated February 1, 2012, can be accessed at: http://www.treasury.gov/​press-center/​press-releases/​Pages/​tg1405.aspx.

6.  77 FR 16116 (March 19, 2012).

7.  The comment letters are available to the public for inspection and downloading at the TreasuryDirect Web site. http://www.treasurydirect.gov/​instit/​statreg/​auctreg/​auctreg_​comltr_​td_​floating rate note.htm.

8.  The August 2012 Quarterly Refunding Statement, dated August 1, 2012, can be accessed at: http://www.treasury.gov/​press-center/​press-releases/​Pages/​tg1663.aspx.

9.  77 FR 72278 (December 5, 2012).

10.  The comment letters are available to the public for inspection and downloading at the TreasuryDirect Web site. http://www.treasurydirect.gov/​instit/​statreg/​auctreg/​auctreg_​advance_​floating_​rate.htm.

11.  See § 356.20(a).

12.  Stripping means separating a security's interest and principal components so they can be traded separately.