 |
Code of Federal Regulations (Last Updated: November 8, 2024) |
 |
Title 31 - Money and Finance: Treasury |
 |
Subtitle B - Regulations Relating to Money and Finance |
 |
Chapter II - Fiscal Service, Department of the Treasury |
 |
SubChapter A - Bureau of the Fiscal Service |
 |
Part 356 - Sale and Issue of Marketable Book-Entry Treasury Bills, Notes, and Bonds (Department of the Treasury Circular, Fiscal Service Series No. 1-93) |
Appendix B to Part 356 - Formulas and Tables
-
Appendix B to Part 356 - Formulas and Tables
I. Computation of Interest on Treasury Bonds and Notes.
II. Formulas for Conversion of Non-indexed Security Yields to Equivalent Prices.
III. Formulas for Conversion of Inflation-Protected Security Yields to Equivalent Prices.
IV. Formulas for Conversion of Floating Rate Note Discount Margins to Equivalent Prices
V. Computation of Adjusted Values and Payment Amounts for Stripped Inflation-Protected Interest Components.
VI. Computation of Purchase Price, Discount Rate, and Investment Rate (Coupon-Equivalent Yield) for Treasury Bills.
The examples in this appendix are given for illustrative purposes only and are in no way a prediction of interest rates on any bills, notes, or bonds issued under this part. In some of the following examples, we use intermediate rounding for ease in following the calculations.
I. Computation of Interest on Treasury Bonds and Notes
A. Treasury Non-indexed Securities
1. Regular Half-Year Payment Period. We pay interest on marketable Treasury non-indexed securities on a semiannual basis. The regular interest payment period is a full half-year of six calendar months. Examples of half-year periods are:
(1) February 15 to August 15,
(2) May 31 to November 30, and
(3) February 29 to August 31 (in a leap year). Calculation of an interest payment for a non-indexed note with a par amount of $1,000 and an interest rate of 8% is made in this manner: ($1,000 × .08)/2 = $40. Specifically, a semiannual interest payment represents one half of one year's interest, and is computed on this basis regardless of the actual number of days in the half-year.
2. Daily Interest Decimal. We compute a daily interest decimal in cases where an interest payment period for a non-indexed security is shorter or longer than six months or where accrued interest is payable by an investor. We base the daily interest decimal on the actual number of calendar days in the half-year or half-years involved. The number of days in any half-year period is shown in Table 1.
Table 1
Interest period Beginning and ending days are 1st or 15th of the months listed under interest period
(number of days)Beginning and ending days are the last days of the months listed under interest period
(number of days)Regular year Leap year Regular year Leap year January to July 181 182 181 182 February to August 181 182 184 184 March to September 184 184 183 183 April to October 183 183 184 184 May to November 184 184 183 183 June to December 183 183 184 184 July to January 184 184 184 184 August to February 184 184 181 182 September to March 181 182 182 183 October to April 182 183 181 182 November to May 181 182 182 183 December to June 182 183 181 182 Table 2 below shows the daily interest decimals covering interest from 1⁄8% to 20% on $1,000 for one day in increments of 1⁄8 of one percent. These decimals represent 1⁄181, 1⁄182, 1⁄183, or 1⁄184 of a full semiannual interest payment, depending on which half-year is applicable.
Table 2
[Decimal for one day's interest on $1,000 at various rates of interest, payable semiannually or on a semiannual basis, in regular years of 365 days and in years of 366 days (to determine applicable number of days, see table 1.)]
Rate per annum (percent) Half-year of 184 days Half-year of 183 days Half-year of 182 days Half-year of 181 days 1⁄8 0.003396739 0.003415301 0.003434066 0.003453039 1⁄4 0.006793478 0.006830601 0.006868132 0.006906077 3⁄8 0.010190217 0.010245902 0.010302198 0.010359116 1⁄2 0.013586957 0.013661202 0.013736264 0.013812155 5⁄8 0.016983696 0.017076503 0.017170330 0.017265193 3⁄4 0.020380435 0.020491803 0.020604396 0.020718232 7⁄8 0.023777174 0.023907104 0.024038462 0.024171271 1 0.027173913 0.027322404 0.027472527 0.027624309 11⁄8 0.030570652 0.030737705 0.030906593 0.031077348 11⁄4 0.033967391 0.034153005 0.034340659 0.034530387 13⁄8 0.037364130 0.037568306 0.037774725 0.037983425 11⁄2 0.040760870 0.040983607 0.041208791 0.041436464 15⁄8 0.044157609 0.044398907 0.044642857 0.044889503 13⁄4 0.047554348 0.047814208 0.048076923 0.048342541 17⁄8 0.050951087 0.051229508 0.051510989 0.051795580 2 0.054347826 0.054644809 0.054945055 0.055248619 21⁄8 0.057744565 0.058060109 0.058379121 0.058701657 21⁄4 0.061141304 0.061475410 0.061813187 0.062154696 23⁄8 0.064538043 0.064890710 0.065247253 0.065607735 21⁄2 0.067934783 0.068306011 0.068681319 0.069060773 25⁄8 0.071331522 0.071721311 0.072115385 0.072513812 23⁄4 0.074728261 0.075136612 0.075549451 0.075966851 27⁄8 0.078125000 0.078551913 0.078983516 0.079419890 3 0.081521739 0.081967213 0.082417582 0.082872928 31⁄8 0.084918478 0.085382514 0.085851648 0.086325967 31⁄4 0.088315217 0.088797814 0.089285714 0.089779006 33⁄8 0.091711957 0.092213115 0.092719780 0.093232044 31⁄2 0.095108696 0.095628415 0.096153846 0.096685083 35⁄8 0.098505435 0.099043716 0.099587912 0.100138122 33⁄4 0.101902174 0.102459016 0.103021978 0.103591160 37⁄8 0.105298913 0.105874317 0.106456044 0.107044199 4 0.108695652 0.109289617 0.109890110 0.110497238 41⁄8 0.112092391 0.112704918 0.113324176 0.113950276 41⁄4 0.115489130 0.116120219 0.116758242 0.117403315 43⁄8 0.118885870 0.119535519 0.120192308 0.120856354 41⁄2 0.122282609 0.122950820 0.123626374 0.124309392 45⁄8 0.125679348 0.126366120 0.127060440 0.127762431 43⁄4 0.129076087 0.129781421 0.130494505 0.131215470 47⁄8 0.132472826 0.133196721 0.133928571 0.134668508 5 0.135869565 0.136612022 0.137362637 0.138121547 51⁄8 0.139266304 0.140027322 0.140796703 0.141574586 51⁄4 0.142663043 0.143442623 0.144230769 0.145027624 53⁄8 0.146059783 0.146857923 0.147664835 0.148480663 51⁄2 0.149456522 0.150273224 0.151098901 0.151933702 55⁄8 0.152853261 0.153688525 0.154532967 0.155386740 53⁄4 0.156250000 0.157103825 0.157967033 0.158839779 57⁄8 0.159646739 0.160519126 0.161401099 0.162292818 6 0.163043478 0.163934426 0.164835165 0.165745856 61⁄8 0.166440217 0.167349727 0.168269231 0.169198895 61⁄4 0.169836957 0.170765027 0.171703297 0.172651934 63⁄8 0.173233696 0.174180328 0.175137363 0.176104972 61⁄2 0.176630435 0.177595628 0.178571429 0.179558011 65⁄8 0.180027174 0.181010929 0.182005495 0.183011050 63⁄4 0.183423913 0.184426230 0.185439560 0.186464088 67⁄8 0.186820652 0.187841530 0.188873626 0.189917127 7 0.190217391 0.191256831 0.192307692 0.193370166 71⁄8 0.193614130 0.194672131 0.195741758 0.196823204 71⁄4 0.197010870 0.198087432 0.199175824 0.200276243 73⁄8 0.200407609 0.201502732 0.202609890 0.203729282 71⁄2 0.203804348 0.204918033 0.206043956 0.207182320 75⁄8 0.207201087 0.208333333 0.209478022 0.210635359 73⁄4 0.210597826 0.211748634 0.212912088 0.214088398 77⁄8 0.213994565 0.215163934 0.216346154 0.217541436 8 0.217391304 0.218579235 0.219780220 0.220994475 81⁄8 0.220788043 0.221994536 0.223214286 0.224447514 81⁄4 0.224184783 0.225409836 0.226648352 0.227900552 83⁄8 0.227581522 0.228825137 0.230082418 0.231353591 81⁄2 0.230978261 0.232240437 0.233516484 0.234806630 85⁄8 0.234375000 0.235655738 0.236950549 0.238259669 83⁄4 0.237771739 0.239071038 0.240384615 0.241712707 87⁄8 0.241168478 0.242486339 0.243818681 0.245165746 9 0.244565217 0.245901639 0.247252747 0.248618785 91⁄8 0.247961957 0.249316940 0.250686813 0.252071823 91⁄4 0.251358696 0.252732240 0.254120879 0.255524862 93⁄8 0.254755435 0.256147541 0.257554945 0.258977901 91⁄2 0.258152174 0.259562842 0.260989011 0.262430939 95⁄8 0.261548913 0.262978142 0.264423077 0.265883978 93⁄4 0.264945652 0.266393443 0.267857143 0.269337017 97⁄8 0.268342391 0.269808743 0.271291209 0.272790055 10 0.271739130 0.273224044 0.274725275 0.276243094 101⁄8 0.275135870 0.276639344 0.278159341 0.279696133 101⁄4 0.278532609 0.280054645 0.281593407 0.283149171 103⁄8 0.281929348 0.283469945 0.285027473 0.286602210 101⁄2 0.285326087 0.286885246 0.288461538 0.290055249 105⁄8 0.288722826 0.290300546 0.291895604 0.293508287 103⁄4 0.292119565 0.293715847 0.295329670 0.296961326 107⁄8 0.295516304 0.297131148 0.298763736 0.300414365 11 0.298913043 0.300546448 0.302197802 0.303867403 111⁄8 0.302309783 0.303961749 0.305631868 0.307320442 111⁄4 0.305706522 0.307377049 0.309065934 0.310773481 113⁄8 0.309103261 0.310792350 0.312500000 0.314226519 111⁄2 0.312500000 0.314207650 0.315934066 0.317679558 115⁄8 0.315896739 0.317622951 0.319368132 0.321132597 113⁄4 0.319293478 0.321038251 0.322802198 0.324585635 117⁄8 0.322690217 0.324453552 0.326236264 0.328038674 12 0.326086957 0.327868852 0.329670330 0.331491713 121⁄8 0.329483696 0.331284153 0.333104396 0.334944751 121⁄4 0.332880435 0.334699454 0.336538462 0.338397790 123⁄8 0.336277174 0.338114754 0.339972527 0.341850829 121⁄2 0.339673913 0.341530055 0.343406593 0.345303867 125⁄8 0.343070652 0.344945355 0.346840659 0.348756906 123⁄4 0.346467391 0.348360656 0.350274725 0.352209945 127⁄8 0.349864130 0.351775956 0.353708791 0.355662983 13 0.353260870 0.355191257 0.357142857 0.359116022 131⁄8 0.356657609 0.358606557 0.360576923 0.362569061 131⁄4 0.360054348 0.362021858 0.364010989 0.366022099 133⁄8 0.363451087 0.365437158 0.367445055 0.369475138 131⁄2 0.366847826 0.368852459 0.370879121 0.372928177 135⁄8 0.370244565 0.372267760 0.374313187 0.376381215 133⁄4 0.373641304 0.375683060 0.377747253 0.379834254 137⁄8 0.377038043 0.379098361 0.381181319 0.383287293 14 0.380434783 0.382513661 0.384615385 0.386740331 141⁄8 0.383831522 0.385928962 0.388049451 0.390193370 141⁄4 0.387228261 0.389344262 0.391483516 0.393646409 143⁄8 0.390625000 0.392759563 0.394917582 0.397099448 141⁄2 0.394021739 0.396174863 0.398351648 0.400552486 145⁄8 0.397418478 0.399590164 0.401785714 0.404005525 143⁄4 0.400815217 0.403005464 0.405219780 0.407458564 147⁄8 0.404211957 0.406420765 0.408653846 0.410911602 15 0.407608696 0.409836066 0.412087912 0.414364641 151⁄8 0.411005435 0.413251366 0.415521978 0.417817680 151⁄4 0.414402174 0.416666667 0.418956044 0.421270718 153⁄8 0.417798913 0.420081967 0.422390110 0.424723757 151⁄2 0.421195652 0.423497268 0.425824176 0.428176796 155⁄8 0.424592391 0.426912568 0.429258242 0.431629834 153⁄4 0.427989130 0.430327869 0.432692308 0.435082873 157⁄8 0.431385870 0.433743169 0.436126374 0.438535912 16 0.434782609 0.437158470 0.439560440 0.441988950 161⁄8 0.438179348 0.440573770 0.442994505 0.445441989 161⁄4 0.441576087 0.443989071 0.446428571 0.448895028 163⁄8 0.444972826 0.447404372 0.449862637 0.452348066 161⁄2 0.448369565 0.450819672 0.453296703 0.455801105 165⁄8 0.451766304 0.454234973 0.456730769 0.459254144 163⁄4 0.455163043 0.457650273 0.460164835 0.462707182 167⁄8 0.458559783 0.461065574 0.463598901 0.466160221 17 0.461956522 0.464480874 0.467032967 0.469613260 171⁄8 0.465353261 0.467896175 0.470467033 0.473066298 171⁄4 0.468750000 0.471311475 0.473901099 0.476519337 173⁄8 0.472146739 0.474726776 0.477335165 0.479972376 171⁄2 0.475543478 0.478142077 0.480769231 0.483425414 175⁄8 0.478940217 0.481557377 0.484203297 0.486878453 173⁄4 0.482336957 0.484972678 0.487637363 0.490331492 177⁄8 0.485733696 0.488387978 0.491071429 0.493784530 18 0.489130435 0.491803279 0.494505495 0.497237569 181⁄8 0.492527174 0.495218579 0.497939560 0.500690608 181⁄4 0.495923913 0.498633880 0.501373626 0.504143646 183⁄8 0.499320652 0.502049180 0.504807692 0.507596685 181⁄2 0.502717391 0.505464481 0.508241758 0.511049724 185⁄8 0.506114130 0.508879781 0.511675824 0.514502762 183⁄4 0.509510870 0.512295082 0.515109890 0.517955801 187⁄8 0.512907609 0.515710383 0.518543956 0.521408840 19 0.516304348 0.519125683 0.521978022 0.524861878 191⁄8 0.519701087 0.522540984 0.525412088 0.528314917 191⁄4 0.523097826 0.525956284 0.528846154 0.531767956 193⁄8 0.526494565 0.529371585 0.532280220 0.535220994 191⁄2 0.529891304 0.532786885 0.535714286 0.538674033 195⁄8 0.533288043 0.536202186 0.539148352 0.542127072 193⁄4 0.536684783 0.539617486 0.542582418 0.545580110 197⁄8 0.540081522 0.543032787 0.546016484 0.549033149 20 0.543478261 0.546448087 0.549450549 0.552486188 3. Short First Payment Period. In cases where the first interest payment period for a Treasury non-indexed security covers less than a full half-year period (a “short coupon”), we multiply the daily interest decimal by the number of days from, but not including, the issue date to, and including, the first interest payment date. This calculation results in the amount of the interest payable per $1,000 par amount. In cases where the par amount of securities is a multiple of $1,000, we multiply the appropriate multiple by the unrounded interest payment amount per $1,000 par amount.
Example
A 2-year note paying 83⁄8% interest was issued on July 2, 1990, with the first interest payment on December 31, 1990. The number of days in the full half-year period of June 30 to December 31, 1990, was 184 (See Table 1.). The number of days for which interest actually accrued was 182 (not including July 2, but including December 31). The daily interest decimal, $0.227581522 (See Table 2, line for 83⁄8%, under the column for half-year of 184 days.), was multiplied by 182, resulting in a payment of $41.419837004 per $1,000. For $20,000 of these notes, $41.419837004 would be multiplied by 20, resulting in a payment of $828.39674008 ($828.40).
4. Long First Payment Period. In cases where the first interest payment period for a bond or note covers more than a full half-year period (a “long coupon”), we multiply the daily interest decimal by the number of days from, but not including, the issue date to, and including, the last day of the fractional period that ends one full half-year before the interest payment date. We add that amount to the regular interest amount for the full half-year ending on the first interest payment date, resulting in the amount of interest payable for $1,000 par amount. In cases where the par amount of securities is a multiple of $1,000, the appropriate multiple should be applied to the unrounded interest payment amount per $1,000 par amount.
Example
A 5-year 2-month note paying 77⁄8% interest was issued on December 3, 1990, with the first interest payment due on August 15, 1991. Interest for the regular half-year portion of the payment was computed to be $39.375 per $1,000 par amount. The fractional portion of the payment, from December 3 to February 15, fell in a 184-day half-year (August 15, 1990, to February 15, 1991). Accordingly, the daily interest decimal for 77⁄8% was $0.213994565. This decimal, multiplied by 74 (the number of days from but not including December 3, 1990, to and including February 15), resulted in interest for the fractional portion of $15.835597810. When added to $39.375 (the normal interest payment portion ending on August 15, 1991), this produced a first interest payment of $55.210597810, or $55.21 per $1,000 par amount. For $7,000 par amount of these notes, $55.210597810 would be multiplied by 7, resulting in an interest payment of $386.474184670 ($386.47).
B. Treasury Inflation-Protected Securities
1. Indexing Process. We pay interest on marketable Treasury inflation-protected securities on a semiannual basis. We issue inflation-protected securities with a stated rate of interest that remains constant until maturity. Interest payments are based on the security's inflation-adjusted principal at the time we pay interest. We make this adjustment by multiplying the par amount of the security by the applicable Index Ratio.
2. Index Ratio. The numerator of the Index Ratio, the Ref CPIDate, is the index number applicable for a specific day. The denominator of the Index Ratio is the Ref CPI applicable for the original issue date. However, when the dated date is different from the original issue date, the denominator is the Ref CPI applicable for the dated date. The formula for calculating the Index Ratio is:

Where Date = valuation date
3. Reference CPI. The Ref CPI for the first day of any calendar month is the CPI for the third preceding calendar month. For example, the Ref CPI applicable to April 1 in any year is the CPI for January, which is reported in February. We determine the Ref CPI for any other day of a month by a linear interpolation between the Ref CPI applicable to the first day of the month in which the day falls (in the example, January) and the Ref CPI applicable to the first day of the next month (in the example, February). For interpolation purposes, we truncate calculations with regard to the Ref CPI and the Index Ratio for a specific date to six decimal places, and round to five decimal places.
Therefore the Ref CPI and the Index Ratio for a particular date will be expressed to five decimal places.
(i) The formula for the Ref CPI for a specific date is:
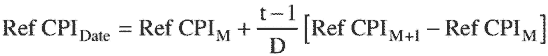
Where Date = valuation date
D = the number of days in the month in which Date falls
t = the calendar day corresponding to Date
CPIM = CPI reported for the calendar month M by the Bureau of Labor Statistics
Ref CPIM = Ref CPI for the first day of the calendar month in which Date falls, e.g., Ref CPIApril1 is the CPIJanuary
Ref CPIM + 1 = Ref CPI for the first day of the calendar month immediately following Date
(ii) For example, the Ref CPI for April 15, 1996 is calculated as follows:
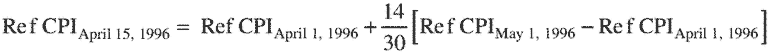
where D = 30, t = 15
Ref CPIApril 1, 1996 = 154.40, the non-seasonally adjusted CPI-U for January 1996.
Ref CPIMay 1, 1996 = 154.90, the non-seasonally adjusted CPI-U for February 1996.
(iii) Putting these values in the equation in paragraph (ii) above:

This value truncated to six decimals is 154.633333; rounded to five decimals it is 154.63333.
(iv) To calculate the Index Ratio for April 16, 1996, for an inflation-protected security issued on April 15, 1996, the Ref CPIApril 16, 1996 must first be calculated. Using the same values in the equation above except that t = 16, the Ref CPIApril 16, 1996 is 154.65000.
The Index Ratio for April 16, 1996 is:
Index RatioApril 16, 1996 = 154.65000/154.63333 = 1.000107803.
This value truncated to six decimals is 1.000107; rounded to five decimals it is 1.00011.
4. Index Contingencies.
(i) If a previously reported CPI is revised, we will continue to use the previously reported (unrevised) CPI in calculating the principal value and interest payments.
If the CPI is rebased to a different year, we will continue to use the CPI based on the base reference period in effect when the security was first issued, as long as that CPI continues to be published.
(ii) We will replace the CPI with an appropriate alternative index if, while an inflation-protected security is outstanding, the applicable CPI is:
• Discontinued,
• In the judgment of the Secretary, fundamentally altered in a manner materially adverse to the interests of an investor in the security, or
• In the judgment of the Secretary, altered by legislation or Executive Order in a manner materially adverse to the interests of an investor in the security.
(iii) If we decide to substitute an alternative index we will consult with the Bureau of Labor Statistics or any successor agency. We will then notify the public of the substitute index and how we will apply it. Determinations of the Secretary in this regard will be final.
(iv) If the CPI for a particular month is not reported by the last day of the following month, we will announce an index number based on the last available twelve-month change in the CPI. We will base our calculations of our payment obligations that rely on that month's CPI on the index number we announce.
(a) For example, if the CPI for month M is not reported timely, the formula for calculating the index number to be used is:
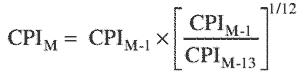
(c) If it is necessary to use these formulas to calculate an index number, we will use that number for all subsequent calculations that rely on the month's index number. We will not replace it with the actual CPI when it is reported, except for use in the above formulas. If it becomes necessary to use the above formulas to derive an index number, we will use the last CPI that has been reported to calculate CPI numbers for months for which the CPI has not been reported timely.
5. Computation of Interest for a Regular Half-Year Payment Period. Interest on marketable Treasury inflation-protected securities is payable on a semiannual basis. The regular interest payment period is a full half-year or six calendar months. Examples of half-year periods are January 15 to July 15, and April 15 to October 15. An interest payment will be a fixed percentage of the value of the inflation-adjusted principal, in current dollars, for the date on which it is paid. We will calculate interest payments by multiplying one-half of the specified annual interest rate for the inflation-protected securities by the inflation-adjusted principal for the interest payment date.
Specifically, we compute a semiannual interest payment on the basis of one-half of one year's interest regardless of the actual number of days in the half-year.
Example
A 10-year inflation-protected note paying 37⁄8% interest was issued on January 15, 1999, with the first interest payment on July 15, 1999. The Ref CPI on January 15, 1999 (Ref CPIIssueDate) was 164, and the Ref CPI on July 15, 1999 (Ref CPIDate) was 166.2. For a par amount of $100,000, the inflation-adjusted principal on July 15, 1999, was (166.2/164) × $100,000, or $101,341. This amount was multiplied by .03875/2, or .019375, resulting in a payment of $1,963.48.
C. Treasury Floating Rate Notes
1. Indexing and Interest Payment Process. We issue floating rate notes with a daily interest accrual feature. This means that the interest rate “floats” based on changes in the representative index rate. We pay interest on a quarterly basis. The index rate is the High Rate of the 13-week Treasury bill auction announced on the auction results press release that has been converted into a simple-interest money market yield computed on an actual/360 basis and rounded to nine decimal places. Interest payments are based on the floating rate note's variable interest rate from, and including, the dated date or last interest payment date to, but excluding, the next interest payment or maturity date. We make quarterly interest payments by accruing the daily interest amounts and adding those amounts together for the interest payment period.
2. Interest Rate. The interest rate on floating rate notes will be the spread plus the index rate (as it may be adjusted on the calendar day following each auction of 13-week bills).
3. Interest Accrual. In general, accrued interest for a particular calendar day in an accrual period is calculated by using the index rate from the most recent auction of 13-week bills that took place before the accrual day, plus the spread determined at the time of a new floating rate note auction, divided by 360, subject to a zero-percent minimum daily interest accrual rate. However, a 13-week bill auction that takes place in the two-business-day period prior to a settlement date or interest payment date will be excluded from the calculation of accrued interest for purposes of the settlement amount or interest payment. Any changes in the index rate that would otherwise have occurred during this two-business-day period will occur on the first calendar day following the end of the period.
4. Index Contingencies.
(i) If Treasury were to discontinue auctions of 13-week bills, the Secretary has authority to determine and announce a new index for outstanding floating rate notes.
(ii) If Treasury were to not conduct a 13-week bill auction in a particular week, then the interest rate in effect for the notes at the time of the last 13-week bill auction results announcement will remain in effect until such time, if any, as the results of a 13-week Treasury auction are again announced by Treasury. Treasury reserves the right to change the index rate for any newly issued floating rate note.
D. Accrued Interest
1. You will have to pay accrued interest on a Treasury bond or note when interest accrues prior to the issue date of the security. Because you receive a full interest payment despite having held the security for only a portion of the interest payment period, you must compensate us through the payment of accrued interest at settlement.
2. For a Treasury non-indexed security, if accrued interest covers a fractional portion of a full half-year period, the number of days in the full half-year period and the stated interest rate will determine the daily interest decimal to use in computing the accrued interest. We multiply the decimal by the number of days for which interest has accrued.
3. If a reopened bond or note has a long first interest payment period (a “long coupon”), and the dated date for the reopened issue is less than six full months before the first interest payment, the accrued interest will fall into two separate half-year periods. A separate daily interest decimal must be multiplied by the respective number of days in each half-year period during which interest has accrued.
4. We round all accrued interest computations to five decimal places for a $1,000 par amount, using normal rounding procedures. We calculate accrued interest for a par amount of securities greater than $1,000 by applying the appropriate multiple to accrued interest payable for a $1,000 par amount, rounded to five decimal places. We calculate accrued interest for a par amount of securities less than $1,000 by applying the appropriate fraction to accrued interest payable for a $1,000 par amount, rounded to five decimal places.
5. For an inflation-protected security, we calculate accrued interest as shown in section III, paragraphs A and B of this appendix.
Examples:
(1) Treasury Non-indexed Securities - (i) Involving One Half-Year: A note paying interest at a rate of 63⁄4%, originally issued on May 15, 2000, as a 5-year note with a first interest payment date of November 15, 2000, was reopened as a 4-year 9-month note on August 15, 2000. Interest had accrued for 92 days, from May 15 to August 15. The regular interest period from May 15 to November 15, 2000, covered 184 days. Accordingly, the daily interest decimal, $0.183423913, multiplied by 92, resulted in accrued interest payable of $16.874999996, or $16.87500, for each $1,000 note purchased. If the notes have a par amount of $150,000, then 150 is multiplied by $16.87500, resulting in an amount payable of $2,531.25.
(2) Involving Two Half-Years:
A 103⁄4% bond, originally issued on July 2, 1985, as a 20-year 1-month bond, with a first interest payment date of February 15, 1986, was reopened as a 19-year 10-month bond on November 4, 1985. Interest had accrued for 44 days, from July 2 to August 15, 1985, during a 181-day half-year (February 15 to August 15); and for 81 days, from August 15 to November 4, during a 184-day half-year (August 15, 1985, to February 15, 1986). Accordingly, $0.296961326 was multiplied by 44, and $0.292119565 was multiplied by 81, resulting in products of $13.066298344 and $23.661684765 which, added together, resulted in accrued interest payable of $36.727983109, or $36.72798, for each $1,000 bond purchased. If the bonds have a par amount of $11,000, then 11 is multiplied by $36.72798, resulting in an amount payable of $404.00778 ($404.01).
6. For a floating rate note, if accrued interest covers a portion of a full quarterly interest payment period, we calculate accrued interest as shown in section IV, paragraphs C and D of this appendix.
II. Formulas for Conversion of Non-indexed Security Yields to Equivalent Prices
Definitions
P = price per 100 (dollars), rounded to six places, using normal rounding procedures.
C = the regular annual interest per $100, payable semiannually, e.g., 6.125 (the decimal equivalent of a 61⁄8% interest rate).
i = nominal annual rate of return or yield to maturity, based on semiannual interest payments and expressed in decimals, e.g., .0719.
n = number of full semiannual periods from the issue date to maturity, except that, if the issue date is a coupon frequency date, n will be one less than the number of full semiannual periods remaining to maturity. Coupon frequency dates are the two semiannual dates based on the maturity date of each note or bond issue. For example, a security maturing on November 15, 2015, would have coupon frequency dates of May 15 and November 15.
r = (1) number of days from the issue date to the first interest payment (regular or short first payment period), or (2) number of days in fractional portion (or “initial short period”) of long first payment period.
s = (1) number of days in the full semiannual period ending on the first interest payment date (regular or short first payment period), or (2) number of days in the full semiannual period in which the fractional portion of a long first payment period falls, ending at the onset of the regular portion of the first interest payment.
vn = 1 / [1 + (i/2)]n = present value of 1 due at the end of n periods.
an = (1 − vn) / (i/2) = v + v2 + v3 + ... + vn = present value of 1 per period for n periods
Special Case: If i = 0, then an⌉ = n. Furthermore, when i = 0, an⌉ cannot be calculated using the formula: (1 − vn)/(i/2). In the special case where i = 0, an⌉ must be calculated as the summation of the individual present values (i.e., v + v2 + v3 + ... + vn). Using the summation method will always confirm that an⌉ = n when i = 0.
A = accrued interest.
A. For non-indexed securities with a regular first interest payment period:
Formula:
P[1 + (r/s)(i/2)] = (C/2)(r/s) + (C/2)an⌉ + 100vn.
Example:
For an 83⁄4% 30-year bond issued May 15, 1990, due May 15, 2020, with interest payments on November 15 and May 15, solve for the price per 100 (P) at a yield of 8.84%.
Definitions:G12752
C = 8.75.
i = .0884.
r = 184 (May 15 to November 15, 1990).
s = 184 (May 15 to November 15, 1990).
n = 59 (There are 60 full semiannual periods, but n is reduced by 1 because the issue date is a coupon frequency date.)
vn = 1 / [(1 + .0884 / 2)]59, or .0779403508.
an⌉ = (1 − .0779403508) / .0442, or 20.8610780353.
Resolution:
P[1 + (r/s)(i/2)] = (C/2)(r/s) + (C/2)an⌉ + 100vn or
P[1 + (184/184)(.0884/2)] = (8.75/2)(184/184) + (8.75/2)(20.8610780353) + 100(.0779403508).
(1) P[1 + .0442] = 4.375 + 91.2672164044 + 7.7940350840.
(2) P[1.0442] = 103.4362514884.
(3) P = 103.4362514884 / 1.0442.
(4) P = 99.057893.
B. For non-indexed securities with a short first interest payment period:
Formula:
P[1 + (r/s)(i/2)] = (C/2)(r/s) + (C/2)an⌉ + 100vn.
Example:
For an 81⁄2% 2-year note issued April 2, 1990, due March 31, 1992, with interest payments on September 30 and March 31, solve for the price per 100 (P) at a yield of 8.59%.
Definitions:
C = 8.50.
i = .0859.
n = 3.
r = 181 (April 2 to September 30, 1990).
s = 183 (March 31 to September 30, 1990).
vn = 1 / [(1 + .0859 / 2)]3, or .8814740565.
an⌉ = (1 − .8814740565) / .04295, or 2.7596261590.
Resolution:
P[1 + (r/s)(i/2)] = (C/2)(r/s) + (C/2)an⌉ + 100vn or
P[1 + (181/183)(.0859/2)] = (8.50/2)(181/183) + (8.50/2)(2.7596261590) + 100(.8814740565).
(1) P[1 + .042480601] = 4.2035519126 + 11.7284111757 + 88.14740565.
(2) P[1.042480601] = 104.0793687354.
(3) P = 104.0793687354 / 1.042480601.
(4) P = 99.838183.
C. For non-indexed securities with a long first interest payment period:
Formula:
P[1 + (r/s)(i/2)] = [(C/2)(r/s)]v + (C/2)an⌉ + 100vn.
Example:
For an 81⁄2% 5-year 2-month note issued March 1, 1990, due May 15, 1995, with interest payments on November 15 and May 15 (first payment on November 15, 1990), solve for the price per 100 (P) at a yield of 8.53%.
Definitions:
C = 8.50.
i = .0853.
n = 10.
r = 75 (March 1 to May 15, 1990, which is the fractional portion of the first interest payment).
s = 181 (November 15, 1989, to May 15, 1990).
v = 1 / (1 + .0853/2), or .9590946147.
vn = 1 / (1 + .0853/2)10, or .658589
an⌉ = (1−.658589)/.04265, or 8.0049454082.
Resolution:
P[1 + (r/s)(i/2)] = [(C/2)(r/s)]v + (C/2)an⌉ + 100vn or
P[1 + (75/181)(.0853/2)] = [(8.50/2)(75/181)].9590946147 + (8.50/2)(8.0049454082) + 100(.6585890783).
(1) P[1 + .017672652] = 1.6890133062 + 34.0210179850 + 65.8589078339.
(2) P[1.017672652] = 101.5689391251.
(3) P = 101.5689391251 / 1.017672652.
(4) P = 99.805118.
D.
(1) For non-indexed securities reopened during a regular interest period where the purchase price includes predetermined accrued interest.
(2) For new non-indexed securities accruing interest from the coupon frequency date immediately preceding the issue date, with the interest rate established in the auction being used to determine the accrued interest payable on the issue date.
Formula:
(P + A)[1 + (r/s)(i/2)] = C/2 + (C/2)an⌉ + 100vn.
Where:
A = [(s−r)/s](C/2).
Example:
For a 91⁄2% 10-year note with interest accruing from November 15, 1985, issued November 29, 1985, due November 15, 1995, and interest payments on May 15 and November 15, solve for the price per 100 (P) at a yield of 9.54%. Accrued interest is from November 15 to November 29 (14 days).
Definitions:
C = 9.50.
i = .0954.
n = 19.
r = 167 (November 29, 1985, to May 15, 1986).
s = 181 (November 15, 1985, to May 15, 1986).
vn = 1 / [(1 + .0954/2)]19, or .4125703996.
an⌉ = (1 − .4125703996) / .0477, or 12.3150859630.
A = [(181 − 167) / 181](9.50/2), or .367403.
Resolution:
(P + A)[1 + (r/s)(i/2)] = C/2 + (C/2)an⌉ + 100vn or
(P + .367403)[1 + (167/181)(.0954/2)] = (9.50/2) + (9.50/2)(12.3150859630) + 100(.4125703996).
(1) (P + .367403)[1 + .044010497] = 4.75 + 58.4966583243 + 41.25703996.
(2) (P + .367403)[1.044010497] = 104.5036982843.
(3) (P + .367403) = 104.5036982843 / 1.044010497.
(4) (P + .367403) = 100.098321.
(5) P = 100.098321 −.367403.
(6) P = 99.730918.
E. For non-indexed securities reopened during the regular portion of a long first payment period:
Formula:
(P + A)[1 + (r/s)(i/2)] = (r′s″)(C/2) + C 2 + (C/2)an⌉ + 100vn.
Where:
A = AI′ + AI,
AI′ = (r′/s″)(C/2),
AI = [(s−r) / s](C/2), and
r = number of days from the reopening date to the first interest payment date,
s = number of days in the semiannual period for the regular portion of the first interest payment period,
r′ = number of days in the fractional portion (or “initial short period”) of the first interest payment period,
s″ = number of days in the semiannual period ending with the commencement date of the regular portion of the first interest payment period.
Example:
A 103⁄4% 19-year 9-month bond due August 15, 2005, is issued on July 2, 1985, and reopened on November 4, 1985, with interest payments on February 15 and August 15 (first payment on February 15, 1986), solve for the price per 100 (P) at a yield of 10.47%. Accrued interest is calculated from July 2 to November 4.
Definitions:
C = 10.75.
i = .1047.
n = 39.
r = 103 (November 4, 1985, to February 15, 1986).
s = 184 (August 15, 1985, to February 15, 1986).
r′ = 44 (July 2 to August 15, 1985).
s″ = 181 (February 15 to August 15, 1985).
vn = 1 / [(1 + .1047 / 2)]39, or .1366947986.
an⌉ = (1 − .1366947986) / .05235, or 16.4910258142.
AI′ = (44 / 181)(10.75 / 2), or 1.306630.
AI = [(184 − 103) / 184](10.75 / 2), or 2.366168.
A = AI′ + AI, or 3.672798.
Resolution:
(P + A)[1 + (r/s)(i/2)] = (r′/s″)(C/2) + C/2 + (C/2)an⌉ + 100vn or
(P + 3.672798)[1 + (103/184)(.1047/2)] = (44/181)(10.75/2) + 10.75/2 + (10.75/2)(16.4910258142) + 100(.1366947986).
(1) (P + 3.672798)[1 + .02930462] = 1.3066298343 + 5.375 + 88.6392637512 + 13.6694798628.
(2) (P + 3.672798)[1.02930462] = 108.9903734482.
(3) (P + 3.672798) = 108.9903734482 / 1.02930462.
(4) (P + 3.672798) = 105.887384.
(5) P = 105.887384 −3.672798.
(6) P = 102.214586.
F. For non-indexed securities reopened during a short first payment period:
Formula:
(P + A)[1 + (r/s)(i/2)] = (r′/s)(C/2) + (C/2)an⌉ + 100vn.
Where:
A = [(r′ − r)/s](C/2) and
r′ = number of days from the original issue date to the first interest payment date.
Example:
For a 101⁄2% 8-year note due May 15, 1991, originally issued on May 16, 1983, and reopened on August 15, 1983, with interest payments on November 15 and May 15 (first payment on November 15, 1983), solve for the price per 100 (P) at a yield of 10.53%. Accrued interest is calculated from May 16 to August 15.
Definitions:
C = 10.50.
i = .1053.
n = 15.
r = 92 (August 15, 1983, to November 15, 1983).
s = 184 (May 15, 1983, to November 15, 1983).
r′ = 183 (May 16, 1983, to November 15, 1983).
vn = 1/[(1 + .1053/2)]15, or .4631696332.
an⌉ = (1 − .4631696332) / .05265, or 10.1962082956.
A = [(183 − 92) / 184](10.50 / 2), or 2.596467.
Resolution:
(P + A)[1 + (r/s)(i/2)] = (r′/s)(C/2) + (C/2)an⌉ + 100vn or
(P + 2.596467)[1 + (92/184)(.1053/2)] = (183/184)(10.50/2) + (10.50/2)(10.1962082956) + 100(.4631696332).
(1) (P + 2.596467)[1 + .026325] = 5.2214673913 + 53.5300935520 + 46.31696332.
(2) (P + 2.596467)[1.026325] = 105.0685242633.
(3) (P + 2.596467) = 105.0685242633 / 1.026325.
(4) (P + 2.596467) = 102.373541.
(5) P = 102.373541 − 2.596467.
(6) P = 99.777074.
G. For non-indexed securities reopened during the fractional portion (initial short period) of a long first payment period:
Formula:
(P + A)[1 + (r/s)(i/2)] = [(r′/s)(C/2)]v + (C/2)an⌉ + 100vn.
Where:
A = [(r′ − r)/s](C/2), and
r = number of days from the reopening date to the end of the short period.
r′ = number of days in the short period.
s = number of days in the semiannual period ending with the end of the short period.
Example:
For a 93⁄4% 6-year 2-month note due December 15, 1994, originally issued on October 15, 1988, and reopened on November 15, 1988, with interest payments on June 15 and December 15 (first payment on June 15, 1989), solve for the price per 100 (P) at a yield of 9.79%. Accrued interest is calculated from October 15 to November 15.
Definitions:
C = 9.75.
i = .0979.
n = 12.
r = 30 (November 15, 1988, to December 15, 1988).
s = 183 (June 15, 1988, to December 15, 1988).
r′ = 61 (October 15, 1988, to December 15, 1988).
v = 1 / (1 + .0979/2), or .9533342867.
vn = [1 / (1 + .0979/2)]12, or .5635631040.
an⌉ = (1 − .5635631040)/.04895, or 8.9159733613.
A = [(61 − 30)/183](9.75/2), or .825820.
Resolution:
(P + A)[1 + (r/s)(i/2)] = [(r′/s)(C/2)]v + (C/2)an⌉ + 100vn or
(P + .825820)[1 + (30/183)(.0979/2)] = [(61/183)(9.75/2)](.9533342867) + (9.75/2)(8.9159733613) + 100(.5635631040).
(1) (P + .825820)[1 + .00802459] = 1.549168216 + 43.4653701362 + 56.35631040.
(2) (P + .825820)[1.00802459] = 101.3708487520.
(3) (P + .825820) = 101.3708487520 / 1.00802459.
(4) (P + .825820) = 100.563865.
(5) P = 100.563865 −. 825820.
(6) P = 99.738045.
III. Formulas for Conversion of Inflation-Indexed Security Yields to Equivalent Prices
Definitions
P = unadjusted or real price per 100 (dollars).
Padj = inflation adjusted price; P × Index RatioDate.
A = unadjusted accrued interest per $100 original principal.
Aadj = inflation adjusted accrued interest; A × Index RatioDate.
SA = settlement amount including accrued interest in current dollars per $100 original principal; Padj + Aadj.
r = days from settlement date to next coupon date.
s = days in current semiannual period.
i = real yield, expressed in decimals (e.g., 0.0325).
C = real annual coupon, payable semiannually, in terms of real dollars paid on $100 initial, or real, principal of the security.
n = number of full semiannual periods from issue date to maturity date, except that, if the issue date is a coupon frequency date, n will be one less than the number of full semiannual periods remaining until maturity. Coupon frequency dates are the two semiannual dates based on the maturity date of each note or bond issue. For example, a security maturing on July 15, 2026 would have coupon frequency dates of January 15 and July 15.
vn = 1/(1 + i/2)n = present value of 1 due at the end of n periods.
an⌉ = (1 − vn) / (i/2) = v + v2 + v3 +... + vn = present value of 1 per period for n periods.
Special Case: If i = 0, then an⌉ = n. Furthermore, when i = 0, an⌉ cannot be calculated using the formula: (1 − vn)/(i/2). In the special case where i = 0, an⌉ must be calculated as the summation of the individual present values (i.e., v + v2 + v3 +... + vn). Using the summation method will always confirm that an⌉ = n when i = 0.
Date = valuation date.
D = the number of days in the month in which Date falls.
t = calendar day corresponding to Date.
CPI = Consumer Price Index number.
CPIM = CPI reported for the calendar month M by the Bureau of Labor Statistics.
Ref CPIM = reference CPI for the first day of the calendar month in which Date falls (also equal to the CPI for the third preceding calendar month), e.g., Ref CPIApril 1 is the CPIJanuary.
Ref CPIM + 1 = reference CPI for the first day of the calendar month immediately following Date.
Ref CPIDate = Ref CPIM − [(t − 1)/D][Ref CPIM + 1-Ref CPIM].
Index RatioDate = Ref CPIDate / Ref CPIIssueDate.
Note:When the Issue Date is different from the Dated Date, the denominator is the Ref CPIDatedDate.
A. For inflation-indexed securities with a regular first interest payment period:
Formulas:

Padj = P × Index RatioDate.
A = [(s−r)/s] × (C/2).
Aadj = A × Index RatioDate.
SA = Padj + Aadj
Index RatioDate = Ref CPIDate/Ref CPIIssueDate.
Example:
We issued a 10-year inflation-indexed note on January 15, 1999. The note was issued at a discount to yield of 3.898% (real). The note bears a 37⁄8% real coupon, payable on July 15 and January 15 of each year. The base CPI index applicable to this note is 164. (We normally derive this number using the interpolative process described in appendix B, section I, paragraph B.)
Definitions:
C = 3.875.
i = 0.03898.
n = 19 (There are 20 full semiannual periods but n is reduced by 1 because the issue date is a coupon frequency date.).
r = 181 (January 15, 1999 to July 15, 1999).
s = 181 (January 15, 1999 to July 15, 1999).
Ref CPIDate = 164.
Ref CPIIssueDate = 164.
Resolution:
Index RatioDate = Ref CPIDate / Ref CPIIssueDate = 164/164 = 1.
A = [(181 − 181)/181] × 3.875/2 = 0.
Aadj = 0 × 1 = 0.
vn = 1/(1 + i/2)n = 1/(1 + .03898/2)19 = 0.692984572.
an⌉ = (1 − vn)/(i/2) = (1-0.692984572) / (.03898/2) = 15.752459107.
Formula:

P = 99.811030.
Padj = P × Index RatioDate.
Padj = 99.811030 × 1 = 99.811030.
SA = Padj × Aadj.
SA = 99.811030 + 0 = 99.811030.
Note:For the real price (P), we have rounded to six places. These amounts are based on 100 par value.
B.
(1) For inflation-indexed securities reopened during a regular interest period where the purchase price includes predetermined accrued interest.
(2) For new inflation-indexed securities accruing interest from the coupon frequency date immediately preceding the issue date, with the interest rate established in the auction being used to determine the accrued interest payable on the issue date.
Bidding: The dollar amount of each bid is in terms of the par amount. For example, if the Ref CPI applicable to the issue date of the note is 120, and the reference CPI applicable to the reopening issue date is 132, a bid of $10,000 will in effect be a bid of $10,000 × (132/120), or $11,000.
Formulas:

Padj = P × Index RatioDate.
A = [(s−r)/s] × (C/2).
Aadj = A × Index RatioDate.
SA = Padj + Aadj.
Index RatioDate = Ref CPIDate/Ref CPIIssueDate.
Example:
We issued a 35⁄8% 10-year inflation-indexed note on January 15, 1998, with interest payments on July 15 and January 15. For a reopening on October 15, 1998, with inflation compensation accruing from January 15, 1998 to October 15, 1998, and accrued interest accruing from July 15, 1998 to October 15, 1998 (92 days), solve for the price per 100 (P) at a real yield, as determined in the reopening auction, of 3.65%. The base index applicable to the issue date of this note is 161.55484 and the reference CPI applicable to October 15, 1998, is 163.29032.
Definitions:
C = 3.625.
i = 0.0365.
n = 18.
r = 92 (October 15, 1998 to January 15, 1999).
s = 184 (July 15, 1998 to January 15, 1999).
Ref CPIDate = 163.29032.
Ref CPIIssueDate = 161.55484.
Resolution:
Index RatioDate = Ref CPIDate/Ref CPIIssueDate = 163.29032/161.55484 = 1.01074.
vn = 1/(1 + i/2)n = 1/(1 + .0365/2)18 = 0.722138438.
an⌉ = (1−vn)/(i/2) = (1 − 0.722138438)/(.0365/2) = 15.225291068.
Formula:

P = 100.703267 − 0.906250.
P = 99.797017.
Padj = P × Index RatioDate.
Padj = 99.797017 × 1.01074 = 100.86883696.
Padj = 100.868837.
A = [(184−92)/184] × 3.625/2 = 0.906250.
Aadj = A × Index RatioDate.
Aadj = 0.906250 × 1.01074 = 0.91598313.
Aadj = 0.915983.
SA = Padj + Aadj = 100.868837 + 0.915983.
SA = 101.784820.
Note:For the real price (P), and the inflation-adjusted price (Padj), we have rounded to six places. For accrued interest (A) and the adjusted accrued interest (Aadj), we have rounded to six places. These amounts are based on 100 par value.
IV. Formulas for Conversion of Floating Rate Note Discount Margins to Equivalent Prices
Definitions for Newly Issued Floating Rate Notes
P = the price per $100 par value.
T0 = the issue date.
N = the total number of quarterly interest payments.
i and k = indexes that identify the sequence of interest payment dates.
Ti = the ith quarterly interest payment date.
Ti − Ti-1 = the number of days between the interest payment date Ti and the preceding interest payment date.
TN = the maturity date.
r = the index rate applicable to the issue date.
s = the spread.
m = the discount margin.
A. For newly issued floating rate notes issued at par:
Formula:
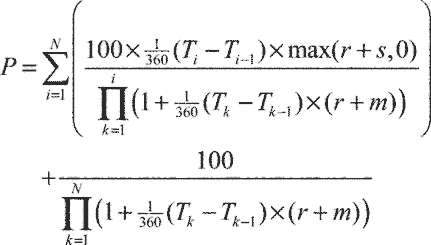
Example:
The purpose of this example is to demonstrate how a floating rate note price is derived at the time of original issuance. Additionally, this example depicts the association of the July 31, 2012 issue date and the two-business-day lockout period. For a new two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, and an interest accrual rate on the issue date of 0.215022819% (index rate of 0.095022819% plus a spread of 0.120%), solve for the price per 100 (P). This interest accrual rate is used for each daily interest accrual over the life of the security for the purposes of this example. In a new issuance (not a reopening) of a floating rate note, the discount margin determined at auction will be equal to the spread.
Definitions:
T0 = July 31, 2012.
N = 8.
TN = July 31, 2014.
r = 0.095022819%.
s = 0.120%.
m = 0.120%.
As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:
Table 1 - 13-Week Bill Auction Data
Auction date Issue date Maturity date Auction
clearing priceAuction high rate Index rate 7/23/2012 7/26/2012 10/25/2012 99.975986 0.095% 0.095022819% The rationale for using a 13-week bill auction that has occurred at least two days prior to the issue date is due to the two-business-day lockout period. This lockout period applies only to the issue date and interest payment dates, thus any 13-week bill auction that occurs during the two-day lockout period is not used for calculations related to the issue date and interest payment dates. The following sample calendar depicts this relationship for the floating rate note issue date.
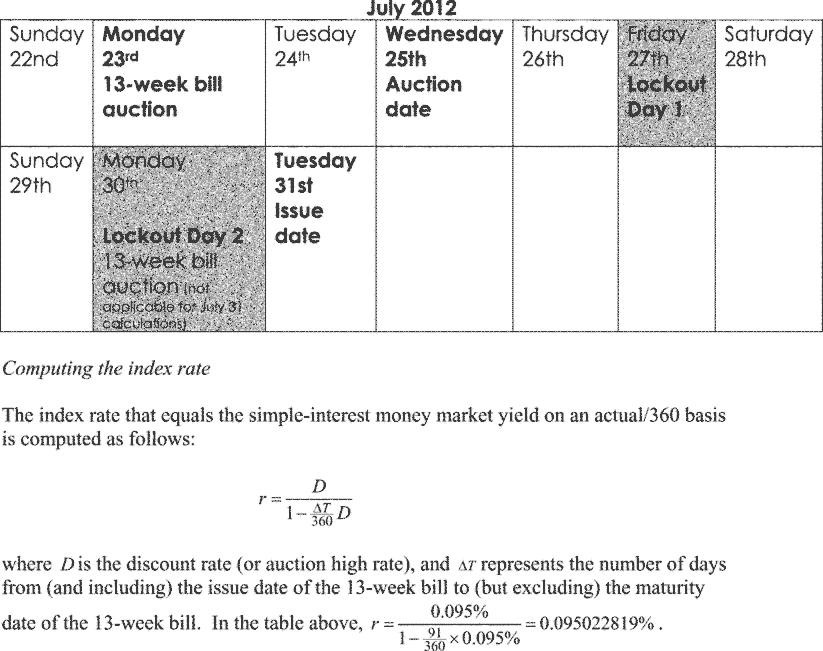
Computing the Projected Cash Flows
The following table presents the future interest payment dates and the number of days between them.
Table 2 - Payment Dates
Dates Days between dates Issue Date: T0 = 7/31/2012 1st Interest Date: T1 = 10/31/2012 T1 − T0 = 92 2nd Interest Date: T2 = 1/31/2013 T2 − T1 = 92 3rd Interest Date: T3 = 4/30/2013 T3 − T2 = 89 4th Interest Date: T4 = 7/31/2013 T4 − T3 = 92 5th Interest Date: T5 = 10/31/2013 T5 − T4 = 92 6th Interest Date: T6 = 1/31/2014 T6 − T5 = 92 7th Interest Date: T7 = 4/30/2014 T7 − T6 = 89 8th Interest & Maturity Dates: T8 = 7/31/2014 T8 − T7 = 92 Let
ai = 100 × max(r + s,0)/360
and
Ai = ai × (Ti − Ti−1) + 100 × 1{i = 8}
ai represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates Ti−1 and Ti, where i = 1,2, . . . ,8. ai's are computed using the spread s = 0.120% obtained at the auction, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:
a1 = 100 × max(0.00095022819 + 0.00120,0)/360 = 0.000597286
Ai represents the projected cash flow the floating rate note holder will receive, for a $100 par value, at the future interest payment date Ti, where i = 1,2, . . . ,8. Ti − Ti−1 is the number of days between the future interest payment dates Ti−1 and Ti. To account for the payback of the par value, the variable 1{i = 8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:
Ai = 92 × 0.000597286 = 0.054950312
and
A8 = 92 × 0.000597286 + 100 = 100.054950312
Let
Bi = 1 + (r + m) × (Ti − Ti − 1)/360
Bi represents the projected compound factor between the future dates Ti−1 and Ti, where i = 1,2, . . . ,8. All Bi's are computed using the discount margin m = 0.120% (equals the spread determined at the auction), and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:
B3 = 1 + (0.00095022819 + 0.00120) × 89/360 = 1.000531584.
The following table shows the projected daily accrued interest values for $100 par value (ai's), cash flows at interest payment dates (Ai's), and the compound factors between payment dates (Bi's).
Table 3 - Projected Cash Flows and Compound Factors
i ai Ai Bi 1 0.000597286 0.054950312 1.000549503 2 0.000597286 0.054950312 1.000549503 3 0.000597286 0.053158454 1.000531584 4 0.000597286 0.054950312 1.000549503 5 0.000597286 0.054950312 1.000549503 6 0.000597286 0.054950312 1.000549503 7 0.000597286 0.053158454 1.000531584 8 0.000597286 100.054950312 1.000549503 Computing the Price
The price is computed as follows:

B. For newly issued floating rate notes issued at a premium:
Formula:
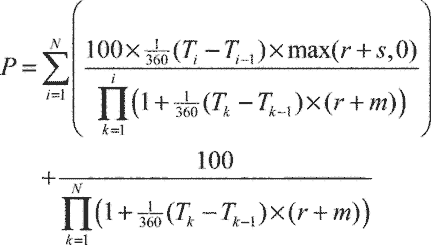
Example:
The purpose of this example is to demonstrate how a floating rate note auction can result in a price at a premium given a negative discount margin and spread at auction. For a new two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, solve for the price per 100 (P). In a new issue (not a reopening) of a floating rate note, the discount margin established at auction will be equal to the spread. In this example, the discount margin determined at auction is −0.150%, but the floating rate note is subject to a daily interest rate accrual minimum of 0.000%.
Definitions:
T0 = July 31, 2012.
N = 8.
TN = July 31, 2014.
r = 0.095022819%.
s = −0.150%.
m = −0.150%.
As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:
Table 1 - 13-Week Bill Auction Data
Auction date Issue date Maturity date Auction
clearing priceAuction high rate Index rate 7/23/2012 7/26/2012 10/25/2012 99.975986 0.095% 0.095022819% 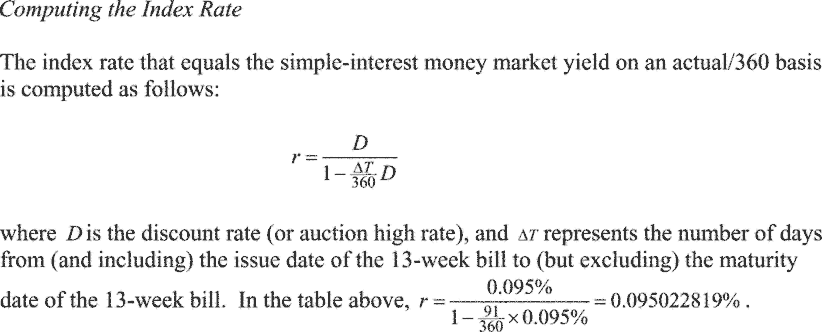
Computing the Projected Cash Flows
The following table presents the future interest payment dates and the number of days between them.
Table 2 - Payment Dates
Dates Days between dates Issue Date: T0 = 7/31/2012 1st Interest Date: T1 = 10/31/2012 T1 − T0 = 92 2nd Interest Date: T2 = 1/31/2013 T2 − T1 = 92 3rd Interest Date: T3 = 4/30/2013 T3 − T2 = 89 4th Interest Date: T4 = 7/31/2013 T4 − T3 = 92 5th Interest Date: T5 = 10/31/2013 T5 − T4 = 92 6th Interest Date: T6 = 1/31/2014 T6 − T5 = 92 7th Interest Date: T7 = 4/30/2014 T7 − T6 = 89 8th Interest & Maturity Dates: T8 = 7/31/2014 T8 − T7 = 92 Let
ai = 100 × max(r + s,0)/360
and
Ai = ai × (Ti− Ti− 1) + 100 × 1{i = 8}
ai Represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates Ti− 1 and Ti where i = 1,2, . . . ,8. ai's are computed using the spread s = − 0.150%, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:
ai = 100 × max(0.00095022819−0.00150,0)/360 = 100 × 0/360 = 0.000000000
Ai represents the projected cash flow the floating rate note holder will receive, for a $100 par value, at the future interest payment date Ti, where i = 1,2, . . ., 8. Ti − Ti−1 is the number of days between the future interest payment dates Ti−1 and Ti. To account for the payback of the par value, the variable 1{i=8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:
A1 = 92 × 0.000000000 = 0.000000000
and
A8 = 92 × 0.000000000 + 100 = 100.000000000
Let
Bi = 1 + (r + m) × (Ti-Ti−1)/360
Bi represents the projected compound factor between the future dates Ti−1 and Ti, where i = 1,2, . . ., 8. All Bi's are computed using the discount margin m = −0.150% (equals the spread obtained at the auction), and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012). For example:
B3 = 1 + (0.00095022819−0.00150) × 89/360 = 0.999864084.
The following table shows the projected daily accrued interests for $100 par value (ai's), cash flows at interest payment dates (Ai's), and the compound factors between payment dates (Bi's).
Table 3 - Projected Cash Flows and Compound Factors
i ai Ai Bi 1 0.000000000 0.000000000 0.999859503 2 0.000000000 0.000000000 0.999859503 3 0.000000000 0.000000000 0.999864084 4 0.000000000 0.000000000 0.999859503 5 0.000000000 0.000000000 0.999859503 6 0.000000000 0.000000000 0.999859503 7 0.000000000 0.000000000 0.999864084 8 0.000000000 100.000000000 0.999859503 Computing the Price
The price is computed as follows:

Definitions for Reopenings of Floating Rate Notes and Calculation of Interest Payments
IPi = the quarterly interest payment at date Ti.
PD = the price that includes the accrued interest per $100 par value as of the reopening issue date.
AI = accrued interest per $100 par value as of the reopening issue date.
PC = the price without accrued interest per $100 par value as of the reopening issue date.
T−1 = the dated date if the reopening occurs before the first interest payment date, or, otherwise, the latest interest payment date prior to the reopening issue date.
T0 = the reopening issue date.
N = the total number of remaining quarterly interest payments as of the reopening issue date.
i and k = indexes that identify the sequence of interest payment dates relative to the issue date. For example T1, T2, and T3 represent the first, second, and the third interest payment dates after the issue date respectively, while T−1 represents the preceding interest payment date before the issue date.
j = an index that identifies days between consecutive interest payment dates.
Ti = the ith remaining quarterly interest payment date.
Ti − Ti−1 = the number of days between the interest payment date Ti and the preceding interest payment date.
TN = the maturity date.
rj 's = the effective index rates for days between the last interest payment date and the reopening issue date.
r = the index rate applicable to the reopening issue date.
s = the spread.
m = the discount margin.
C. Pricing and accrued interest for reopened floating rate notes
Formula:

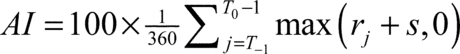
Example:
The purpose of this example is to determine the floating rate note prices with and without accrued interest at the time of the reopening auction. For a two-year floating rate note that was originally auctioned on July 25, 2012, with an issue date of July 31, 2012, reopened in an auction on August 30, 2012 and issued on August 31, 2012, with a maturity date of July 31, 2014, solve for accrued interest per 100 (AI), the price with accrued interest per 100 (PD) and the price without accrued interest per 100 (PC). Since this is a reopening of an original issue from the prior month, Table 2 as shown in the example is used for accrued interest calculations. In the case of floating rate note reopenings, the spread on the security remains equal to the spread that was established at the original auction of the floating rate notes.
Definitions:
T−1 = July 31, 2012.
T0 = August 31, 2012.
N = 8.
TN = July 31, 2014.
r = 0.105027876%.
s = 0.120%.
m = 0.100%.
The following table shows the past results for the 13-week bill auction.
Table 1 - 13-Week Bill Auction Data
Auction date Issue date Maturity date Auction
clearing
priceAuction
high rate
(percent)Index rate
(percent)7/23/2012 7/26/2012 10/25/2012 99.975986 0.095 0.095022819 7/30/2012 8/2/2012 11/1/2012 99.972194 0.110 0.110030595 8/6/2012 8/9/2012 11/8/2012 99.974722 0.100 0.100025284 8/13/2012 8/16/2012 11/15/2012 99.972194 0.110 0.110030595 8/20/2012 8/23/2012 11/23/2012 99.973167 0.105 0.105028183 8/27/2012 8/30/2012 11/29/2012 99.973458 0.105 0.105027876 
The following table shows the index rates applicable for the accrued interest.
Table 2 - Applicable Index Rate
Accrual starts Accrual ends Number of days in accrual period Applicable floating rate Auction date Index rate
(percent)7/31/2012 7/31/2012 1 7/23/2012 0.095022819 8/1/2012 8/6/2012 6 7/30/2012 0.110030595 8/7/2012 8/13/2012 7 8/6/2012 0.100025284 8/14/2012 8/20/2012 7 8/13/2012 0.110030595 8/21/2012 8/27/2012 7 8/20/2012 0.105028183 8/28/2012 8/30/2012 3 8/27/2012 0.105027876 Computing the Accrued Interest
The accrued interest as of the new issue date (8/31/2012) for a $100 par value is:
AI = 1 × 100 × max (0.00095022819 + 0.00120,0)/360
+ 6 × 100 × max (0.00110030595 + 0.00120,0)/360
+ 7 × 100 × max (0.00100025284 + 0.00120,0)/360
+ 7 × 100 × max (0.00110030595 + 0.00120,0)/360
+ 7 × 100 × max (0.00105028183 + 0.00120,0)/360
+ 3 × 100 × max (0.00105027876 + 0.00120,0)/360
AI = 1 × 0.000597286
+ 6 × 0.000638974
+ 7 × 0.000611181
+ 7 × 0.000638974
+ 7 × 0.000625078
+ 3 × 0.000625077
AI = 0.000597286 + 0.003833844 + 0.004278267 + 0.004472818 + 0.004375546 + 0.001875231
AI = 0.019432992 = $0.019433
Computing the Projected Cash Flows
The following table presents the future interest payment dates and the number of days between them.
Table 3 - Payment Dates
Dates Days between dates Original Issue Date: T−1 = 7/31/2012 New Issue Date: T0 = 8/31/2012 T0 − T−1 = 31 1st Interest Date: T1 = 10/31/2012 T1 − T0 = 61 2nd Interest Date: T2 = 1/31/2013 T2 − T1 = 92 3rd Interest Date: T3 = 4/30/2013 T3 − T2 = 89 4th Interest Date: T4 = 7/31/2013 T4 − T3 = 92 5th Interest Date: T5 = 10/31/2013 T5 − T4 = 92 6th Interest Date: T6 = 1/31/2014 T6 − T5 = 92 7th Interest Date: T7 = 4/30/2014 T7 − T6 = 89 8th Interest & Maturity Dates: T8 = 7/31/2014 T8 − T7 = 92 Let
ai = 100 × max(r + s, 0)/360
and
Ai = ai × (Ti − Ti−1) + 100 × 1{i = 8}
ai represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates Ti−1 and Ti, where i = 1,2,...,8. ai's are computed using the spread s = 0.120% obtained at the original auction, and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012). For example:
ai = 100 × max(0.00105027876 + 0.00120,0)/360 = 0.000625077
Ai represents the projected cash flow the floating rate note holder will receive, less accrued interest, for a $100 par value, at the future interest payment date Ti, where i = 1,2,...,8. Ti − Ti-1 is the number of days between the future interest payment dates Ti−1 and Ti. To account for the payback of the par value, the variable 1{i = 8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:
A1 = 61 × 0.000625077 = 0.038129697
and
A8 = 92 × 0.000625077 + 100 = 100.057507084
Let
Bi = 1 + (r + m) × (Ti − Ti-1)/360
Bi represents the projected compound factor between the future dates Ti−1 and Ti, where i = 1,2,...,8. All Bi's are computed using the discount margin m = 0.100% obtained at the reopening auction, and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012). For example:
B3 = 1 + (0.00105027876 + 0.00100) × 89/360 = 1.000506874
The following table shows the projected daily accrued interests for $100 par value (ai's), cash flows at interest payment dates (Ai's), and the compound factors between payment dates (Bi's).
Table 4 - Projected Cash Flows and Compound Factors
i ai Ai Bi 1 0.000625077 0.038129697 1.000347408 2 0.000625077 0.057507084 1.000523960 3 0.000625077 0.055631853 1.000506874 4 0.000625077 0.057507084 1.000523960 5 0.000625077 0.057507084 1.000523960 6 0.000625077 0.057507084 1.000523960 7 0.000625077 0.055631853 1.000506874 8 0.000625077 100.057507084 1.000523960 Computing the Price
The price with accrued interest is computed as follows:

D. For calculating interest payments:
Example:
For a new issue of a two-year floating rate note auctioned on July 25, 2012, and issued on July 31, 2012, with a maturity date of July 31, 2014, and a first interest payment date of October 31, 2012, calculate the quarterly interest payments (IPi) per 100. In a new issuance (not a reopening) of a new floating rate note, the discount margin determined at auction will be equal to the spread. The interest accrual rate used for this floating rate note on the issue date is 0.215022819% (index rate of 0.095022819% plus a spread of 0.120%) and this rate is used for each daily interest accrual over the life of the security for the purposes of this example.
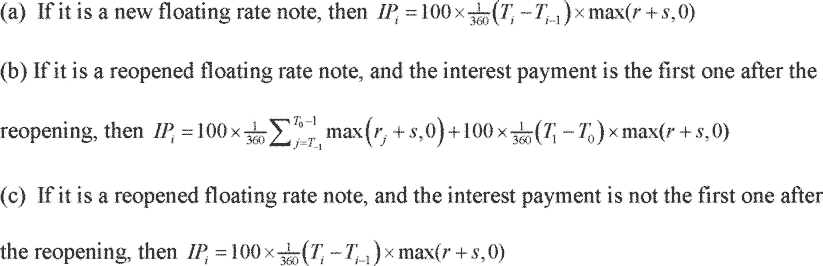
Example 1:
Projected interest payment as of the original issue date.
T0 = July 31, 2012.
N = 8.
TN = July 31, 2014.
r = 0.095022819%.
s = 0.120%.
m = 0.120%.
As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:
Table 1 - 13-Week Bill Auction Data
Auction date Issue date Maturity date Auction
clearing priceAuction high rate Index rate 7/23/2012 7/26/2012 10/25/2012 99.975986 0.095% 0.095022819% 
Computing the Projected Cash Flows
The following table presents the future interest payment dates and the number of days between them.
Table 2 - Payment Dates
Dates Days between dates Issue Date: T0 = 7/31/2012 1st Interest Date: T1 = 10/31/2012 T1 − T0 = 92 2nd Interest Date: T2 = 1/31/2013 T2 − T1 = 92 3rd Interest Date: T3 = 4/30/2013 T3 − T2 = 89 4th Interest Date: T4 = 7/31/2013 T4 − T3 = 92 5th Interest Date: T5 = 10/31/2013 T5 − T4 = 92 6th Interest Date: T6 = 1/31/2014 T6 − T5 = 92 7th Interest Date: T7 = 4/30/2014 T7 − T6 = 89 8th Interest & Maturity Dates: T8 = 7/31/2014 T8 − T7 = 92 Using the spread s = 0.120%, and the fixed index rate of r = 0.095022819% applicable to the issue date (7/31/2012), the first and seventh projected interest payments are computed as follows:
IP1 = 92 × [100 × max(0.00095022819 + 0.00120,0)/360]
IP1 = 92 × 0.000597286 = 0.054950312
IP7 = 89 × [100 × max(0.00095022819 + 0.00120,0)/360]
IP7 = 89 × 0.000597286 = 0.053158454
The following table shows all projected interest payments as of the issue date.
Table 3 - Projected Interest Payments
i Dates IPi 1 10/31/2012 0.054950312 2 1/31/2013 0.054950312 3 4/30/2013 0.053158454 4 7/31/2013 0.054950312 5 10/31/2013 0.054950312 6 1/31/2014 0.054950312 7 4/30/2014 0.053158454 8 7/31/2014 0.054950312 Example 2:
Projected interest payment as of the reopening issue date (intermediate values, including rates in percentage terms, are rounded to nine decimal places).
This example demonstrates the calculations required to determine the interest payment due when the reopened floating rate note is issued. This example also demonstrates the need to calculate accrued interest at the time of a floating rate reopening auction. Since this is a reopening of an original issue from 31 days prior, Table 5 as shown in the example is used for accrued interest calculations. For a two-year floating rate note originally auctioned on July 25, 2012 with an original issue date of July 31, 2012, reopened by an auction on August 30, 2012 and issued on August 31, 2012, with a maturity date of July 31, 2014, calculate the quarterly interest payments (IPI) per 100. T−1 is the dated date if the reopening occurs before the first interest payment date, or otherwise the latest interest payment date prior to the new issue date.
T−1 = July 31, 2012.
T0 = August 31, 2012.
N = 8.
TN = July 31, 2014.
r = 0.105027876%.
s = 0.120%.
m = 0.100%.
The following table shows the past results for the 13-week bill auction.
Table 4 - 13-Week Bill Auction Data
Auction date Issue date Maturity date Auction
clearing priceAuction
high rate
(percent)Index rate
(percent)7/23/2012 7/26/2012 10/25/2012 99.975986 0.095 0.095022819 7/30/2012 8/2/2012 11/1/2012 99.972194 0.110 0.110030595 8/6/2012 8/9/2012 11/8/2012 99.974722 0.100 0.100025284 8/13/2012 8/16/2012 11/15/2012 99.972194 0.110 0.110030595 8/20/2012 8/23/2012 11/23/2012 99.973167 0.105 0.105028183 8/27/2012 8/30/2012 11/29/2012 99.973458 0.105 0.105027876 
The following table shows the index rates applicable for the accrued interest.
Table 5 - Applicable Index Rate
Accrual starts Accrual ends Number of days in
accrual periodApplicable floating rate Auction date Index rate
(percent)7/31/2012 7/31/2012 1 7/23/2012 0.095022819 8/1/2012 8/6/2012 6 7/30/2012 0.110030595 8/7/2012 8/13/2012 7 8/6/2012 0.100025284 8/14/2012 8/20/2012 7 8/13/2012 0.110030595 8/21/2012 8/27/2012 7 8/20/2012 0.105028183 8/28/2012 8/30/2012 3 8/27/2012 0.105027876 Computing the accrued interest
The accrued interest as of 8/31/2012 for a $100 par value is:
AI = 1 × 100 × max (0.00095022819 + 0.00120,0)/360
+ 6 × 100 × max (0.00110030595 + 0.00120,0)/360
+ 7 × 100 × max (0.00100025284 + 0.00120,0)/360
+ 7 × 100 × max (0.00110030595 + 0.00120,0)/360
+ 7 × 100 × max (0.00105028183 + 0.00120,0)/360
+ 3 × 100 × max (0.00105027876 + 0.00120,0)/360
AI = 1 × 0.000597286
+ 6 × 0.000638974
+ 7 × 0.000611181
+ 7 × 0.000638974
+ 7 × 0.000625078
+ 3 × 0.000625077
AI = 0.000597286 + 0.003833844 + 0.004278267 + 0.004472818 + 0.004375546 + 0.001875231
AI = 0.019432992 = $0.019433
The following table presents the future interest payment dates and the number of days between them.
Table 6 - Payment Dates
Dates Days between dates Original Issue Date: T−1 = 7/31/2012 New Issue Date: T0 = 8/31/2012 T0 − T−1 = 31 1st Interest Date: T1 = 10/31/2012 T1 − T0 = 61 2nd Interest Date: T2 = 1/31/2013 T2 − T1 = 92 3rd Interest Date: T3 = 4/30/2013 T3 − T2 = 89 4th Interest Date: T4 = 7/31/2013 T4 − T3 = 92 5th Interest Date: T5 = 10/31/2013 T5 − T4 = 92 6th Interest Date: T6 = 1/31/2014 T6 − T5 = 92 7th Interest Date: T7 = 4/30/2014 T7 − T6 = 89 8th Interest & Maturity Dates: T8 = 7/31/2014 T8 − T7 = 92 Using the original spread s = 0.120% (obtained on 7/25/2012), and the fixed index rate of r = 0.105027876% applicable to the new issue date (8/31/2012), the first and eighth projected interest payments are computed as follows:
IP1 = 0.019432992 + 61 × [100 × max (0.00105027876 + 0.00120,0)/360]
IP1 = 0.019432992 + 61 × 0.000625077
IP1 = 0.019432992 + 0.038129697 = 0.057562689
and
IP8 = 92 × [100 × max (0.00105027876 + 0.00120,0)/360]
IP8 = 92 × 0.000625077 = 0.057507084
The following table shows all projected interest payments as of the new issue date.
Table 7 - Projected Interest Payments
i Dates IPi 1 10/31/2012 0.057562689 2 1/31/2013 0.057507084 3 4/30/2013 0.055631853 4 7/31/2013 0.057507084 5 10/31/2013 0.057507084 6 1/31/2014 0.057507084 7 4/30/2014 0.055631853 8 7/31/2014 0.057507084 Definitions for Newly Issued Floating Rate Notes with an Issue Date that Occurs after the Dated Date
PD = the price that includes accrued interest from the dated date to the issue date per $100 par value as of the issue date.
AI = the accrued interest per $100 par value as of the issue date.
PC = the price without accrued interest per $100 par value as of the issue date.
T−1 = the dated date.
T0 = the issue date.
N = the total number of remaining quarterly interest payments as of the new issue date.
i and k = indexes that identify the sequence of interest payment dates.
j = an index that identifies days between the dated date and the issue date.
Ti = the ith quarterly future interest payment date.
Ti − Ti−1 = the number of days between the interest payment date Ti and the preceding interest payment date.
TN = the maturity date.
rj 's = the effective index rates for days between the dated date and the issue date.
r = the index rate applicable to the issue date.
s = the spread.
m = the discount margin.
E. Pricing and accrued interest for new issue floating rate notes with an issue date that occurs after the dated date
Formula:
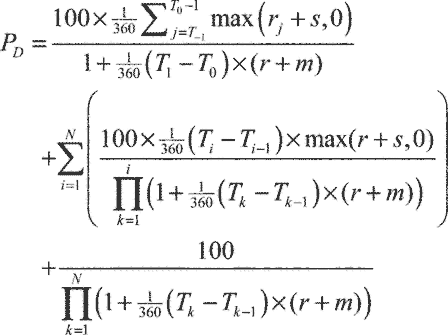
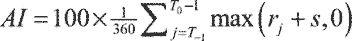
Example:
The purpose of this example is to demonstrate how a floating rate note can have a price without accrued interest of less than $100 par value when the issue date occurs after the dated date. An original issue two-year floating rate note is auctioned on December 29, 2011, with a dated date of December 31, 2011, an issue date of January 3, 2012, and a maturity date of December 31, 2013.
Definitions:
Dated date = 12/31/2011.
Issue date = 1/3/2012.
Maturity date = 12/31/2013.
Spread = 1.000% at auction.
Discount margin = 1.000%.
As of the issue date the latest 13-week bill, auctioned at least two days prior, has the following information:
Table 1 - 13-WEEK BILL AUCTION DATA
Auction date Issue date Maturity date Auction
clearing priceAuction high rate Index rate 12/27/2011 12/29/2011 3/29/2012 99.993681 0.025% 0.025001580% 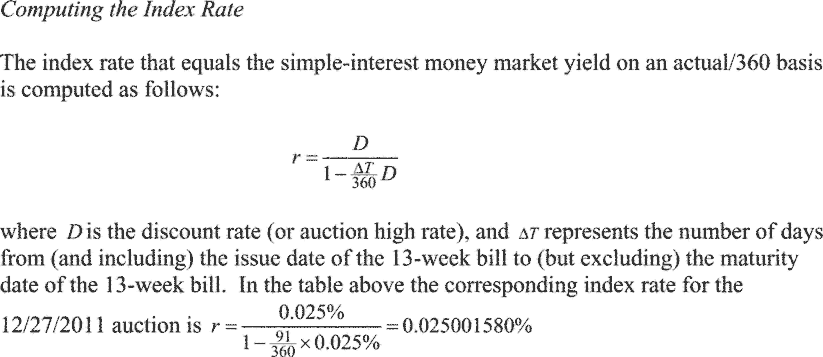
The following table shows the index rates applicable for the accrued interest.
Table 2 - Applicable Index Rate
Accrual starts Accrual ends Number of days in
accrual periodApplicable floating rate Auction date Index rate 12/31/2011 1/2/2012 3 12/27/2011 0.025001580% Computing the accrued interest
The accrued interest as of the new issue date (1/3/2012) for a $100 par value is:
AI = 3 × 100 × max (0.00025001580 + 0.01000,0)/360
AI = 3 × 0.002847227
AI = 0.008541681 = $0.008542
Computing the Projected Cash Flows
The following table presents the future interest payment dates and the number of days between them.
Table 3 - Payment Dates
Dates Days between dates Dated Date: = T−1 = 12/31/2011 Issue Date: T0 = 1/3/2012 T0 − T−1 = 3 1st Interest Date: T1 = 3/31/2012 T1 − T0 = 88 2nd Interest Date: T2 = 6/30/2012 T2 − T1 = 91 3rd Interest Date: T3 = 9/30/2012 T3 − T2 = 92 4th Interest Date: T4 = 12/31/2012 T4 − T3 = 92 5th Interest Date: T5 = 3/31/2013 T5 − T4 = 90 6th Interest Date: T6 = 6/30/2013 T6 − T5 = 91 7th Interest Date: T7 = 9/30/2013 T7 − T6 = 92 8th Interest & Maturity Dates: T8 = 12/31/2013 T8 − T7 = 92 Let
ai = 100 × max(r + s, 0)/360
and
Ai = ai × (Ti − Ti−1) + 100 × 1{i = 8}
ai represents the daily projected interest, for a $100 par value, that will accrue between the future interest payment dates Ti−1 and Ti, where i = 1,2,...,8. ai's are computed using the spread s = 1.000% obtained at the auction, and the fixed index rate of r = 0.025001580% applicable to the issue date (1/3/2012). For example:
a1 = 100 × max(0.00025001580 + 0.01000,0)/360 = 0.002847227
Ai represents the projected cash flow the floating rate note holder will receive, less accrued interest, for a $100 par value, at the future interest payment date Ti, where i = 1,2,...,8. Ti − Ti−1 is the number of days between the future interest payment dates Ti-1 and Ti. To account for the payback of the par value, the variable 1{i = 8} takes the value 1 if the payment date is the maturity date, or 0 otherwise. For example:
A1 = 88 × 0.002847227 = 0.250555976
and
A8 = 92 × 0.002847227 + 100 = 100.261944884
Let
Bi = 1 + (r + m) × (Ti − Ti−1)/360
Bi represents the projected compound factor between the future dates Ti−1 and Ti, where i = 1,2,...,8. All Bi's are computed using the discount margin m = 1.000% (equals the spread obtained at the auction), and the fixed index rate of r = 0.025001580% applicable to the issue date (1/3/2012). For example:
B3 = 1 + (0.00025001580 + 0.01000) × 92/360 = 1.002619448
The following table shows the projected daily accrued interests for $100 par value (ai 's), cash flows at interest payment dates (Ai 's), and the compound factors between payment dates (Bi's).
Table 4 - Projected Cash Flows and Compound Factors
i ai Ai Bi 1 0.002847227 0.250555976 1.002505559 2 0.002847227 0.259097657 1.002590976 3 0.002847227 0.261944884 1.002619448 4 0.002847227 0.261944884 1.002619448 5 0.002847227 0.256250430 1.002562504 6 0.002847227 0.259097657 1.002590976 7 0.002847227 0.261944884 1.002619448 8 0.002847227 100.261944884 1.002619448 Computing the price
The price with accrued interest is computed as follows:
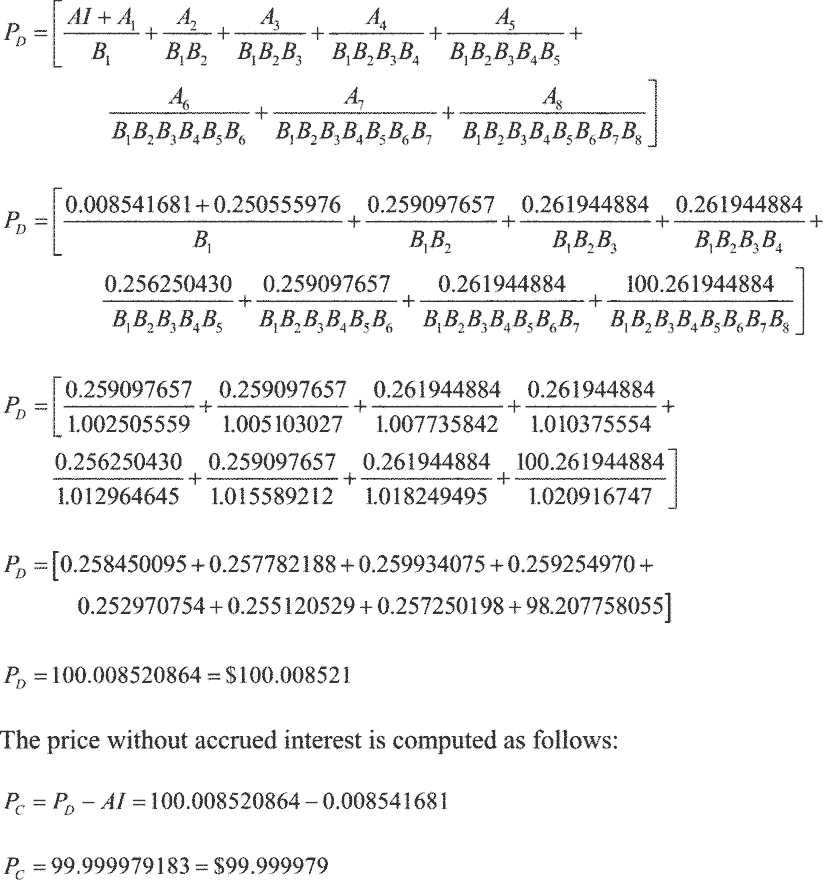
V. Computation of Adjusted Values and Payment Amounts for Stripped Inflation-Protected Interest Components
Note:Valuing an interest component stripped from an inflation-protected security at its adjusted value enables this interest component to be interchangeable (fungible) with other interest components that have the same maturity date, regardless of the underlying inflation-protected security from which the interest components were stripped. The adjusted value provides for fungibility of these various interest components when buying, selling, or transferring them or when reconstituting an inflation-protected security.
Definitions:
c = C/100 = the regular annual interest rate, payable semiannually, e.g., .03625 (the decimal equivalent of a 35⁄8% interest rate)
Par = par amount of the security to be stripped
Ref CPIIssueDate = reference CPI for the original issue date (or dated date, when the dated date is different from the original issue date) of the underlying (unstripped) security
Ref CPIDate = reference CPI for the maturity date of the interest component
AV = adjusted value of the interest component
PA = payment amount at maturity by Treasury
Formulas:
AV = Par(C/2)(100/Ref CPIIssueDate) (rounded to 2 decimals with no intermediate rounding)
PA = AV(Ref CPIDate/100) (rounded to 2 decimals with no intermediate rounding)
Example:
A 10-year inflation-protected note paying 37⁄8% interest was issued on January 15, 1999, with the second interest payment on January 15, 2000. The Ref CPI of January 15, 1999 (Ref CPIIssueDate) was 164.00000, and the Ref CPI on January 15, 2000 (Ref CPIDate) was 168.24516. Calculate the adjusted value and the payment amount at maturity of the interest component.
Definitions:
c = .03875
Par = $1,000,000
Ref CPIIssueDate = 164.00000
Ref CPIDate = 168.24516
Resolution:
For a par amount of $1 million, the adjusted value of each stripped interest component was $1,000,000(.03875/2)(100/164.00000), or $11,814.02 (no intermediate rounding).
For an interest component that matured on January 15, 2000, the payment amount was $11,814.02 (168.24516/100), or $19,876.52 (no intermediate rounding).
VI. Computation of Purchase Price, Discount Rate, and Investment Rate (Coupon-Equivalent Yield) for Treasury Bills
A. Conversion of the discount rate to a purchase price for Treasury bills of all maturities:
Formula:
P = 100 (1 − dr / 360).
Where:
d = discount rate, in decimals.
r = number of days remaining to maturity.
P = price per 100 (dollars).
Example:
For a bill issued November 24, 1989, due February 22, 1990, at a discount rate of 7.610%, solve for price per 100 (P).
Definitions:
d = .07610.
r = 90 (November 24, 1989 to February 22, 1990).
Resolution:
P = 100 (1 − dr / 360).
(1) P = 100 [1 − (.07610)(90) / 360].
(2) P = 100 (1 − .019025).
(3) P = 100 (.980975).
(4) P = 98.097500.
Note:Purchase prices per $100 are rounded to six decimal places, using normal rounding procedures.
B. Computation of purchase prices and discount amounts based on price per $100, for Treasury bills of all maturities:
1. To determine the purchase price of any bill, divide the par amount by 100 and multiply the resulting quotient by the price per $100.
Example:
To compute the purchase price of a $10,000 13-week bill sold at a price of $98.098000 per $100, divide the par amount ($10,000) by 100 to obtain the multiple (100). That multiple times 98.098000 results in a purchase price of $9,809.80.
2. To determine the discount amount for any bill, subtract the purchase price from the par amount of the bill.
Example:
For a $10,000 bill with a purchase price of $9,809.80, the discount amount would be $190.20, or $10,000 − $9,809.80.
C. Conversion of prices to discount rates for Treasury bills of all maturities:
Formula:

Where:
P = price per 100 (dollars).
d = discount rate.
r = number of days remaining to maturity.
Example:
For a 26-week bill issued December 30, 1982, due June 30, 1983, with a price of $95.934567, solve for the discount rate (d).
Definitions:
P = 95.934567.
r = 182 (December 30, 1982, to June 30, 1983).
Resolution:

(2) d = [.04065433 × 1.978021978].
(3) d = .080415158.
(4) d = 8.042%.
Note:Prior to April 18, 1983, we sold all bills in price-basis auctions, in which discount rates calculated from prices were rounded to three places, using normal rounding procedures. Since that time, we have sold bills only on a discount rate basis.
D. Calculation of investment rate (coupon-equivalent yield) for Treasury bills:
1. For bills of not more than one half-year to maturity:
Formula:

Where:
i = investment rate, in decimals.
P = price per 100 (dollars).
r = number of days remaining to maturity.
y = number of days in year following the issue date; normally 365 but, if the year following the issue date includes February 29, then y is 366.
Example:
For a cash management bill issued June 1, 1990, due June 21, 1990, with a price of $99.559444 (computed from a discount rate of 7.930%), solve for the investment rate (i).
Definitions:
P = 99.559444.
r = 20 (June 1, 1990, to June 21, 1990).
y = 365.
Resolution:
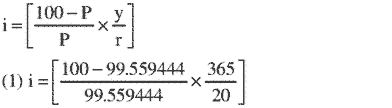
(2) i = [.004425 × 18.25].
(3) i = .080756.
(4) i = 8.076%.
2. For bills of more than one half-year to maturity:
Formula:
P [1 + (r − y/2)(i/y)] (1 + i/2) = 100.
This formula must be solved by using the quadratic equation, which is:
ax2 + bx + c = 0.
Therefore, rewriting the bill formula in the quadratic equation form gives:

and solving for “i” produces:

Where:
i = investment rate in decimals.
b = r/y.
a = (r/2y) − .25.
c = (P−100)/P.
P = price per 100 (dollars).
r = number of days remaining to maturity.
y = number of days in year following the issue date; normally 365, but if the year following the issue date includes February 29, then y is 366.
Example:
For a 52-week bill issued June 7, 1990, due June 6, 1991, with a price of $92.265000 (computed from a discount rate of 7.65%), solve for the investment rate (i).
Definitions:
r = 364 (June 7, 1990, to June 6, 1991).
y = 365.
P = 92.265000.
b = 364 / 365, or .997260274.
a = (364 / 730) − .25, or .248630137.
c = (92.265 − 100) / 92.265, or −.083834607.
Resolution:

(3) i = (−.997260274 + 1.038221216) / .497260274.
(4) i = .040960942 / .497260274.
(5) i = .082373244 or
(6) i = 8.237%.
[69 FR 45202, July 28, 2004, as amended at 69 FR 52967, Aug. 30, 2004; 69 FR 53622, Sept. 2, 2004; 73 FR 14939, Mar. 20, 2008; 78 FR 46428, 46430, July 31, 2013; 78 FR 50335, Aug. 19, 2013; 78 FR 52857, Aug. 27, 2013; 78 FR 59228-59230, Sept. 26, 2013; 81 FR 43070, July 1, 2016]
